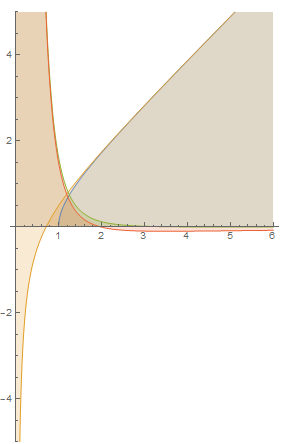
So, I am trying to find the regularized value of the divergent integral $I=\int_1^\infty \sqrt{x^2-1}dx$. Since the area of $\int_0^1 \sqrt{1-x^2}dx=\frac\pi4$, I wonder whether the area under hyperbola would be interesting as well.
So, I applied my method that uses Laplace transform $\mathcal{L}_t[t f(t)](x)$ so to convert divergent integrals into equivalent ones.
This way I obtained a family of equivalent integrals:
$\int_1^\infty \sqrt{x^2-1}dx=\int_0^\infty \frac{K_2(x)}{x}dx=\int_0^\infty \left(x-\frac{1}{2 x}\right) dx =\int_0^\infty \left(\frac{2}{x^3}-\frac{1}{2 x}\right)dx$
Now, we already know that $\int_0^\infty \frac1xdx$ regularizes to $\gamma$ while $\int_0^\infty \frac1{x^3}dx$ and $\int_0^\infty xdx$ regularize to zero, so integral $I$ regularizes to $-\frac\gamma2$.
This is a very interesting result. One also can notice that the area of the hyperbolic sector between the hyperbola and $y=x$ regularizes to $\gamma/2$, but we know that twice the area of the hyperbolic sector corresponds to hyperbolic angle. So, does $\gamma$ corresponds to infinite hyperbolic angle?

P.S. The area under the conjugate hyperbola is $\int_0^\infty \sqrt{x^2+1}dx=\int_0^\infty \left(x+\frac{1}{2 x}\right)dx$, which regularizes to $\gamma/2$.