I don't know a general answer for your question, but I would be very excited to learn more about this topic. Even having more examples would be very interesting. Here is what I do know.
In the forward direction - from the upper half plane $\mathbb{H}$ to the polygon $P$. If $P$ is a triangle, then the SC map is basically given by the Beta function. If $P$ is a regular $n$-gon, the SC map can given in terms of a hypergeometric function. See Exercise 5.19 of the paper "Mappings to polygonal domains" in the book "Explorations in complex analysis".
In the backwards direction - from $P$ to $\mathbb{H}$. If $P$ is a "rational" triangle (all angles are rational multiples of $\pi$) then it may be unfolded to give a quadratic differential $q_P$ on a Riemann surface $X_P$. (The unfolding procedure comes from the theory of billiards.) The inverse to the SC map is then, by the Schwarz reflection principle, a branched covering from $X_P$ to the Riemann sphere. In the presence of symmetries this covering map can have pretty expressions.
I carefully understood this covering map in two cases beyond the trigonometric example. When $P$ is a square or a hexagon we treat it as the unfolding of a rational triangle $Q$. We deduce that $X_Q$ is a square or hexagonal torus. Thus the covering map is the Weierstrass $\wp$-function for the square and hexagonal lattices, respectively. We can express these (as usual) in terms of theta functions
Remarks:
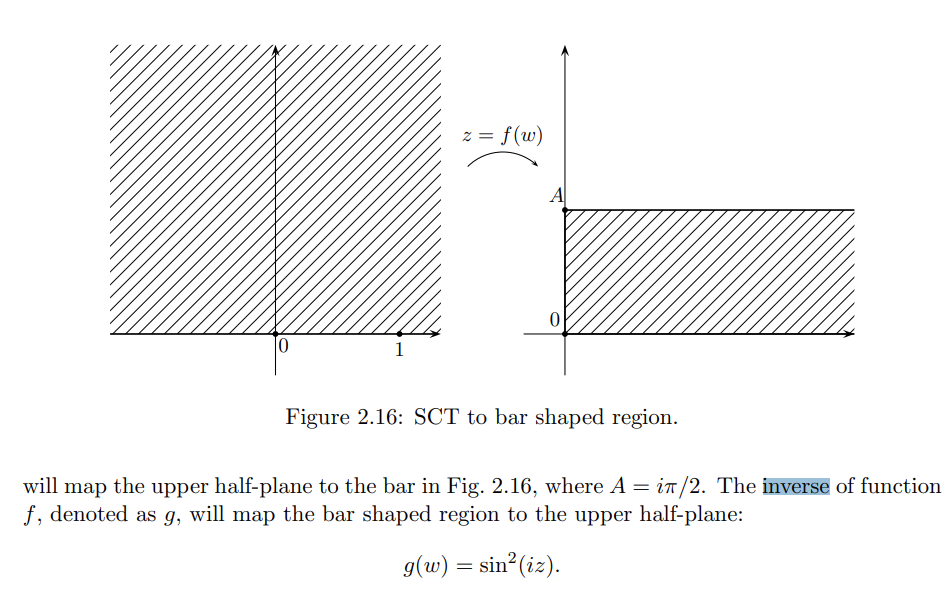
When $P$ is a rectangle then a similar discussion holds - this case is discussed in the BSc thesis you link to.
When $P$ is rational, the covering map lifts to give an automorphic map from $\tilde{X}_P$ (the universal cover) to $\mathbb{H}$. This will have a Poincaré series. But Poincaré series have very poor convergence properties, so I don't really regard this as an "answer".
When $P$ is not rational, I don't see how to proceed... It would be nice to have "non-existence results", but I don't know what those would look like.