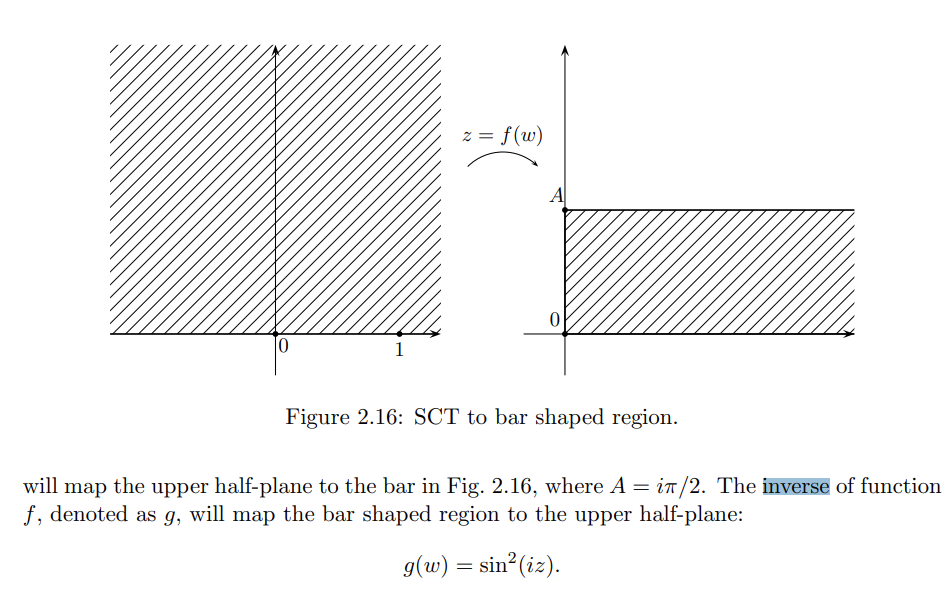
As an elementary result in Complex Analysiscomplex analysis, one can use the Argument Principleargument principle to show that the Schwarz-Christoffel transform is injective on the interior of the polygon to which it maps. Could this one-to-one correspondence be used to show that such an inverse mapping exists for at least a subset of the upper half-plane? In my search for an answer, I found page 24 of the following paper that mentions the existence of a trigonometric inverse for a mapping to a bar-shaped region. Does a generalization of this result hold? More specifically, has existing literature produced an inverse formula for any polygonal geometry?
I should also hope to refer anyone curious about the existence and Holder continuity of an inverse to the following post.
Thank you all.