Why is the regulator of the degree 11 extension of $\mathbb{Q}$ so large?

Question

Let $K_\infty/\mathbb{Q}$ denote the $\hat{\mathbb{Z}}$-extension of $\mathbb{Q}$. Then for each $n\geq1$, $K_\infty$ has a unique subfield $K_n$ of degree $n$ over $\mathbb{Q}$.

The fields $K_n$ are rather nice:

• $K_n$ is Galois over $\mathbb{Q}$, and $\mathrm{Gal}(K_n/\mathbb{Q})$ is cyclic of order $n$ (so Kronecker-Weber applies),
• The subfields of $K_n$ are exactly $K_m$ for $m\mid n$,
• $K_n$ is totally real,
• It is conjectured that $K_{p^j}$ always has class number 1 (see this answer).

I was looking through these fields in the LMFDB (links at the end of this question), and I noticed something peculiar: $$\begin{array}{l|l} n&\lim_{s\to1^+}(s-1)\zeta_{K_n}(s)\\\hline 1&1\\ 2&0.623225240141\\ 3&0.3774610891760\\ 4&0.431652451862\\ 5&0.411382062671\\ 6&0.26281913742\\ 7&0.29967691809\\ 8&0.3399574212\\ 9&0.231073814089\\ 10&0.59812750863\\ 11&\mathbf{\color{red}{10.9924154253}}\\ 12&0.272503409905\\ 13&0.480494415585\\ 14&0.303247583959919\\ 15&0.126218760817\\ 16&0.271689000529\\ 17&0.149703203263054\\ 18&0.121386452739\\ \end{array}\qquad\qquad\qquad\begin{array}{l|l} n&\lim_{s\to1^+}(s-1)\zeta_{K_n}(s)\\\hline 19&0.127769546503240\\ 20&0.148293571484876\\ 21&0.325798468160489\\ 22&0.481687105754706\\ 23&0.123796267561194\\ 24&0.139575702501298\\ 25&0.167117342071058\\ 26&0.522724794223398\\ 27&0.174105094869797\\ 28&0.448262864859088\\ 29&0.239734187517100\\ 30&0.106243397680971\\ 31&\\ 32&0.278099976864148\\ 33&\mathbf{\color{red}{10.8513191982336}}\\ 34&\\ 35&\\ 36&0.121939637724450 \end{array}$$ For these values of $n$, the limit always lies in the interval $[0.1,1]$, except for $n=11$ and $n=33$ (but not $n=22$), where the limit is over $10$ times larger!

Why is the residue of the pole of $\zeta_{K_n}(s)$ so large for $n=11$? (and also $n=33$, but not $n=22$)

Here are two potential ways of approaching the question:

1. If $p$ is an odd prime then $\Delta_{K_p}=p^{2(p-1)}$ and conjecturally $h_{K_p}=1$ so we have $$\lim_{s\to1^+}(s-1)\zeta_{K_p}(s)=(2/p)^{p-1}\mathrm{Reg}_{K_p}.$$ Thus, the question could be phrased as:

Why is the regulator of $K_{11}$ so large?

2. We have $\lim_{s\to1^+}(s-1)\zeta_{K_n}(s)=\prod_{\chi\neq1}L(1,\chi)$. Here are pictures of $L(1,\chi)$ for $n=7,11,13$. Of course, these pictures don't really explain anything by themselves.

$p=7$ (mostly in the unit circle so the product is small, $\approx0.3$)

$p=11$ (almost entirely outside of the unit circle so the product is large, $\approx11$)

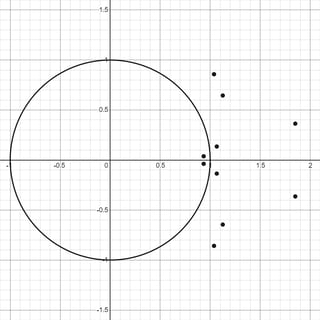
$p=13$ (mostly in the unit circle so the product is small, $\approx0.5$)

---

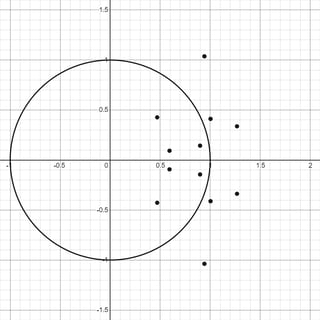
Here are the LMFDB links:

$K_1$ $K_2$ $K_3$ $K_4$ $K_5$ $K_6$ $K_7$ $K_8$ $K_9$ $K_{10}$ $K_{11}$ $K_{12}$ $K_{13}$ $K_{14}$ $K_{15}$ $K_{16}$ $K_{17}$ $K_{18}$ $K_{19}$ $K_{20}$ $K_{21}$ $K_{22}$ $K_{23}$ $K_{24}$ $K_{25}$ $K_{26}$ $K_{27}$ $K_{28}$ $K_{29}$ $K_{30}$ $K_{31}$ $K_{32}$ $K_{33}$ $K_{34}$ $K_{35}$ $K_{36}$

Answer 1

A prime $\ell$ splits in $K_p$ for $p$ odd if and only if $$\ell^{p-1} \equiv 1 \mod p^2.$$

We can express $\lim_{s \to 1} (s-1) \zeta_{K_n(s)}$ as the product of $\frac{1}{ 1- |\mathfrak a|^{-1} }$ over primes $\mathfrak a$ of $K_n$, regualarized by dividing by the product of $\frac{1}{1-\ell}$ over primes $\ell$ of $\mathbb Q$.

From this perspective, large values of $\lim_{s \to 1} (s-1) \zeta_{K_p(s)}$ are "explained" by the existence of small primes that split in $K_p$, which give a large contribution to this product.

For fixed $\ell$, the $p$ for which $\ell$ splits in $K_p$ are the generalized Wieferich primes. Examining that table, we see that for $\ell=3$, $11$ is the smallest Wieferich prime. It is exceptionally small. In particular, if we ignore $\ell> p$ as that is the range where we start expecting to have a split prime for random reasons, then the next-smallest Wiefierch prime for any $\ell<11$ is $p=1093$ for $\ell=2$.

The contribution of the primes lying over $3$ to the zeta function is $(3/2)^{11}\approx 86.5$. If we divide by the Euler factors for primes $<11$ of the zeta function of $\mathbb Q$, or $ 2 \cdot (3/2) \cdot (5/4) \cdot (7/6) $, we get $19.8$, which is on the same order of magnitude as your limit. Thus, this exceptional split prime gives an explanation for the unusually large value.

Examining the table linked above, we might suspect that exceptionally large values might occur for $K_p$ when $p=43, 71, 137 863, 1093, 3511, \dots $ owing to the split primes $\ell=19, 11,19, 13, 2,2,\dots$ respectively.

The distinct behavior of $K_{22}$ and $K_{33}$ is probably related to what happens to these small primes in those two extensions. In $K_{22}$ they are inert, taking the local factor of the zeta function lying over $3$ from $(3/2)^{11}$ to $(9/8)^{11}$, a loss of a factor of $\approx 23.7$ (matching the ratio of the residues in your table), while in $K_{33}$ they are ramified, preserving their norms, and preserving the local factor $(3/2)^{11}$ (explaining why the residue barely changes).