$\newcommand\R{\mathbb R}\newcommand\ep\epsilon\newcommand\bad{\text{bad}}$One would think that the answer is "of course no, descent does not have to monotonic". However, the no stationary points condition is a strong one. So, the answer actually is "no, but the effect seems to be small and require fine tuning to be seen".
Indeed, let $n=2$, $f:=P$, and $X:=\mathcal X=\{0\}$, so that $X^c=\R^2\setminus\{0\}$. Let us seek the counterexample of the form
\begin{equation*}
f(r,t)=g(r)\Big(2+\sin\frac{h(r,t)}r\Big),\tag{1}
\end{equation*}
where $r\in(0,\infty)$ and $t\in\R$ are the polar coordinates of a point in $X^c$; $g$ is differentiable, with $g(0+)=0$, $g'>0$, and $g'(0+)=0$; and $h(r,t)$ is differentiable in $(r,t)\in X^c$ and periodic in $t$ with period $2\pi$; we also assume that $h(r,t)\to1$ uniformly in $t$ as $r\downarrow0$, so that $\sin\frac{h(r,t)}r$ in (1) is highly oscillating as $r\downarrow0$. Here we identify $X^c$ with the set $(0,\infty)\times(-\pi,\pi]$ of the pairs $(r,t)$ of polar coordinates.
The partial derivatives of $f(r,t)$ in $r$ and $t$ are
\begin{equation*}
f'_r(r,t)=g'(r) \Big(2+\sin\frac{h(r,t)}r\Big) \\
+\frac{g(r)}{r^2}\,(r h'_r(r,t)-h(r,t))
\cos\frac{h(r,t)}r\tag{2}
\end{equation*}
and
\begin{equation*}
f'_t(r,t)=\frac{g(r)}r\,h'_t(r,t)\cos\frac{h(r,t)}r.\tag{3}
\end{equation*}
We need to ensure the no stationary points condition, that is, the condition that, for any $(r,t)\in X^c$, either $f'_r(r,t)\ne0$ or $f'_t(r,t)\ne0$. If $f'_t(r,t)=0$ for some $(r,t)\in X^c$, then either (i) $\cos\frac{h(r,t)}r=0$ or (ii) $h'_t(r,t)=0$. In case (i), $f'_r(r,t)=g'(r) \big(2+\sin\frac{h(r,t)}r\big)>0$.
Consider now case (ii), which will necessarily occur for each real $r>0$, at least for two values of $t\in(-\pi,\pi]$, where the maximum and minimum of $h(r,t)$ in $t$ occur. Note that
\begin{equation*}
k:=\max_{t\in(-\pi,\pi]}\Big|\frac{\cos u}{2+\sin u}\Big|=\frac1{\sqrt3},\tag{4}
\end{equation*}
with the maximum attained at $u\in\{-\pi/6,-5\pi/6\}$. So, by (2), for $f'_r(r,t)\ne0$ it is enough that
\begin{equation*}
\frac{r^2g'(r)}{g(r)}>\max_{t\in(-\pi,\pi]}|r h'_r(r,t)-h(r,t)| \tag{5}
\end{equation*}
(for real $r>0$).
At the points $t$ where the extrema of $|h(r,t)|$ in $t$ occur, we have $r h'_r(r,t)=0$ and hence $|r h'_r(r,t)-h(r,t)|=|h(r,t)|$. At all points $(r,t)\in X^c$ with such $t$, we want $h'_r(r,t)$ to have the same sign as $h(r,t)$, to make the restriction (5) as weak as possible.
Certain considerations suggest that it may make sense to define the differentiable $2\pi$-periodic in $t$ function $h$ by the formula
\begin{equation*}
h(r,t):=1-\frac{r^2}{1+r^2}\, t^2 (\pi -| t| )^2\tag{6}
\end{equation*}
for $t\in(-\pi,\pi]$. Then case (ii) $h'_t(r,t)=0$ (for $t\in(-\pi,\pi]$) means that $t\in\{0,\pi\}$, and
\begin{equation*}
f'_r(r,t)\big|_{t\in\{0,\pi\}}=g'(r) \Big(2+\sin\frac1r\Big)
-\frac{g(r)}{r^2}\,\cos\frac1r.\tag{7}
\end{equation*}
If $g$ solves the ODE $g'(r)=k\frac{g(r)}{r^2}$ (with $k$ as in (4)) -- e.g. when $g(r)=e^{-k/r}$, then, by (7), we will have $f'_r(r,t)\ge0$. Now, to ensure that $f'_r(r,t)>0$ (still in case (ii) $h'_t(r,t)=0$), it is enough to let
\begin{equation*}
g(r):=(1+cr)e^{-k/r}
\end{equation*}
for any fixed real $c>0$ and all real $r>0$. Indeed, then
\begin{equation*}
r^2e^{k/r}\,f'_r(r,t)\big|_{t\in\{0,\pi\}} \\
=
\Big(2+\sin\frac1r\Big) (k(1+cr)r+c r^2)-(1+c r) \cos\frac1r \\
\ge\Big(2+\sin\frac1r\Big)c r^2>0.\tag{8}
\end{equation*}
Thus, we have a nonnegative differentiable function on $\R^2$ vanishing only on $X=\{0\}$, with no stationary points in $X^c$.
On the other hand,
\begin{equation*}
\bad(r):=f'_r(r,1)\,\frac{kr^2 e^{k/r}}{k + c k r + c r^2} \\
=
k(2+\sin u_r) -q(r) \cos u_r,\tag{9}
\end{equation*}
where
\begin{equation*}
u_r:=\frac1r-\frac{(\pi -1)^2 r}{1+r^2}\sim\frac1r\tag{10}
\end{equation*}
and
\begin{equation*}
q(r):=\frac{k (1+(3-2 \pi +\pi ^2) r^2-(\pi -2) \pi r^4) (c r+1)}{(1+r^2)^2(c k r+c r^2+k)} \\
=1+((\pi -1)^2-c\sqrt3) r^2+O(r^3)
\end{equation*}
as ($r\downarrow0$), so that
\begin{equation*}
q(r)>1+4r^2>1\tag{11}
\end{equation*}
eventually (that is, for all small enough $r>0$) if $c=1/10$ (say).
Moreover, in view of (10), the equation $u_r=-\pi/6+2\pi m$ has solutions $r_m\sim1/(2\pi m)$ for all large enough natural $m$. So (cf. (4)), for such $m$, by (11),
\begin{equation*}
\bad(r_m)=
\frac{\sqrt3}2(1-q(r_m))<-\frac{\sqrt3}2\,4r_m^2<0,
\end{equation*}
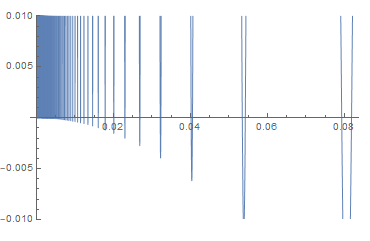
whence, by (9), the radial derivative $f'_r(r_m,1)$ is strictly negative, whereas $r_m\downarrow0$. $\Box$
Here is the graph $\{(r,\bad(r))\colon0<r<1/10,-1/100<\bad(r)<1/100\}$: