1
$\begingroup$

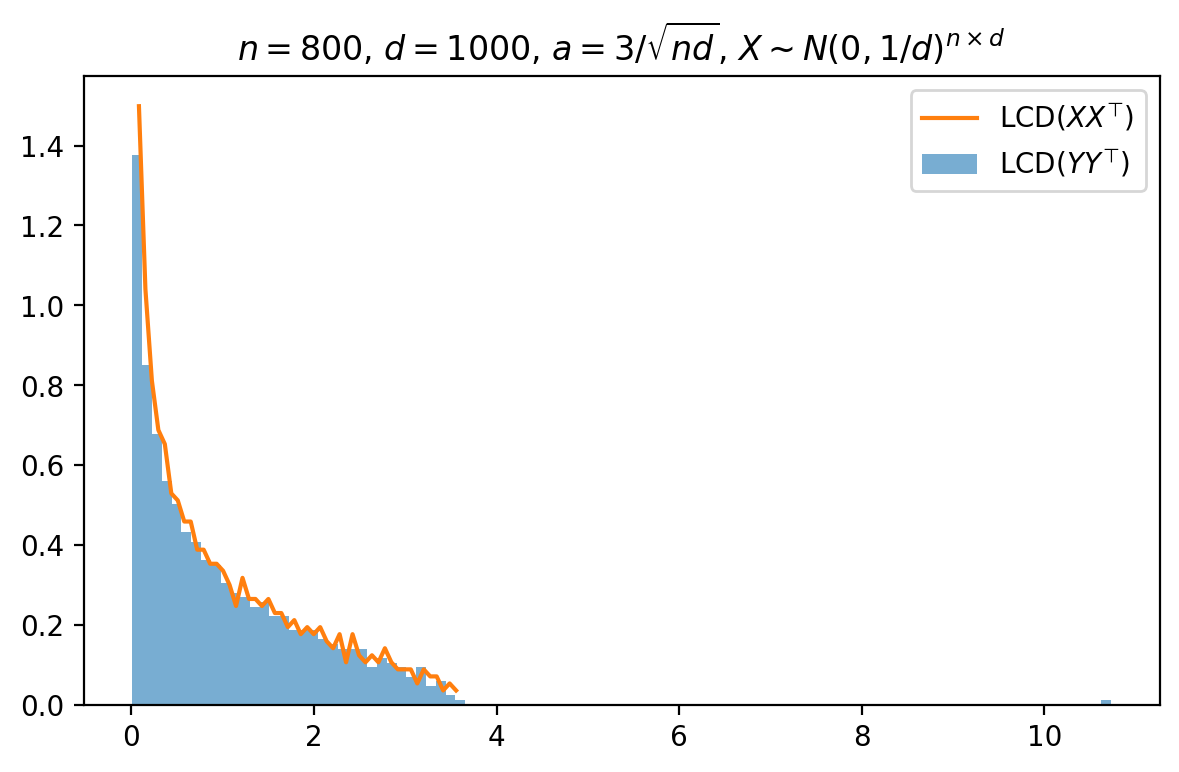
Let $a \in \mathbb R$ be a determinstic scalar and let $X$ be and $n \times d$ such that the $n \times n$ psd random matrix $S=XX^T$ has limiting eigenvalue distribution $\mu$, when $n,d \to \infty$ with $n/d = \gamma \in (0,\infty)$. Consider the psd random matrix $T=YY^\top$, where $Y$ is the $n \times d$ random matrix with entries $Y_{ij} = X_{ij} + a$.

Question. What is the limiting eigenvalue distribution $\lambda$ of $T$ ?

The example I have in mind is when $X$ has iid rows drawn from an isotropic log-concave distribution in $\mathbb R^d$. For gaussian of uniform on unit-sphere in $\mathbb R^d$.

Notes

  • My guess is that the Stieltjes transform of $\lambda$ would be some how given implicitly via the Stieltjes transform of $\mu$.
  • I'm only interesting in being able to integrate w.r.t $\lambda$. For example, I'm interested computing sums like $\sum_i \dfrac{\ell_i}{(\ell_i + \lambda)^2}$, where $\ell_1 \ge \ell_2 \ge \ldots $ are the eigenvalues of $T$.
Improve this question
$\endgroup$
6
  • $\begingroup$ It is a finite rank perturbation, since $YY^T =XX^T+ AX^T+X^TA+AA^T$ where $A_{ij}=a$; so you won't feel any change in the limit of the emprirical measure (due to interleaving) $\endgroup$
    – ofer zeitouni
    Commented Mar 9, 2021 at 11:50
  • $\begingroup$ @oferzeitouni Thanks for the comment. Do you mean $YY^\top$ and $XX^\top + aa^\top$ have the same LSD and that the latter can be directly related to the LSD of $XX^\top$ ? $\endgroup$
    – dohmatob
    Commented Mar 9, 2021 at 16:28
  • $\begingroup$ Your question is not clear, so my comment referred to the fact that if you are interested in the limit of the empirical measure, as the dimension grows, it is the same for $YY^T$ and $XX^T$, for the reason I mentioned (which is actually a proof). It is not the same LSD, only the limits are the same. This is a much weaker statement than your conjecture. $\endgroup$
    – ofer zeitouni
    Commented Mar 10, 2021 at 22:03
  • $\begingroup$ @oferzeitouni I'm even more confused. It's probably a problem with terminology on my part. By "only the limits are the same" do you mean "only the extreme eigenvalues" are the same ? Otherwise I don't understand the difference between limit of empirical (eigenvalue) measure and limiting spectral distribution (LSD). Thanks in advance. $\endgroup$
    – dohmatob
    Commented Mar 24, 2021 at 13:03
  • 1
    $\begingroup$ An individual eigenvalue does not affect the limit of empirical measure. As an example, take a sequence where $n-1$ eigenvalues are 0 and one is 1. Then, the empirical measure is $(1-1/n)\delta_0+\delta_1/n$. Then, the limit (under the weak topology) when $n\to\infty$ is $\delta_0$ and does not see the eigenvalue at $1$. $\endgroup$
    – ofer zeitouni
    Commented Mar 25, 2021 at 0:49

1 Answer 1

Reset to default
1
$\begingroup$

A heuristic argument based on Ofer's comment

Note that $T := YY^\top = XX^\top + AX^\top +X^\top A + AA^\top$, where $A:=a 1_n 1_d^\top$ is the all-ones matrix of shape $n \times d$, times the scalar $a \in \mathbb R$. Note that $AA^\top=da^21_n1_n^\top$.

Conjecture. $\lambda_i(YY^\top) \to \lambda_i(XX^\top) + \delta_{i=1}nda^2$.

I've empirically observed that the above conjecture is likely to be true. Below, I sketch some ideas which point in the direction of a possible proof.

enter image description here

Fact 1: $\mbox{LSD}(XX^\top + AX^\top +X^\top A + AA^\top) \to \mbox{LSD}(XX^\top + AA^\top)$, in some sense (probably weakly).

The above fact (it seems) was implied by Ofer's comment. I've observed this empirically, but I'm not quite sure why it should be true. Maybe this is a consequence of some classical / well-known lemma from free probability theory ?

Also, using a result due to G. Golub and V. Loan (see Lemma 1 of this monograph), we deduce that

Fact 2: There exist $q_1,\ldots,q_n \ge 0$ with $\sum_{i=1}^n q_i = 1$, such that $\lambda_i(XX^\top+AA^\top) = \lambda_i(XX^\top + \alpha^2d 1_n1_n^\top) = \lambda_i(XX^\top) + q_i nda^2$.

Moreover, we have the formula $q_i = \dfrac{(1_n^\top u_i)(1_n^\top v_i)}{nu_i^\top v_i}$ (provided $u_i^\top v_i \ne 0$), where $u_1,\ldots,u_n$ are the eigenvectors of $XX^\top$ and $v_1,\ldots,v_n$ are the eigenvector of $v_i$. Intuitively, one whould expect $u_i$ to be pretty much orthogonal to $v_i$ for $i \ne 1$. As a consequnce, one would expect $q \to \delta_{i=1} \in \ell^2$, in some sense.

Putting all the bits together (and of course making some of the above arguments rigorous) should prove the above claim / conjecture.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .