4
$\begingroup$

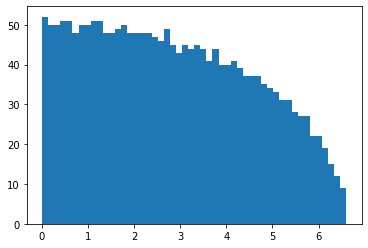
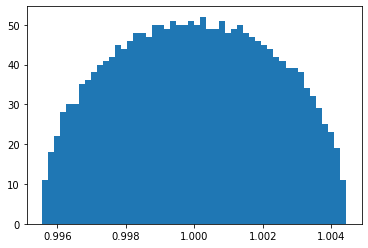
Let $A\in\mathbb R^{n\times n}$ be a random Gaussian matrix with i.i.d entries from $\mathcal N (0, \frac{a}{\sqrt{n}})$. By Marchenko-Pastur we know the limiting distribution of the eigenvalue of $A^TA$. Now I want to understand the eigenvalue behavior of $(I-A)^{T}(I-A)$. Based on some simulations, if $a$ is big, then the distribution converges to the quarter circle law (which is intuitive as the effect of $I$ shrinks), and a small $a$ leads to a deformed semicircle. Is there an analytical depiction of this distribution? Thanks.

a=10

a=0.01

Improve this question
$\endgroup$
1

1 Answer 1

Reset to default
0
$\begingroup$

I know that the limiting eigenvalue distribution satisfies a variational principle (see e.g. the joint work with A. Kuijlaars Large Deviations for a Non-Centered Wishart Matrix) from which you may derive a degree three algebraic equation for the Sieltjes transform, and eventually recover an analytic formula for the limiting density using the Cauchy–Plemelj inversion formula.

More generally, the limiting distribution can be expressed as the rectangular free convolution (introduced by Benaych-Georges in Rectangular random matrices, related convolution) of the Marchenko–Pastur distribution and a Dirac mass; next you can recover this algebraic equation using the associate rectangular R-transform.

I hope this helps and that you can work out the details by yourself. Otherwise, I'd start from the original Marchenko–Pastur proof and adapt it so as to compute the asymptotics of the Stieljes transform of your matrix model, eventually leading as well after tedious computations to the same degree three algebraic equation.

Improve this answer
$\endgroup$
2
  • $\begingroup$ These references are very helpful! To double check my understanding: 1) the free convolution of Marchenko-Pastur with a Dirac mass describes the eigenvalue distribution of $A^TA+I$, right? So the distribution I want is slightly different (which I believe is $(\sqrt{\mu_{MP}}\oplus \sqrt{\delta_1})^2$); and 2) the standard procedure after applying the R-transform is still to find the Stieljes transform and apply the Plemelj relation to find the pdf. Is this correct or am I overlooking something? Thanks. $\endgroup$
    – user3799934
    Commented Nov 6, 2021 at 15:15
  • $\begingroup$ 1) There are different free convolutions. The one you mention indeed describes the limiting distribution for A+B for asymptotically free A,B's (usually called the additive free convolution); there is also the mutiplicative free convolution for A^{1/2} B A^{1/2} and the rectangular one for (A+B)^T(A+B). I don't think you recover the law you mention. 2) This is indeed the approach I suggest. $\endgroup$
    – Adrien Hardy
    Commented Nov 8, 2021 at 8:24

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .