I was wondering if there is a classification of the indecomposable quiver representations of (not necessarily acyclic) quivers that are mutation equivalent to the $D_n$ Dynkin diagram. Such quivers are classified by Vatne, see https://arxiv.org/abs/0810.4789.
For a small example, what are the indecomposable representations of the "kite" quiver given by:
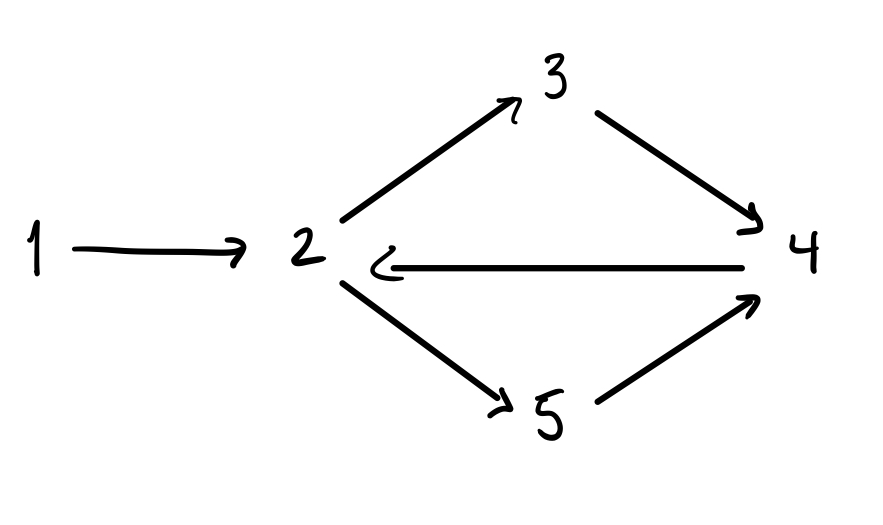
The quiver given in the question has five simple modules, six which correspond to a single arrow, and the remaining representations have support as follows: 123, 124, 125, 235, 345, 1235, 12235 (note the dimension over vertex 2 is 2 in this case), 2345, 12345 (note that the 42 arrow acts as zero in the last two).
Note that the 1235 subquiver is an acyclic subquiver of type $D_4$, so it has the 12 representations which Gabriel's theorem guarantees.
I think it is clear that there is an indecomposable representation corresponding to each of the dimension vectors I listed (though ask me if that's not clear). It isn't obvious that I have successfully listed all of them, but we know there must be as many as the number of non-initial cluster variables for $D_5$, so that justifies that there shouldn't be any others.
Edited, following Matt's suggstion, to add that I am imposing the relations that, for each arrow, the sum of the paths from the head of the arrow to the tail of the arrow is zero. These are the relations which define the cluster tilted algebra. (Without imposing those relations, the quiver has far more indecomposable representations -- there are infinitely many and, in fact, in a precise sense, there are so many as to be unclassifiable.)
Edited again to add that it is easy to classify all the representations for any "kite"-type quiver. That is to say, any quiver like the one drawn, but with a tail of $n-4$ vertices, instead of the tail of one vertex given by the OP.
To fix numbering, let $n$ be the vertex at the tip of the kite (numbered 4); let $n-3$, $n-4$, ..., $1$ be the tail of the kite, and let $n-1$ and $n-2$ be the other two vertices (3 and 5 in the picture).
The total is $n(n-1)$, which is the right answer, so this must be all of them.
Up to possibly learning some new technology, you can get a good answer to this question using the cluster category of type $D_n$ (or indeed of any Dynkin type). Given a Dynkin quiver $Q$, its associated cluster category $\mathcal{C}_Q$ which has finitely many indecomposable objects up to isomorphism, and it is possible to draw its Auslander–Reiten quiver, the vertices of which represent isoclasses of indecomposables and the arrows represent irreducible maps, via a completely combinatorial procedure. The original reference for this category is Tilting theory and cluster combinatorics by Buan, Marsh, Reineke, Reiten and Todorov.
(Roughly, you take the translation quiver $\mathbb{Z} Q$, which has one copy of $Q$ for each integer joined together by additional arrows $(i,n)\to (j,n+1)$ for each arrow $j\to i$ in $Q$, where $(i,n)$ is vertex $i$ in the $n$-th copy of $Q$, and $(j,n+1)$ is vertex $j$ in the $(n+1)$-st copy. Then you have to quotient out by a symmetry which depends on the Dynkin type of $Q$. This is explained in Section 1 of BMRRT and Figure 1 shows the result in the case that $Q$ is of type $A_3$.)
This category contains special objects, called cluster-tilting, and if $T$ is such an object, the endomorphism algebra $A_T=\operatorname{End}_{\mathcal{C}_Q}(T)$ (or its opposite, depending on your conventions) is presented by a quiver $Q'$ mutation equivalent to $Q$ (with relations, as Hugh indicated in the comments), and all quivers mutation equivalent to $Q$ appear in this way.
There is then an equivalence of categories $$\mathcal{C}_Q/(T)\simeq\operatorname{mod}{A_T}$$ where the left hand-side means $\mathcal{C}_Q$ modulo the ideal of morphisms which factor over a direct sum of copies of $T$, and the right-hand side is the category of finite-dimensional $A_T$-modules (i.e. representations of the quiver $Q'$ satisfying the appropriate relations). In this context I think this is due to Buan, Marsh and Reiten in Cluster-tilted algebras, but it has been generalised many times. (Sometimes, as in this reference, an automorphism of $\mathcal{C}_Q$ appears in front of $T$ on the left-hand side, giving a different but equivalent statement.) Incidentally, cluster-tilted algebras are by definition the algebras of the form $A_T$, for $T\in\mathcal{C}_Q$ cluster-tilting, and $Q$ Dynkin.
The payoff then is that if you have drawn the Auslander–Reiten quiver of $\mathcal{C}_Q$, and you can find in it the vertices corresponding to indecomposable summands of a cluster-tilting object $T$, then you can just delete these vertices (and all incident arrows) to get the Auslander–Reiten quiver of $A_T$. So not only do you 'classify' the indecomposable $A_T$-modules (by vertices of this quiver), you can also see all of the morphisms between them. It is also not so hard to work out from the Auslander–Reiten quiver of $\mathcal{C}_Q$ what each vertex really is as a quiver representation.
