Do morphisms of finitely-decomposable Quiver representations map indecomposables nicely?

Question

Consider two quivers $Q$ and $Q'$ of type $A_n$, laid out horizontally like so:

Given representations of $Q$ and $Q'$, Gabriel's theorem guarantees the existence of finitely many indecomposables for each. Now assume that we have a morphism of quiver representations, i.e., some vertical arrows from the representation of $Q$ to that of $Q'$ so that anything involving black arrows that can commute, does actually commute. For instance, given the system of black arrows below, the shaded triangle must commute:

Note that the "new" quiver so formed, consisting of $Q$, $Q'$ and all the intermediate black arrows, does not admit finitely many indecomposables because it does not have the type of a simply laced Dynkin diagram. Here's the question:

Is there any sense in which the morphism of representations maps indecomposables of $Q$ to those of $Q'$?

I suppose another way of asking is, "is Gabriel's theorem functorial", but I'm not quite sure how to categorify the indecomposables. Sorry if this is elementary, representation theory is not my field and I'm not sure where to look: I'm happy to delete if this is too easy for MO.

Answer 1

I can't say I understand your notion of "morphism of quiver representations," but I can tell you a canonical bijection between representations for one orientation and another, which might be what you're looking for.

Let $\bar Q$ be the doubled $A_n$ quiver, that is, the same set of dots with all arrows replaced by a pair of oppositely oriented arrows.

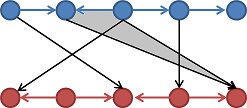
Given a representation $M$ of $Q$, consider the representations $E_M$ of $\bar Q$ on the same vector space that agree with $M$ on the arrows in $Q$, do whatever they want on the others, and have the property that the paths $i\to i-1 \to i$ and $i\to i+1\to i$ give the same self-map of $M_i$. Note that this is a set of linear equations on the choices for each new arrow in $\bar Q$, so its set of solutions is a linear space.

Now, consider the map of this linear space to the set of $Q'$-representations given by forgetting the arrows not in $Q'$. This isn't independent of our choices, but there's one isomorphism type that occurs generically in $E_M$ and all the others only show up in lower dimensional subvarieties So, consider the map $\varphi$ that sends a isomorphism class of $Q$ reps to the $Q'$ rep that occurs generically in $E_M$ after forgetting the arrows not in $Q'$.

Theorem. The map $\varphi$ is a bijection.

Note that this bijection is not extremely easy to describe combinatorially; for example, it is piecewise linear in the multiplicity of the different indecomposables in your representation.

EDIT: Why does this work? Well, by definition, a preprojective lift of a $Q$-rep is an element of the conormal bundle to the $G$-orbit (as usual, $G$ is the product of $GL_n$'s acting on the spaces of the quiver rep) through that point. Since $G$ has finitely many orbits (Dynkin type is very necessary here!), the space of preprojective representations is the union of the conormal bundles to $G$-orbits, and each component is the closure of exactly one of these (this is 14.2 in Lusztig's "Quivers, perverse sheaves and quantized universal enveloping algebras"). Thus, there is a canonical bijection between preprojective components and isomorphism types of $Q$-reps. One map is close up the conormal bundle to the space of reps of that type, the opposite is grab a generic element of the component and forget the arrows that aren't in $Q$.

So the bijection I described is applying one of these maps for $Q$ and the other for $Q'$.

Answer 2

There is another way to relate representations of $Q$ to representations of $Q'$: reflection functors. These are quite easy to describe combinatorially. One downside is that the way they work is by making a small modification to the quiver, so that if you want to make a more substantial modification to the quiver, you will need to apply a composition of reflection functors.

A reflection functor is defined like this. Let $Q$ be a quiver, and let $i$ be a vertex of $Q$, and let $V$ be a representation. We assume that $i$ is a source (i.e., all arrows incident to $i$ point towards $i$). It is also possible to assume, dually, that $i$ is a sink. If you want to allow $i$ to be neither a source nor a sink, life is quite a bit more complicated.

Let $Q'$ be the quiver obtained by reversing all the arrows at $i$. Define a representation $V'$ of $Q'$ by putting the corresponding vector spaces of $V$ at all the vertices of $Q'$ except $i$. At $i$, you put $$V'_i = \textrm{cokernel}(V_i \rightarrow \bigoplus_{i\rightarrow j} V_j).$$ The direct sum is over the arrows out of $i$ in $Q$.

The maps are the same as in $V$, except that, for the arrows into $i$, we put the natural projections from $V_j$ to the cokernel.

Note that the dimension vector of the new representation will typically not equal the dimension vector of the old representation. (This is a difference from what Ben described.)

Indecomposable representations are taken to indecomposable representations, except that the simple representation at $i$ is sent to the zero representation. For all other indecomposable representations, their dimensions are multiplied by a simple reflection at $i$ in the Weyl group corresponding to $Q$.

Reflection functors go back to a 1973 paper of Bernstein-Gelfand-Ponomarev, which is still not a bad source. They are also described in textbooks, such as that of Assem-Simson-Skowronski, which I recommend.