I was wondering if there is a classification of the indecomposable quiver representations of (not necessarily acyclic) quivers that are mutation equivalent to the $D_n$ Dynkin diagram. Such quivers are classified by Vatne, see https://arxiv.org/abs/0810.4789.
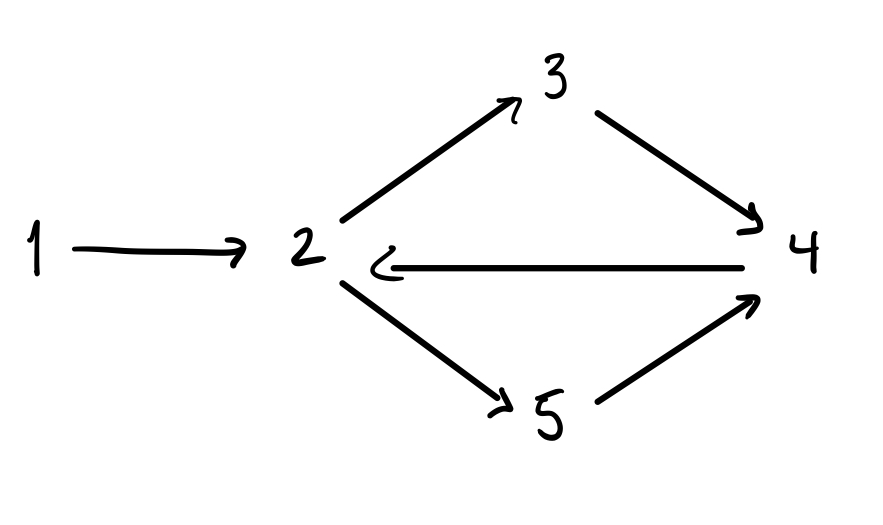
For a small example, what are the indecomposable representations of the "kite" quiver given by: $$ Q= [[1,2],[2,3],[3,4],[4,2],[2,5],[5,4]] $$