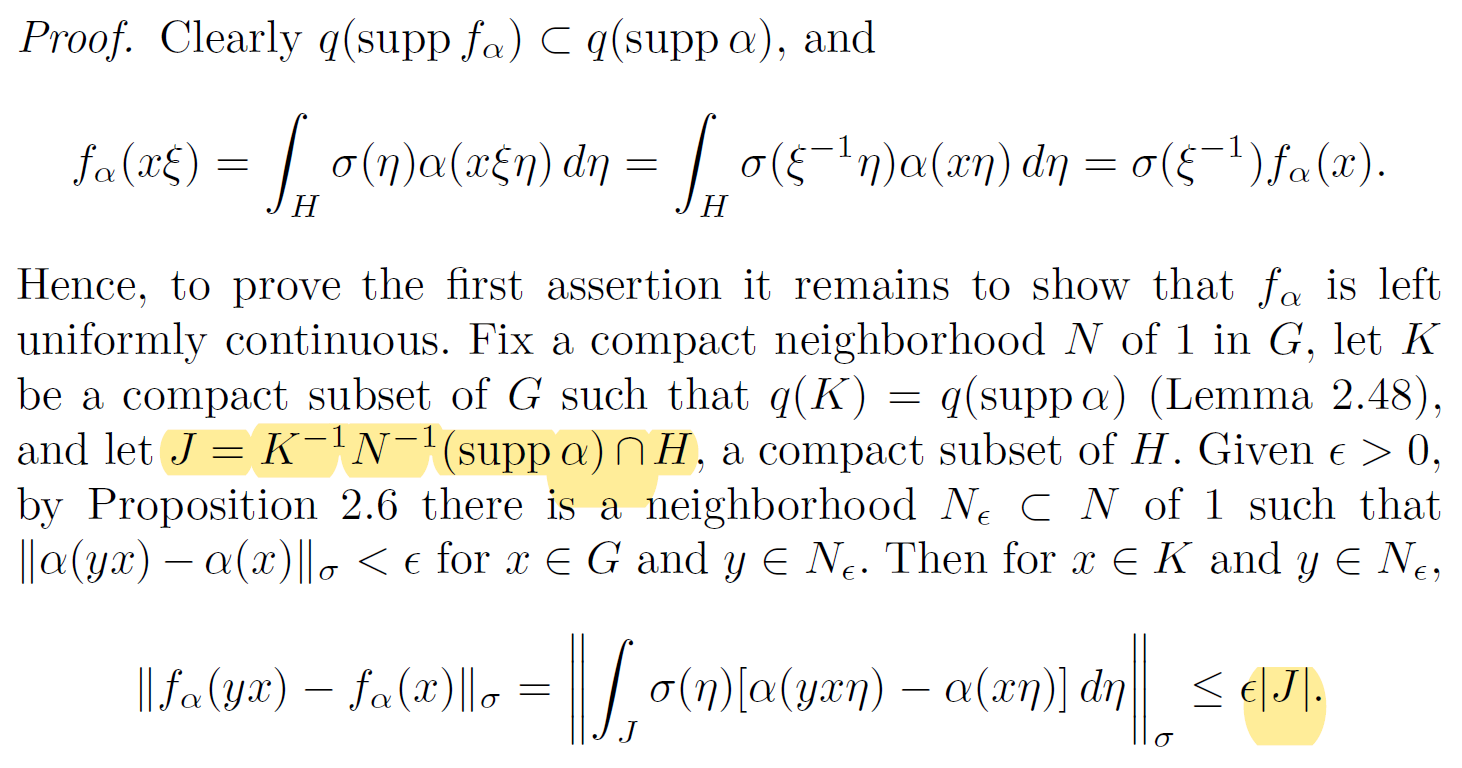Induced representations: space of continuous functions on $G$ to a Hilbert space

Question

This question was asked in math stack exchange but received no replies:
https://math.stackexchange.com/questions/4000655/induced-representations-space-of-continuous-functions-on-g-to-a-hilbert-space

If this post violates community rules, please remark and I'll remove it.

---

Let $G$ be a locally compact group, $H$ a closed subgroup, $q:G\rightarrow G/H$ the canonical quotient map, and $\sigma$ a unitary representation of H on $\mathcal{H}_{\sigma}$. We denote the norm and inner product on $\mathcal{H}_{\sigma}$ by $\left\Vert u\right\Vert _{\sigma}$ and $\left\langle u,v\right\rangle _{\sigma}$, and we denote by $C(G,\mathcal{H}_{\sigma})$ the space of continuous functions from $G$ to $\mathcal{H}_{\sigma}$.

Let $\mathcal{F}_{0}=\{ f\in C(G,\mathcal{H}_{\sigma}):q(supp f)$ compact, $f(x\xi)=\sigma(\xi^{-1})f(x)$ for $x\in G,\:\xi\in H\}$.

Proposition. If $\alpha:G\rightarrow\mathcal{H}_{\sigma}$ is continuous with compact support, then the function $f_{\alpha}(x)=\int_{H}\sigma(\eta)\alpha(x\eta)d\eta$ belongs to $\mathcal{F}_{0}$ and is left uniformly continuous on $G$. Moreover, every element of $\mathcal{F}_{0}$ is of the form $f_{\alpha}$ for some $\alpha\in C_{c}(G,\mathcal{H}_{\sigma})$.

The following questions refer to the attached section below:

1. Why is $J$ (first yellow part) defined this way? why not just $(supp \alpha)\cap H$?
2. Regarding the second yellow part, it might be a silly question, but what if $|J|$ is infinity?

Attached is the relevant section of the proof from A Course in Abstract Harmonic Analysis by Gerald B. Folland, 2nd edition, prop 6.1 (Note that proposition 2.6, mentioned in the text, refers to the fact that $\alpha$ is uniformly continuous):

Answer 1

1. We need $J$ to have the property that in the formula for $f_\alpha$ it suffices to integrate over $J$ (as opposed to all of $H$). That is needed for the first equality in the final displayed formula. Thus we need $\alpha(yx\eta) = \alpha(x\eta) = 0$ for $\eta$ outside of $J$, which is to say that for any $y \in N_\epsilon$ and $x \in K$, we need $yx\eta$ not to lie in the support of $\alpha$ whenever $\eta$ lies outside of $J$.

2. $J$ is compact, so its measure is finite.

(The question does belong on math.stackexchange, but if no one answers it there I think it's okay to ask it here.)