3
$\begingroup$

Rigid indecomposable modules in the category ${\rm CM}(A)$ of Cohen-Macaulay $A$-module are parametrized by certain arrays of integers called profiles as shown in the paper A categorification of Grassmannian cluster algebras. For example, $P_1' = \begin{array}{cccc} 2 & 4 & 7 & 9 \\ \hline 1 & 3 & 5 & 8 \\ \hline 2 & 4 & 6 & 9 \end{array}$ is a profile. We have that $\tau(1357)=\begin{array}{cccc} 2 & 4 & 7 & 9 \\ \hline 1 & 3 & 5 & 8 \\ \hline 2 & 4 & 6 & 9 \end{array}$, where $\tau$ is the Auslander-Reiten translation.

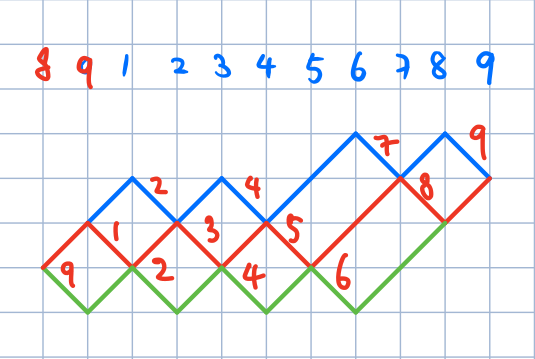
We translate the array of integers $P_1'$ into another array of integers as follows. We draw the picture of $P_1'$ as follows (in the ith path of the picture, the down steps are the numbers in the ith row of $P_1'$, cf. Figure 9 of A categorification of Grassmannian cluster algebras).

enter image description here

I draw the picture in a way such that the pictures is formed by closed boxes. Now we define a new array of integers $P_1$ by requiring the ith column of $P_1$ is the numbers appearing in the $(m-i)$th (in the above example, $m=3, n=9$) path of the picture of $P_1'$. Then $P_1 = \left( \begin{matrix} 9 & 1 & 2 \\ 2 & 3 & 4 \\ 4 & 5 & 7 \\ 6 & 8 & 9 \end{matrix} \right)$.

On the other hand, Marsh and Scott defined a twist map on Grassmannian in the paper. The twist of the Plucker coordinate $P_{1,3,5,7}$ gives a tableau $T_1 = \left(\begin{array}{ccc} 1 & 2 & 4\\ 2 & 3 & 7\\ 4 & 5 & 8\\ 6 & 9 & 9 \end{array}\right)$. The twist map corresponds to Auslander-Reiten translation. Therefore $P_1$ and $P_1'$ should correspond to $T_1$.

The shift of a profile by $1$ (adding $1$ to each number mod $n$) will correspond to the promotion of the corresponding tableau.

Let $P_1, \ldots, P_9$ be the following arrays of integers: \begin{align*} \left( \begin{matrix} 9 & 1 & 2 \\ 2 & 3 & 4 \\ 4 & 5 & 7 \\ 6 & 8 & 9 \end{matrix} \right), \left( \begin{matrix} 1 & 2 & 3 \\ 3 & 4 & 5 \\ 5 & 6 & 8 \\ 7 & 9 & 1 \end{matrix} \right), \left( \begin{matrix} 2 & 3 & 4 \\ 4 & 5 & 6 \\ 6 & 7 & 9 \\ 8 & 1 & 2 \end{matrix} \right), \left( \begin{matrix} 3 & 4 & 5 \\ 5 & 6 & 7 \\ 7 & 8 & 1 \\ 9 & 2 & 3 \end{matrix} \right), \left( \begin{matrix} 4 & 5 & 6 \\ 6 & 7 & 8 \\ 8 & 9 & 2 \\ 1 & 3 & 4 \end{matrix} \right), \left( \begin{matrix} 5 & 6 & 7 \\ 7 & 8 & 9 \\ 9 & 1 & 3 \\ 2 & 4 & 5 \end{matrix} \right), \left( \begin{matrix} 6 & 7 & 8 \\ 8 & 9 & 1 \\ 1 & 2 & 4 \\ 3 & 5 & 6 \end{matrix} \right), \left( \begin{matrix} 7 & 8 & 9 \\ 9 & 1 & 2 \\ 2 & 3 & 5 \\ 4 & 6 & 7 \end{matrix} \right), \left( \begin{matrix} 8 & 9 & 1 \\ 1 & 2 & 3 \\ 3 & 4 & 6 \\ 5 & 7 & 8 \end{matrix} \right). \end{align*} $P_i$ is obtained from $P_{i-1}$ by adding $1$ (mod $9$) to every entry of $P_{i-1}$.

These arrays of integers correspond to the following semistandard Young tableaux $T_1, \ldots, T_9$ respectively (these tableaux can be obtained from each other by promotion): \begin{align*} & \left(\begin{array}{ccc} 1 & 2 & 4\\ 2 & 3 & 7\\ 4 & 5 & 8\\ 6 & 9 & 9 \end{array}\right),\left(\begin{array}{ccc} 1 & 1 & 3\\ 2 & 4 & 5\\ 3 & 6 & 8\\ 5 & 7 & 9 \end{array}\right),\left(\begin{array}{ccc} 1 & 2 & 4\\ 2 & 3 & 6\\ 4 & 5 & 7\\ 6 & 8 & 9 \end{array}\right),\left(\begin{array}{ccc} 1 & 3 & 5\\ 2 & 4 & 7\\ 3 & 6 & 8\\ 5 & 7 & 9 \end{array}\right),\left(\begin{array}{ccc} 1 & 2 & 4\\ 3 & 5 & 6\\ 4 & 7 & 8\\ 6 & 8 & 9 \end{array}\right), \\ & \left(\begin{array}{ccc} 1 & 3 & 5\\ 2 & 4 & 7\\ 5 & 6 & 8\\ 7 & 9 & 9 \end{array}\right),\left(\begin{array}{ccc} 1 & 1 & 4\\ 2 & 5 & 6\\ 3 & 7 & 8\\ 6 & 8 & 9 \end{array}\right),\left(\begin{array}{ccc} 1 & 2 & 5\\ 2 & 3 & 7\\ 4 & 6 & 8\\ 7 & 9 & 9 \end{array}\right),\left(\begin{array}{ccc} 1 & 1 & 3\\ 2 & 4 & 6\\ 3 & 7 & 8\\ 5 & 8 & 9 \end{array}\right). \end{align*}

I think that there is a combinatorial rule to obtain $T_i$ from $P_i$. We can move elements which are not in the correct position to a correct position. It seems that it is similar to Jeu de taquin. But I have not figured out that. Any help would be greatly appreciated. Thank you very much.

Improve this question
$\endgroup$
9
  • $\begingroup$ In what way can you get the SSYT from the arrays? There are several ways to produce SSYTs from arrays... $\endgroup$
    – Per Alexandersson
    Commented Dec 22, 2020 at 18:48
  • $\begingroup$ @Per Alexandersson, thank you very much. For $P_1$, we can first reorder each row from small to large. Then we reorder each column from small to large. And we move some numbers down when a column is not strictly increasing. But this does not work for $P_3$. We need to interchange $7$ and $8$ in the end. Do you have some uniform rule which work for every $P_i$? $\endgroup$
    – Jianrong Li
    Commented Dec 22, 2020 at 21:04
  • $\begingroup$ I do not think I understand your question then - it sounds that you have some uniform rule to get T_i from the T_i, and is looking for some more straightforward one... One way to produce SSYTs from a list of numbers is to use RSK insertion. Another way, to get pairs of SSYTs from integer arrays is also to use a variant of RSK insertion... $\endgroup$
    – Per Alexandersson
    Commented Dec 22, 2020 at 21:19
  • $\begingroup$ I think that Jianrong's point when they say the arrays "correspond" to the SSYT is that the contents (i.e., numbers of each entry) match up the arrays and tableaux. Of course, promotion rotates content, just like adding $1$ mod $9$ to each entry does. $\endgroup$
    – Sam Hopkins
    Commented Dec 23, 2020 at 5:15
  • $\begingroup$ @Per Alexandersson, thanks. Yes, exactly. The rule I know to get T_i from P_i is not complete. So I am trying to find a some more straightforward rule. $\endgroup$
    – Jianrong Li
    Commented Dec 23, 2020 at 7:54

0

Reset to default

You must log in to answer this question.

Browse other questions tagged .