1
$\begingroup$

I am reading a paper called A NOTE ON THE RADICAL OF A MODULE CATEGORY by CLAUDIA CHAIO AND SHIPING LIU. This is part of the proof of Lemma 2.3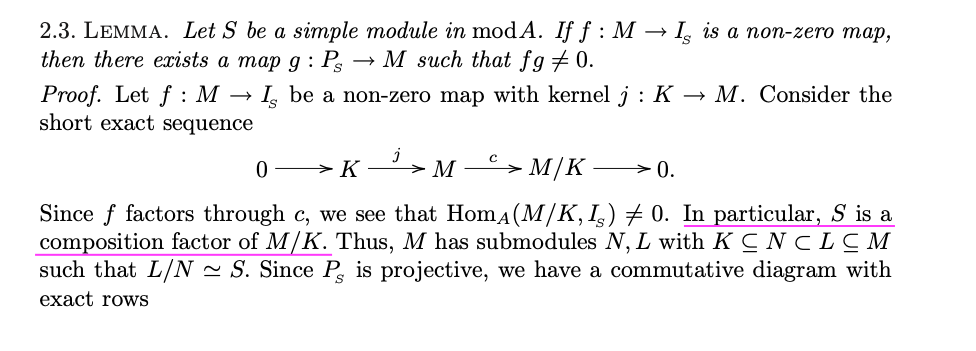enter image description here

$A$ is assumed to be an Artin algebra and mod(A) the category of finitely generated $A$ - modules. By $S \rightarrow I_s$ one means the injective hull of a simple module $S$. $P_s \rightarrow S$ denoted the projective cover of $S$.

The sentence underlined in pink confuses me. How does it follow that $S$ is a composition factor of $M/K$? Any help is appreciated!

Improve this question
$\endgroup$
4
  • $\begingroup$ Let $f:M/K\rightarrow I_S$ be a nonzero homomorphism, and view $S\subset I_S$. By definition of an injective hull $f^{-1}(S)$ is a nonzero submodule of $M/K$, which maps surjectively to $S$. $\endgroup$
    – abx
    Commented Dec 21, 2020 at 13:11
  • $\begingroup$ @abx I'm afraid I don't quite understand your argument. How does it follow from definition that $f^-1(S)$ is a nonzero submodule of $M/K$? And why does it follow that if a submodule of $M/K$ maps surjectively to $S$, that $S$ is a composition factor of $M/K$? $\endgroup$
    – mathStudent
    Commented Dec 21, 2020 at 13:37
  • $\begingroup$ 1) $S\subset I_S$ injective hull means that $I_S$ is injective and every nonzero submodule $M$ of $I_S$ meets nontrivially $S$, hence contains it since $S$ is simple. $\endgroup$
    – abx
    Commented Dec 21, 2020 at 13:59
  • 1
    $\begingroup$ 2) Let $N\subset M/K$ such that $f(N)=S$, and let $P:=\operatorname{Ker}(f)\cap N\ $. Then $\ \ P\subset N\subset M/K\ \ $ and $\ \ N/P\cong S\ \ $. 3) The question, and this discussion, would be more appropriate on MSE. $\endgroup$
    – abx
    Commented Dec 21, 2020 at 13:59

1 Answer 1

Reset to default
1
$\begingroup$

Let $A$ be an Artin algebra, $S$ a simple $A$-module, and $M$ a finitely generated $A$-module. Then $\operatorname{dim}\operatorname{Hom}(P_s,M)$ and $\operatorname{dim}\operatorname{Hom}_A(M,I_s)$ are both equal to the number of composition factors of $M$ isomorphic to $S$. I am not immediately able to find a good reference for this, but it is very often used, and not so hard to prove.

A more direct argument for the precise statement you want uses that $I_S$ has socle $S$, so any non-zero submodule of $I_S$ has $S$ as a composition factor. In particular, this applies to the image of any non-zero morphism $M/K\to I_S$. The composition factors of the image of a morphism are a subset of those of its domain, so $M/K$ must have $S$ has a composition factor.

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged .