Is there some analytic expression or even an approximation of the definite 2D Gaussian integral of the form: $$E=\int_a^b Dg \int_{cg+d}^\infty Dh$$ where $Dg=\frac{dg}{\sqrt{2 \pi}} e^{-g^2/2}$ and a,b,c,d are real numbers. That is, the boundary of the inner integral is a linear function of the outer integration variable.
1 Answer
$\begingroup$
$\endgroup$
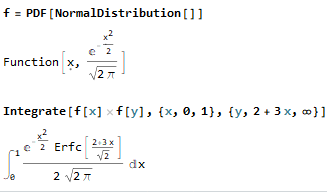
An explicit expression is extremely unlikely. Mathematica cannot do anything with this integral even for specific values of $a,b,c,d$:
As for an approximation, it all depends on what kind(s) of $a,b,c,d$ you are dealing with and what your specific goals are. Of course, you can always approximate the integral numerically with any given degree of accuracy.