1
$\begingroup$

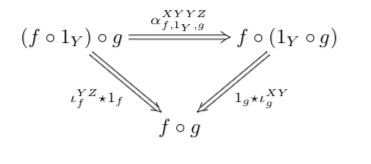
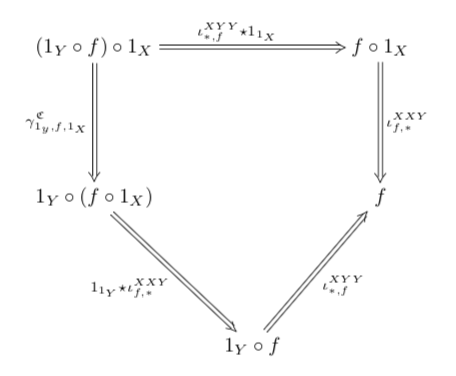
In the standard definition of a bicategory, the unitors are required to satisfy the 'triangle identity'

enter image description here

for any composable $1$-cells $f:Y\to Z,gX\to Y$. But it seems like we also want

to commute for any $1$-cell $f:X\to Y$. (apologies for notational differences between diagrams, they're from two different note files. $\alpha$ and $\gamma$ are both just associators)

Does this pentagon commuting follow from the triangle identities? Does this pentagon commuting imply the triangle identities?

Any assistance is appreciated.

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ This pentagon must follow from the usual definition of bicategory; Lemma 2.2 here may help you prove that: ncatlab.org/nlab/show/… $\endgroup$
    – John Baez
    Commented Nov 26, 2020 at 22:46
  • $\begingroup$ @JohnBaez Much appreciated John. $\endgroup$
    – Alec Rhea
    Commented Nov 28, 2020 at 19:20

1 Answer 1

Reset to default
3
$\begingroup$

Draw the unitor from $(1_Y \circ f) \circ 1_X$ to $1_Y \circ f$, splitting your pentagon into a triangle and a square. The square is a naturality square, so it commutes. The triangle is an instance of the dual form of Lemma 2.2 at the link that John provided.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thank you Mike. $\endgroup$
    – Alec Rhea
    Commented Nov 28, 2020 at 18:32

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .