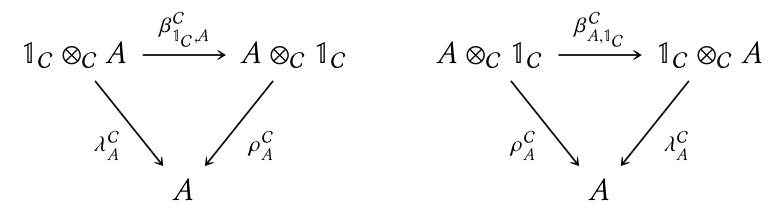Coherence $2$-cells in braided monoidal bicategories

Question

In a braided monoidal category $(\mathcal{C},\otimes_{\mathcal{C}},\mathbf{1}_{\mathcal{C}},\alpha,\lambda,\rho,\beta)$, we have $\beta_{\mathbf{1}_{\mathcal{C}},\mathbf{1}_{\mathcal{C}}}=\mathrm{id}_{\mathbf{1}_{\mathcal{C}}\otimes_{\mathcal{C}}\mathbf{1}_{\mathcal{C}}}$ and the diagrams

commute.

In a braided monoidal bicategory $(\mathcal{C}$, $\otimes_{\mathcal{C}}$, $\mathbf{1}_{\mathcal{C}}$, $(\alpha,\alpha^{\bullet},\phi,\phi^{\bullet})$, $(\lambda,\lambda^{\bullet},\eta,\eta^{\bullet})$, $(\rho,\rho^{\bullet},\epsilon,\epsilon^{\bullet})$, $\pi\mspace{-9.5mu}\pi$, $\mu\mspace{-10.0mu}\mu$, $\lambda\mspace{-10.0mu}\lambda$, $\rho\mspace{-10.0mu}\rho$, $(\beta,\beta^{\bullet},\gamma,\gamma^{\bullet})$, $R_{-,-|-}$, $R_{-|-,-})$, these identities should be replaced by invertible $2$-cells $$ \begin{align*} \mathrm{id}_{\mathbf{1}_{\mathcal{C}}\otimes_{\mathcal{C}}\mathbf{1}_{\mathcal{C}}} &\Longrightarrow \beta_{\mathbf{1}_{\mathcal{C}},\mathbf{1}_{\mathcal{C}}}\\ \lambda_{A} &\Longrightarrow \rho_{A}\circ\beta_{\mathbf{1}_{\mathcal{C}},A},\\ \rho_{A} &\Longrightarrow \lambda_{A}\circ\beta_{A,\mathbf{1}_{\mathcal{C}}}, \end{align*} $$ similarly to how the identity $\lambda_{\mathbf{1}_{\mathcal{C}}}=\rho_{\mathbf{1}_{\mathcal{C}}}$ in a monoidal category is replaced by an invertible $2$-cell $\theta\colon\lambda_{\mathbf{1}_{\mathcal{C}}}\Longrightarrow\rho_{\mathbf{1}_{\mathcal{C}}}$ in a monoidal bicategory (see [Enriched categories as a free cocompletion, Lemma 2.1]).

Question: How are the above $2$-cells constructed from the data $(\mathcal{C},\ldots,R_{-|,-,-})$?

Answer 1

I found a reference which discusses precisely this issue:

[Hou07] Robin Houston. ‘Linear Logic without Units’. PhD thesis. University of Manchester, 2007, p. 213. URL:https://arxiv.org/abs/1305.2231

There, you find the following remark at the start of Section 3.3:

These definitions [of braided monoidal bicategories in the earlier literature] both take the unit to be strict ― in terms of our definition below, they take the $1$-cells $s_{A,\mathbb{I}}$ and $s_{\mathbb{I},A}$ and the $2$-cells $U_{A|\mathbb{I}}$ and $U_{\mathbb{I}|A}$ to be identities [these are the cells this question asks about]. The justification for such a restriction is presumably the reasonable expectation that a coherence theorem for tetracategories would show every braided monoidal bicategory to be suitably equivalent to one with such a strict unit. Since an expectation, however reasonable, is not a proof,we have opted to eschew the modest simplification that such a restriction brings. Thus the definition below is conceivably the first that includes all the structure known to be necessary at its natural level of strictness, though we do not claim that any new insight was needed to formulate it. (It is not unimaginable that further axioms should yet be found wanting, though the results of Chapter 4 provide strong evidence that these axioms do suffice.)

Houston then goes on to define braided monoidal bicategories including the $2$-cells $\sigma\mspace{-11.mu}\sigma_{\mathbf{1}|A}\colon\lambda_{A}\Longrightarrow\rho_{A}\circ\beta_{\mathbf{1}_{\mathcal{C}},A}$ and $\sigma\mspace{-11.0mu}\sigma_{A|\mathbf{1}}\colon\rho_{A}\Longrightarrow\lambda_{A}\circ\beta_{A,\mathbf{1}_{\mathcal{C}}}$ as part of the data. These are required to satisfy coherence conditions, which Houston states for $\mathsf{Gray}$-monoids.

Here's the part of their definition (Definition 3.9) relevant to this question (the rest of it goes as usual, say as in [Johnson–Yau, Definition 12.1.6]):

Definition. A braided monoidal bicategory $(\mathcal{C}$, $\otimes_{\mathcal{C}}$, $\mathbf{1}_{\mathcal{C}}$, $(\alpha,\alpha^{\bullet},\phi,\phi^{\bullet})$, $(\lambda,\lambda^{\bullet},\eta,\eta^{\bullet})$, $(\rho,\rho^{\bullet},\epsilon,\epsilon^{\bullet})$, $\pi\mspace{-9.5mu}\pi$, $\mu\mspace{-10.0mu}\mu$, $\lambda\mspace{-10.0mu}\lambda$, $\rho\mspace{-10.0mu}\rho$, $(\beta,\beta^{\bullet},\gamma,\gamma^{\bullet})$, $R_{-,-|-}$, $R_{-|-,-}$, $\sigma\mspace{-11.mu}\sigma_{\mathbf{1}|A}$, $\sigma\mspace{-11.mu}\sigma_{A|\mathbf{1}})$ consists of

• [...] (usual data in a braided monoidal bicategory, as defined in [Johnson–Yau, Definition 12.1.6]);
• Left Triangulators. An invertible modification

with components

• Right Triangulators. An invertible modification

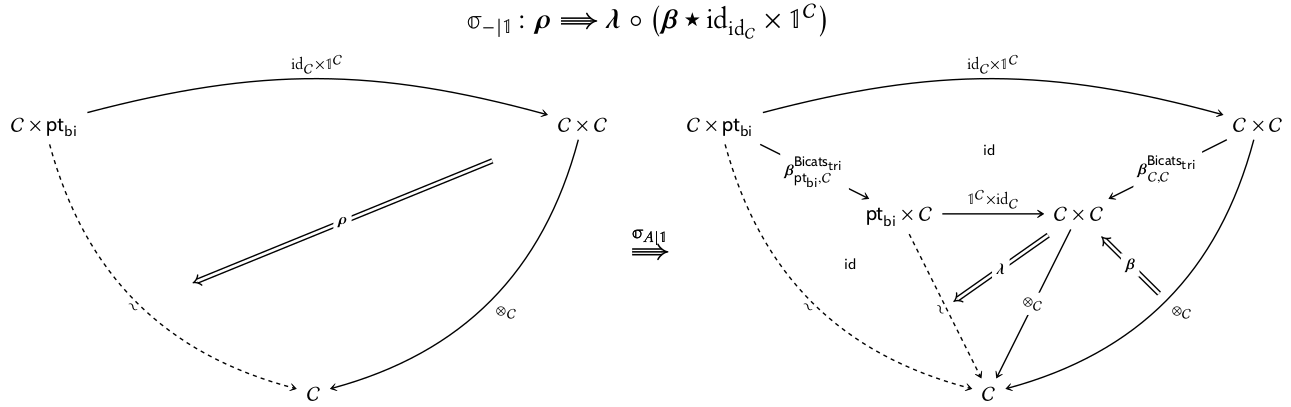
with components

satisfying the following conditions:

• [...] (usual requirements for braided monoidal bicategories ($(1,3)$-crossing, $(3,1)$-crossing, $(2,2)$-crossing, and Yang–Baxter), as defined in [Johnson–Yau, Definition 12.1.6]);
• We require the $2$-cell $\sigma\mspace{-11.mu}\sigma_{\mathbf{1}|A}$ to be compatible with the left hexagonator $R_{-,-|-}$ of $\mathcal{C}$ in that we have an equality

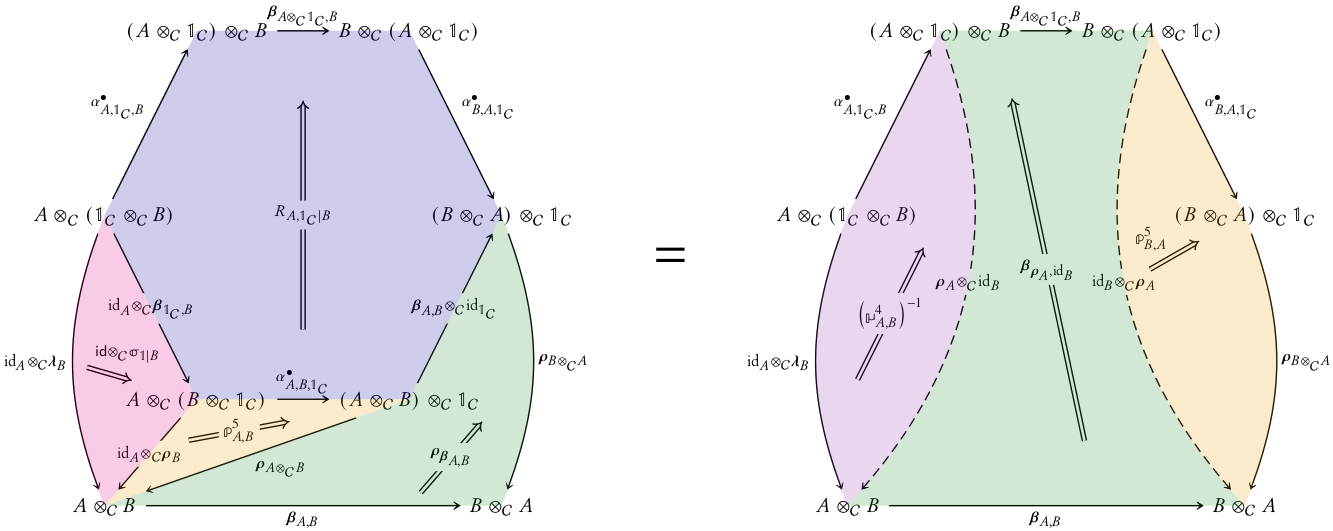
of pasting diagrams in $\mathcal{C}$.

• We require the $2$-cell $\sigma\mspace{-11.mu}\sigma_{A|\mathbf{1}}$ to be compatible with the right hexagonator $R_{-|-,-}$ of $\mathcal{C}$ in that we have an equality

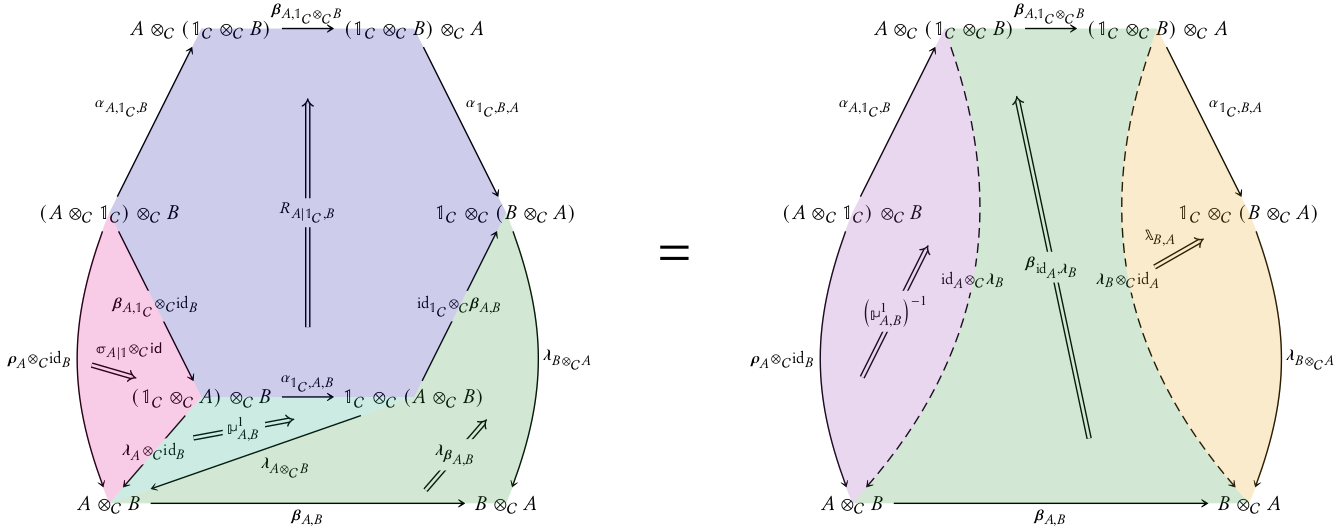
of pasting diagrams in $\mathcal{C}$.

Finally, Houston proves some extra coherence conditions for the "triangulators" $\sigma\mspace{-11.mu}\sigma_{\mathbf{1}|A}$ and $\sigma\mspace{-11.mu}\sigma_{A|\mathbf{1}}$, again in the $\mathsf{Gray}$-setting (Propositions 3.12 and 3.13). I believe these take the following forms for bicategories, where the first two of these relate the triangulators and hexagonators of $\mathcal{C}$ again, and the last two relate the triangulators with themselves ("applying $\sigma$ twice is the same as applying $\sigma$ once and $R_{-|-,-}$"):

• We have equalities

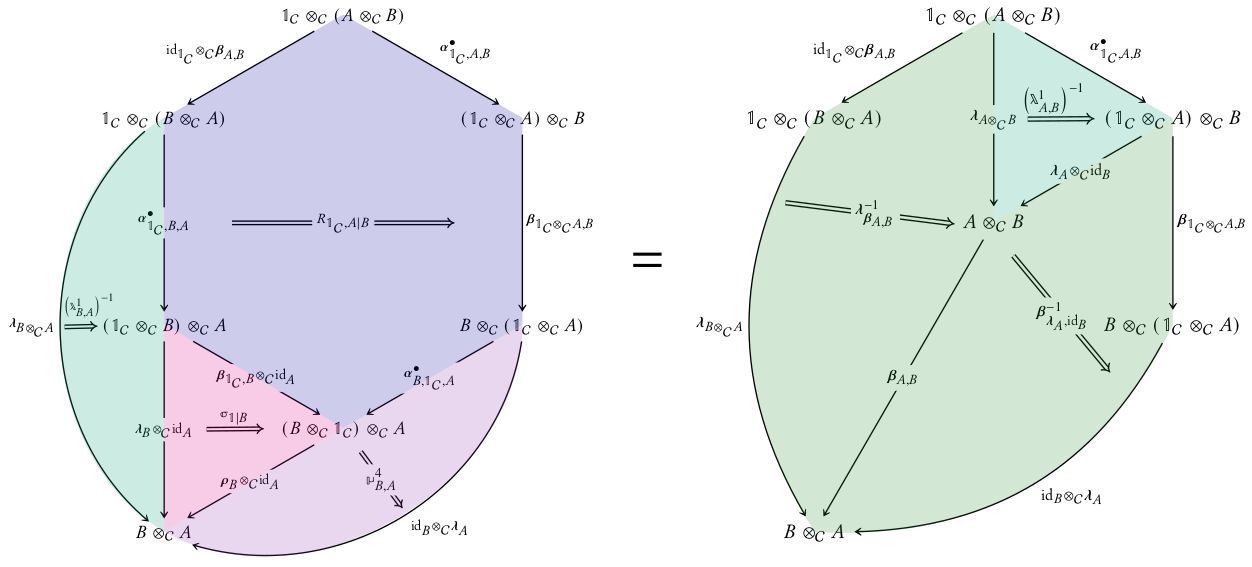
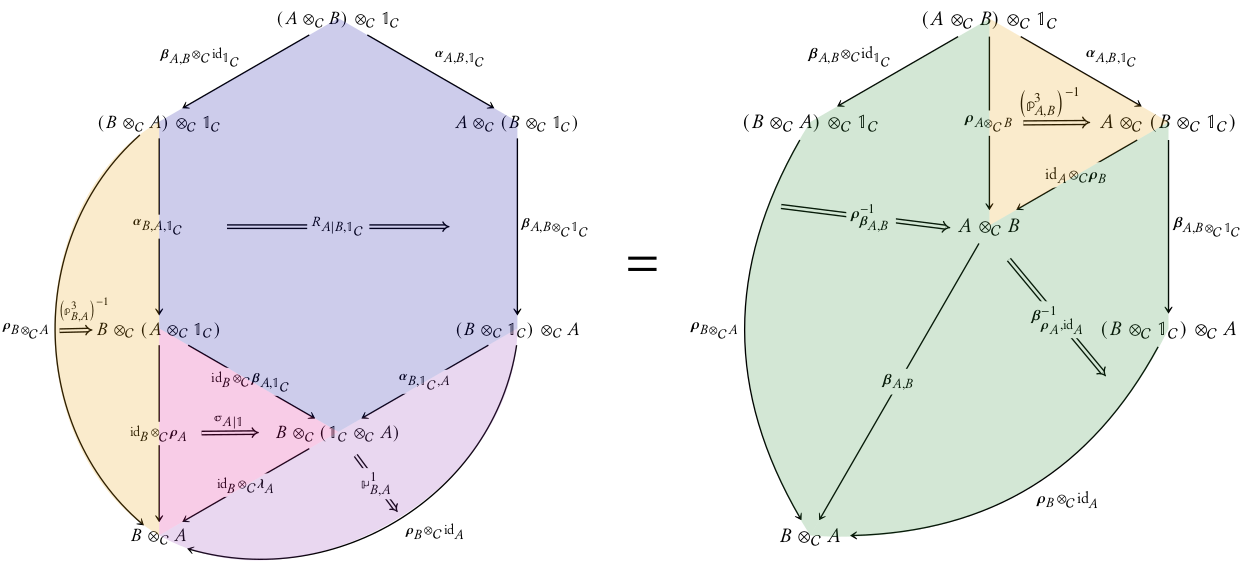
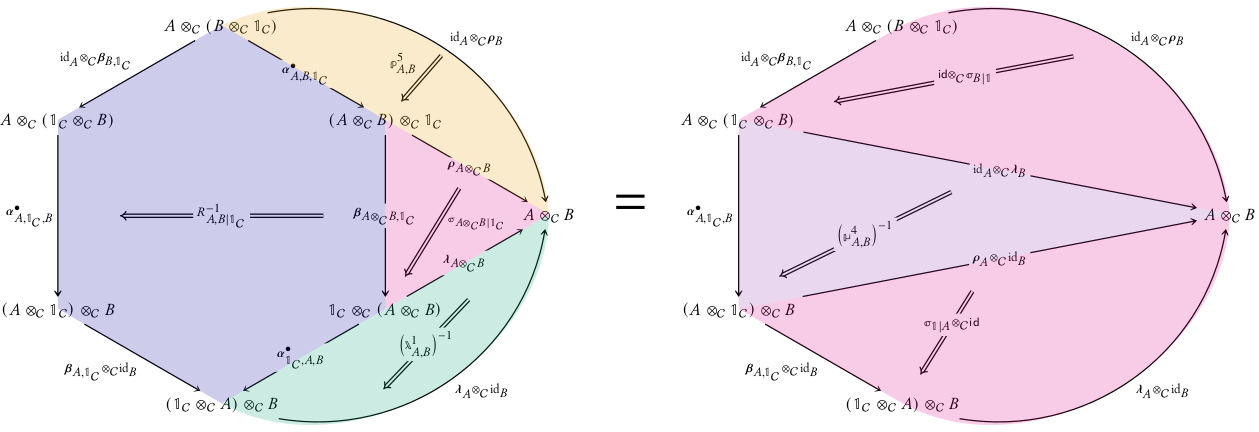
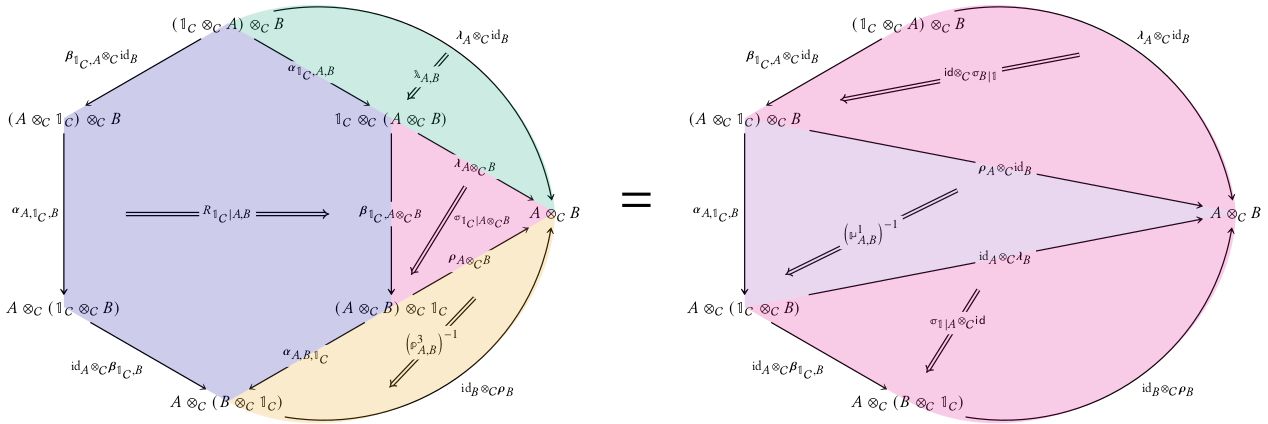
of pasting diagrams in $\mathcal{C}$.