This question was asked on MSE a year ago. Motivation for this question can be found in other MSE questions here, here or here.
Convex solids can have all sorts of symmetries:
the platonic solids are vertex and face-transitive, meaning there is a subgroup of the rotations of 3-dimensional space which can bring any vertex onto another one (and the same for faces). The list there is limited to the 5 platonic solids.
face transitive (or isohedral) solids include the Catalan solids, the (infinite family) of dipyramids and the (infinite family) of trapezohedra. Note that without further restricitions these solids can come in infinite families: the rhombic dodecahedron has an infinite number of deltoidal cousins (see deltoidal dodecahedron); it also fits in a one-parameter family of dodecahedra called pyritohedra; the dodecahedron and the triakis tetrahedron fit in the one-parameter family called tetartoid; dipyramids and trapezohedron also admit all sorts of deformations beside the number of faces.
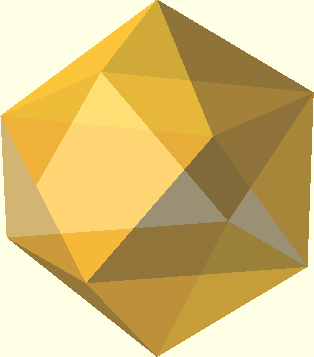
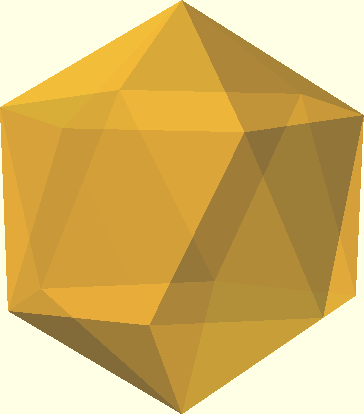
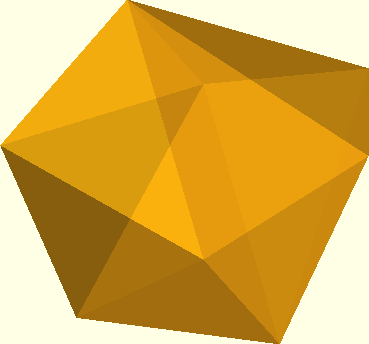
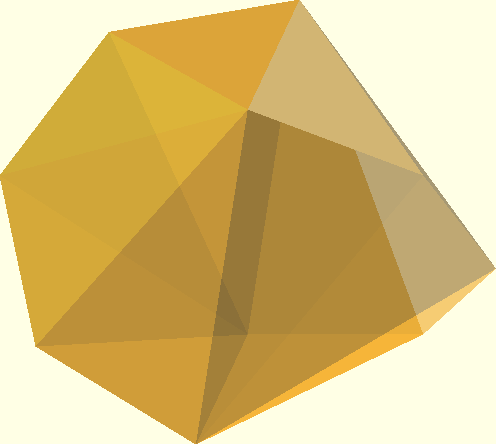
there is a much weaker symmetry one can ask for. Let's call it pseudo-Catalan (for lack of a better name). Fix a "centre" $C$. The convex solid is pseudo-Catalan, if each face can be sent to another face by a rotation with centre $C$ or a reflection (whose plane goes through $C$). Note that there is no requirement that this rotation (+ reflection) preserve the whole solid. An example of such a solid which is not a Catalan solid is the gyrate deltoidal icositetrahedron.
Question: is there a list of solids which are pseudo-Catalan but not Catalan? [More desperately: is there any such solid beside the gyrate deltoidal icositetrahedron?]
- note that there would be a last category, where the solid is convex and all the faces are congruent (a convex monohedral solid). The difference with the previous category is that translations are now allowed. In particular, to check that a solid belongs to the previous category, the choice of $C$ (and the fact that all rotations and reflections are constrained by this point) is important. Examples of such solids are the the triaugmented triangular prism and the gyroelongated square dipyramid.