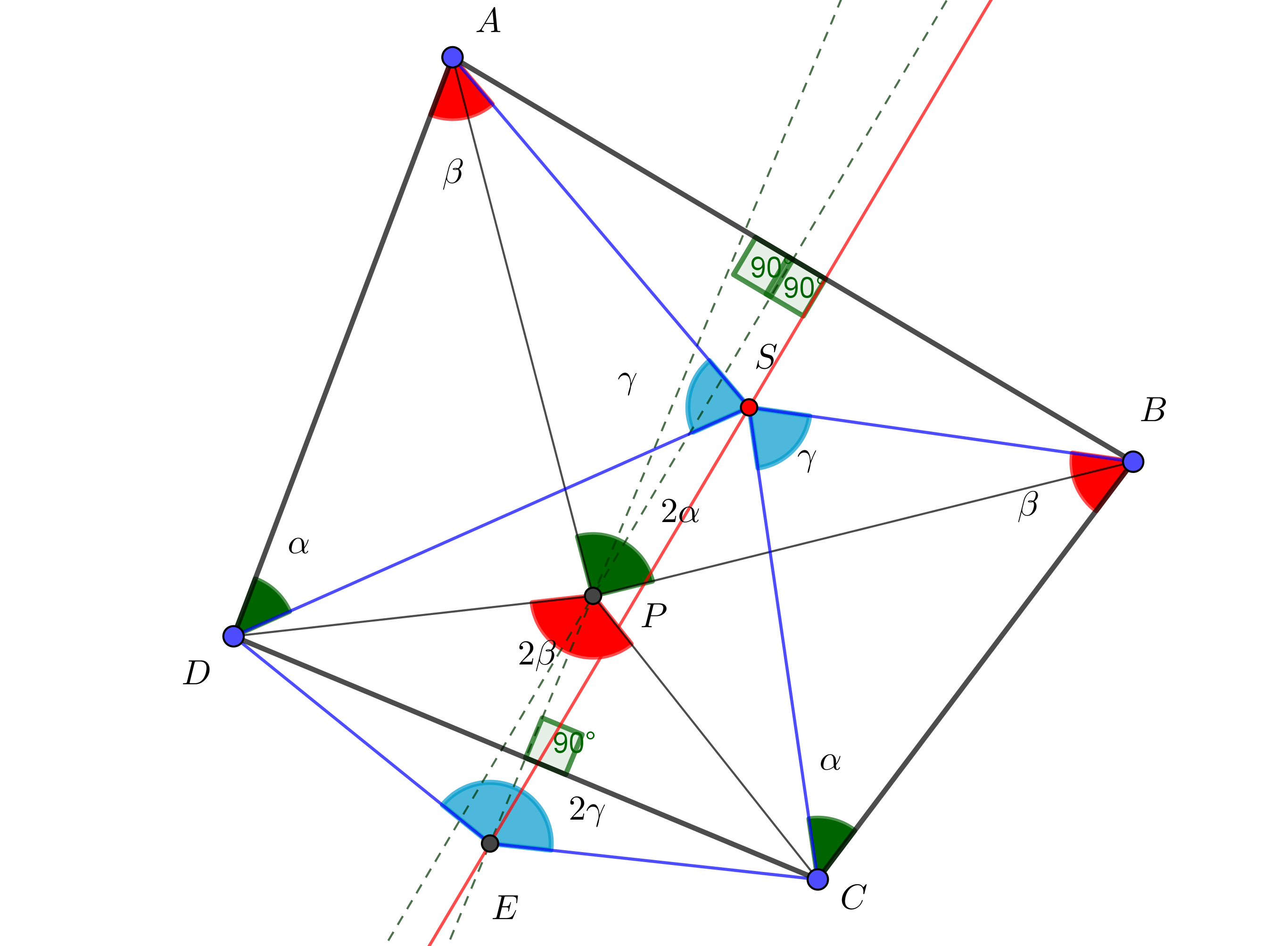
I am looking for a proof of a generalization Napoleon theorem, Bottema theorem and Brahmagupta theorem and van Aubel theorem, and Finsler–Hadwiger theorem in one configuration, as follows:
Let four points $A, B, C, D$ in the plain, the perpendicular bisector of $AB$ meets the perpendicular bisector of $CD$ at $P$. then always exist only one point $S$ such that:
$(\overrightarrow{\rm PD}, \overrightarrow{\rm PC})\equiv 2(AD, AS) \equiv 2(BS, BC)$ and $(\overrightarrow{\rm PB}, \overrightarrow{\rm PA})\equiv 2(CB, CS) \equiv 2(DS, DA)$ (in the figure).
The line through $S$ meets the perpendicular bisector of $CD$ at $E$ then $ES \perp AB$ if only if $(\overrightarrow{\rm EC}, \overrightarrow{\rm ED})\equiv 2(SC, SB) \equiv 2(SA, SD)$
Application:
If the problem was proved. Then we can apply the theorem to proof two famous theorem:
A Proof of the Napoleon theorem: Apply the theorem part one with $\beta=120^\circ$ and $\alpha=30^\circ$ (See Figure 1).
A Proof of the Bottema theorem: Apply the theorem part one with $\beta=45^\circ$ and $\alpha=90^\circ$ (See Figure 1).
A proof of Van Aubel theorem. Apply the theorem part one, with $\alpha=\gamma=45^\circ$
A roof of Finsler–Hadwiger theorem. Apply the theorem part one, with $\alpha=\gamma=45^\circ$
A Proof of the Brahmagupta theorem: Apply the theorem part two with $\gamma=90^\circ$ (See Figure 1).
See also: