If $K$ is a finitely generated field extension of $k$, then there exists an irreducible affine $k$-variety with function field $K$. The idea is that if $x_1, \dots, x_n$ are generators of $K$ under $k$, i.e each elements of $K$ is a rational function in $x_1, \dots , x_n$, then the kernel of the map $k[t_1,\dots, t_n]\to K$ is a prime ideal and the induced map between their field fractions is an isomorphism:
$(k[t_1,\dots, t_n]/I)_0\cong K$
This means $Z(I)\subseteq k^n$ is the affine irreducible variety which field fraction corresponds to $K$.
Now I have the following problem:
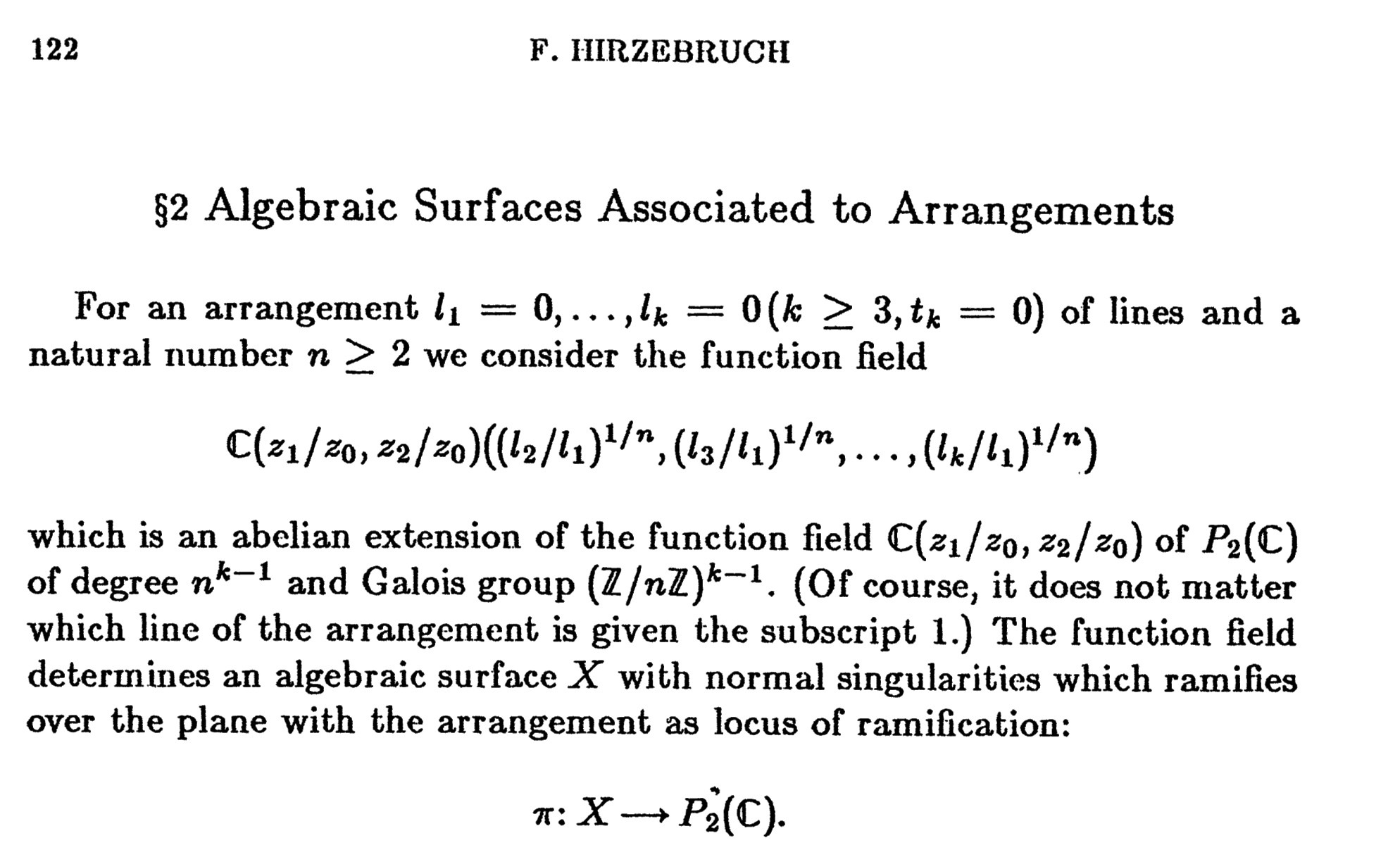
In this case I have $k$ equal to the function field of $\mathbb{P}^2$, and $K$ equal to the finite extension $k((\frac{l_2}{l_1})^{\frac{1}{n}},\dots, , (\frac{l_k}{l_1})^{\frac{1}{n}})$. In the paper the author tells us $K$ determine an algebraic (affine?) surface $X$ with normal singularities and a natural map $\pi: X\to \mathbb{P}^2$.
I don't understand how to define this natural map $\pi$ and what is exactly this surface $X$. I think that $K$ determine an affine variety up to birational morphisms and so I don't understand how to define exactly $X$.
Can you give me an example for $n=2$ and $k=3$, please?