Folding a non-rectangular shape into a rectangle of uniform thickness

Question

I think the following might be an interesting subproblem of this question:

Question: For an odd number $n\ge 3$, is there a non-rectangular but still convex shape of area $A=1$, that can be folded (no tearing) into a rectangle of area $1/n$ of uniform thickness, that is, the resulting rectangle has $n$ layers of paper everywhere?

---

Why these restriction?

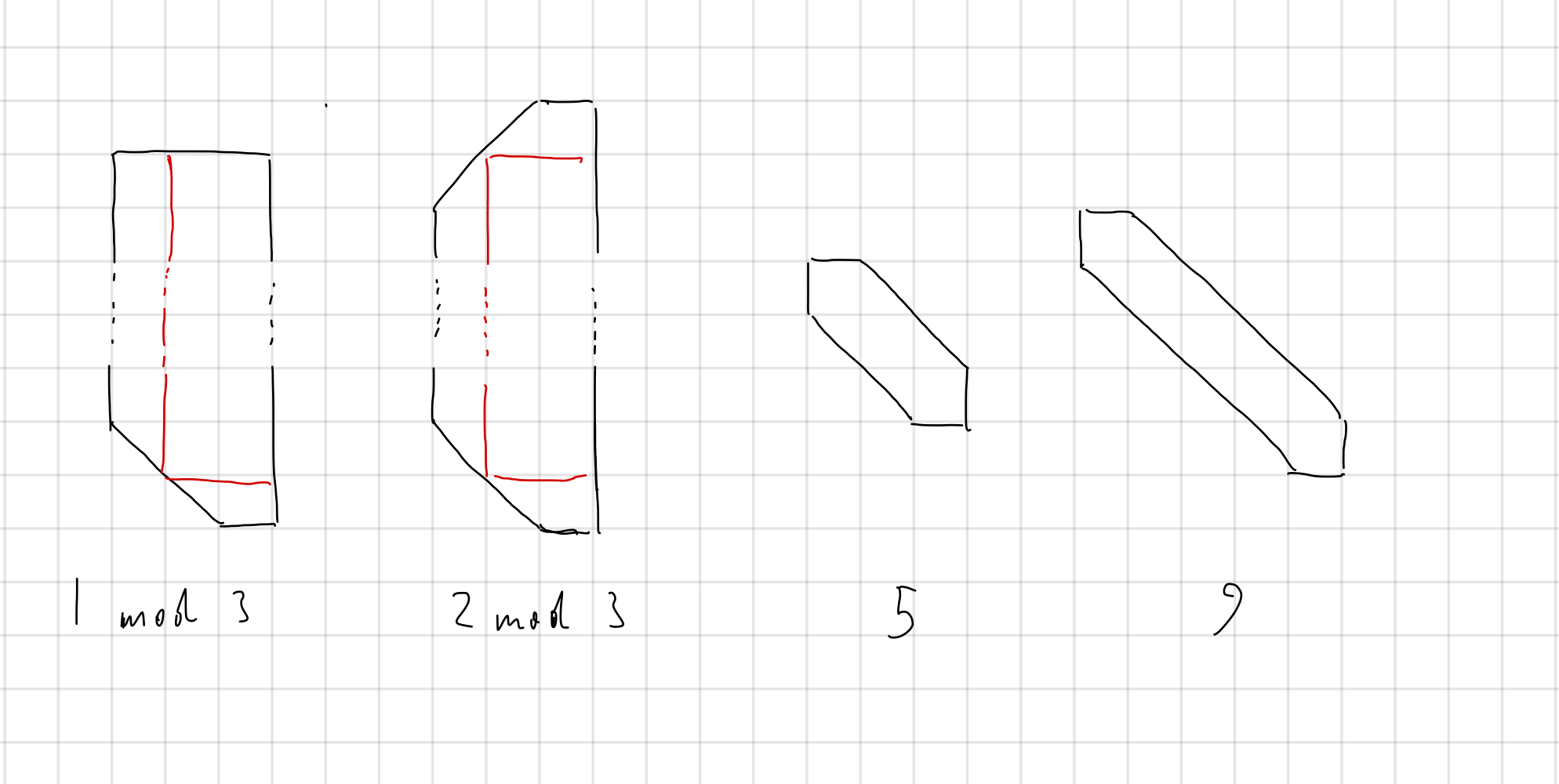
It is easy for every $n$ when dropping convexity (see my answer).

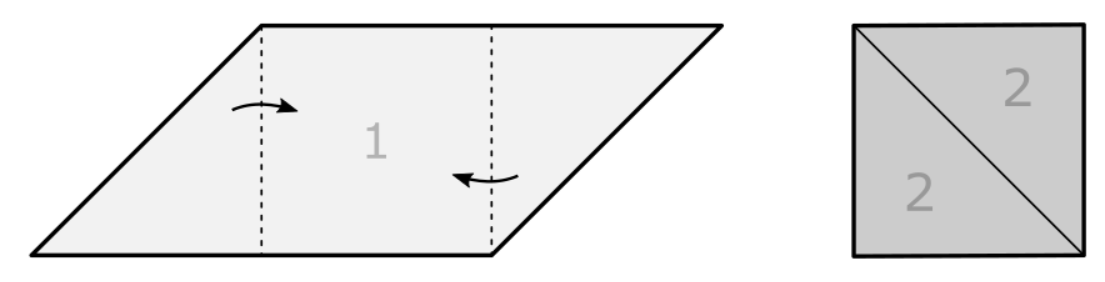
It is also easy for $n=2$ (see the parallelogram below), and once you have a rectangle, you can get any multiple of $n$ too. This is why I ask for odd $n$.

It is also easy if the result does not have to be a rectangle: a circular disc admits an $n$-layer folding for all $n$, and for each fixed $n$ there is a polygonal shape admitting an $n$-layer folding (again, see my answer). Here is a solution for $n=3$:

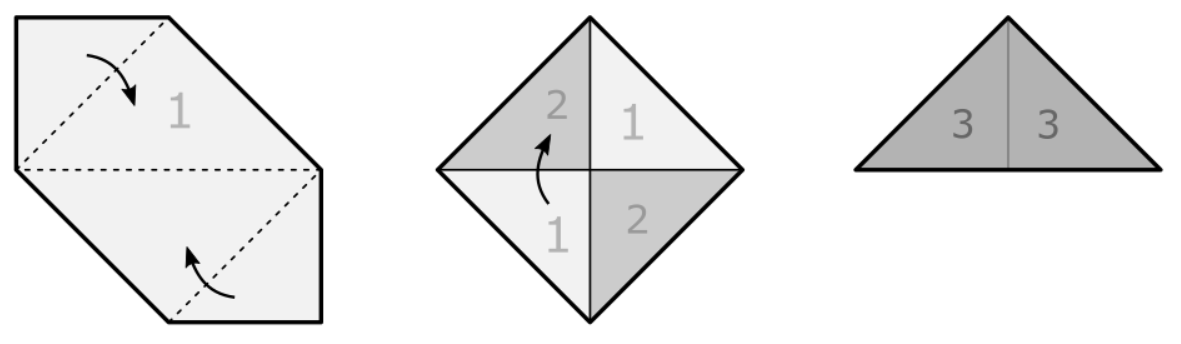
But as far as I can tell, the resulting triangle cannot be made into a 9-layer folding. This would be possible if the result would have been a rectangle.

In particular, I have no solution to my questions for $n=3$, nor an argument why it should not be possible.

Answer 1

I also have no solution for $n=3$, but I have solutions for every $n > 3$. The constructions are sketched below, for each of them we fold along all grid lines; folding along the red lines first has helped me understand that this really gives a uniform cover of the unit square. The number of layers is equal to the area of the polygon.

Constructions for multiples of $3$ can be obtained by further folding the rectangles obtained from the constructions for their other prime divisors or (for powers of $3$) from the construction for $n=9$.