ratio between a polygon bounded in another polygon [closed]

Question

Let A be a convex polygon with area SA. Construct a new polygon B by orderly connecting the midpoints of the segments of A. Denote the area of B by SB. Claim : the ratio SB/SA is constant for all polygons of the same number of vertices. This ratio is easy to calculate for regular polygons. According to the avove claim , it is identical for all polygons with the same number of vertices. For example , for Hexagons , this ratio is 0.75. This claim can be observed empirically by using GEOGEBRA.

Answer 1

All you need to do is note that if you regard the set of vertices as an element of $2n$-dimensional space in the usual way, then the vertex transformation is represented by a simple matrix. Then use the formula $$A=1/2 \sum (x_iy_{i+1}-x_{i+1}y_i)$$ for the area of the polyhedron.

Added: as mentioned below, the statement of the OP is incorrect, but the method suggested here does provide a formula for the required area. It is of the form $A/2$ plus a correcting term which can be interpreted as a sum of areas of associated triangles. Not sure if it is much use though.

Answer 2

This is true only for triangles and quadrilaterals. These shapes have area ratios of 1/4 and 1/2 respectively. It does not hold for polygons with more than 4 sides.

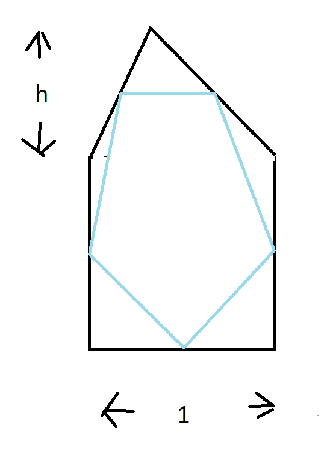
To prove this consider the pentagon consisting of a unit square with a triangle of height $h$ placed on top:

Then the area of the midpoint polygon is $5/8+3h/8$ and the original polygon, $1+h/2$. Therefore the ratio is $\frac{3h+5}{4h+8}$ which is clearly not constant as we vary $h$. Similar arguments show that the ratio is non-constant for more vertices.

For example by adding further vertices onto the bottom side of the pentagon, keeping it a single line, we keep the area of the polygon the same and increase the midpoint area by a fixed amount independent of $h$. This produces a ratio of the form $\frac{3h+a}{4h+b}$ for some $a$ and $b$ which again is non-constant for varying $h$.