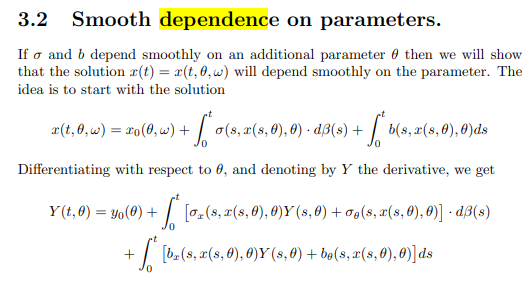
Let
- $b,\sigma:\mathbb R\to\mathbb R$ be differentiable and Lipschitz continuous
- $(\Omega,\mathcal A,\operatorname P)$ be a probability space
- $(\mathcal F_t)_{t\ge0}$ be a complete and right-continuous filtration on $(\Omega,\mathcal A,\operatorname P)$
- $(W_t)_{t\ge0}$ be an $\mathcal F$-Brownian motion on $(\Omega,\mathcal A,\operatorname P)$
- $(X^x_t)_{(t,\:x)\in[0,\:\infty)\times\mathbb R}$ be a real-valued continuous $\mathcal F$-adapted process on $(\Omega,\mathcal A,\operatorname P)$ with $$X^x_t=x+\int_0^tb(X^x_s)\:{\rm d}s+\int_0^t\sigma(X^x_s)\:{\rm d}W_s\tag1$$ for all $t\ge0$ almost surely for all $x\in E$
Are we able to show that $\mathbb R\ni x\mapsto X^x_t(\omega)$ is differentiable for all $(\omega,t)\in(\Omega\setminus N)\times[0,\infty)$ for some $\operatorname P$-null set $N$?
Note that the hypotheses are sufficient to guarantee the existence of the process $X$ above. However, I'm not sure if they are sufficient to guarantee the existence of a real-valued continuous $\mathcal F$-adapted process $(Y^x_t)_{(t,\:x)\in[0,\:\infty)\times\mathbb R}$ with $$Y^x_t=\int_0^tb'(X^x_s)Y^x_s\:{\rm d}s+\int_0^t\sigma'(X^x_s)Y^x_s\:{\rm d}W_s\tag2$$ for all $t\ge0$ almost surely for all $x\in\mathbb R$ as well. However, if this process $Y$ exists, then it's clearly a candidate for the desired process obtained by differentiating $X$ with respect to the spatial parameter.
Maybe it's useful to remember how the existence of $X$ is proved: For fixed $T>0$ and $\xi\in L^2(\operatorname P)$, we know that the map $$\Xi_T(Z):=\xi+\left(\int_0^tb(s,Z_s)\:{\rm d}s\right)_{t\in[0,\:T]}+\left(\int_0^t\sigma(s,Z_s)\:{\rm d}W_s\right)_{t\in[0,\:T]}\in\mathcal B_T$$ for $Z\in\mathcal B_T$, where $$\mathcal C_T:=\left\{Z:Z\text{ is a continuous }(\mathcal F_t)_{t\in[0,\:T]}\text{-adapted process on }(\Omega,\mathcal A,\operatorname P)\right\}$$ is equipped with $$\left\|Z\right\|_T:=\left\|\sup_{t\in[0,\:T]}Z_t\right\|_{L^2(\operatorname P)}\;\;\;\text{for }Z\in\mathcal C_T$$ and $$\mathcal B_T:=\left\{Z\in\mathcal C_T:\left\|Z\right\|_T<\infty\right\},$$ has a unique fixed point and there is a constant $c\ge0$, depending only on $b$ and $\sigma$, with $$\left\|\Xi_T(Z)\right\|_T^2\le3\left(\operatorname E\left[|\xi|^2\right]+c(T+4)\left(T+\int_0^T\left\|Z\right\|_t^2\:{\rm d}t\right)\right)\tag3$$ for all $Z\in\mathcal B_T$.
Maybe this can be used to show that $$\left\|\frac{X^{x+h}-X^x}h-Y^x\right\|_T\le c_1|h|\;\;\;\text{for all }T>0\text{ and }h\in\mathbb R\tag4$$ for some $c_1\ge0$, depending only on $b$ and $\sigma$, which should even allow for a stronger conclusion then the one asked for in the question.