If a morphism of topological spaces $X\rightarrow Y$ is a fibration, and the target space is connected, then the fibers of the points $y\in Y$ are homotopy equivalent, i.e. for all $y_1,y_2\in Y$ we have $X_{y_1}\cong X_{y_2}$. My question is whether or not this is a sufficent condition for this map to be a fibration or what the proper counter-example is to keep in mind.
$\begingroup$
$\endgroup$
2
-
6$\begingroup$ An important weaker notion is that of a quasi-fibration: a map $f: X \to Y$ for which the canonical map $f^{-1}(x) \to \text{hofib}(f;x)$ is a homotopy equivalence. In particular, all fibers are homotopy equivalent. The usual example of a quasifibration which is not a fibration is the map from the letter T to the unit interval I which collapses the top bar to a point. $\endgroup$– mmeCommented May 3, 2020 at 22:15
-
1$\begingroup$ The identity map $i:X_{dis}\rightarrow X_{ind}$ from the discrete topology on a set $X$ to the indiscrete topology on the same set. Assume the topologies don't coincide. The fibres are all points. $i$ is not a Serre fibration since it doesn't have the HLP with respect to $I^0=\ast$. Hence it is not a Hurewicz fibration (more directly: one such would necessarily be a quotient). $i$ is not a quasifibration since the homotopy fibre is $X_{dis}$ and is not contractible. It is not a Dold fibration since it is not fibre homotopy equivalent to the identity on $X_{ind}$ (use numerable contractibility) $\endgroup$– TyroneCommented May 4, 2020 at 10:21
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
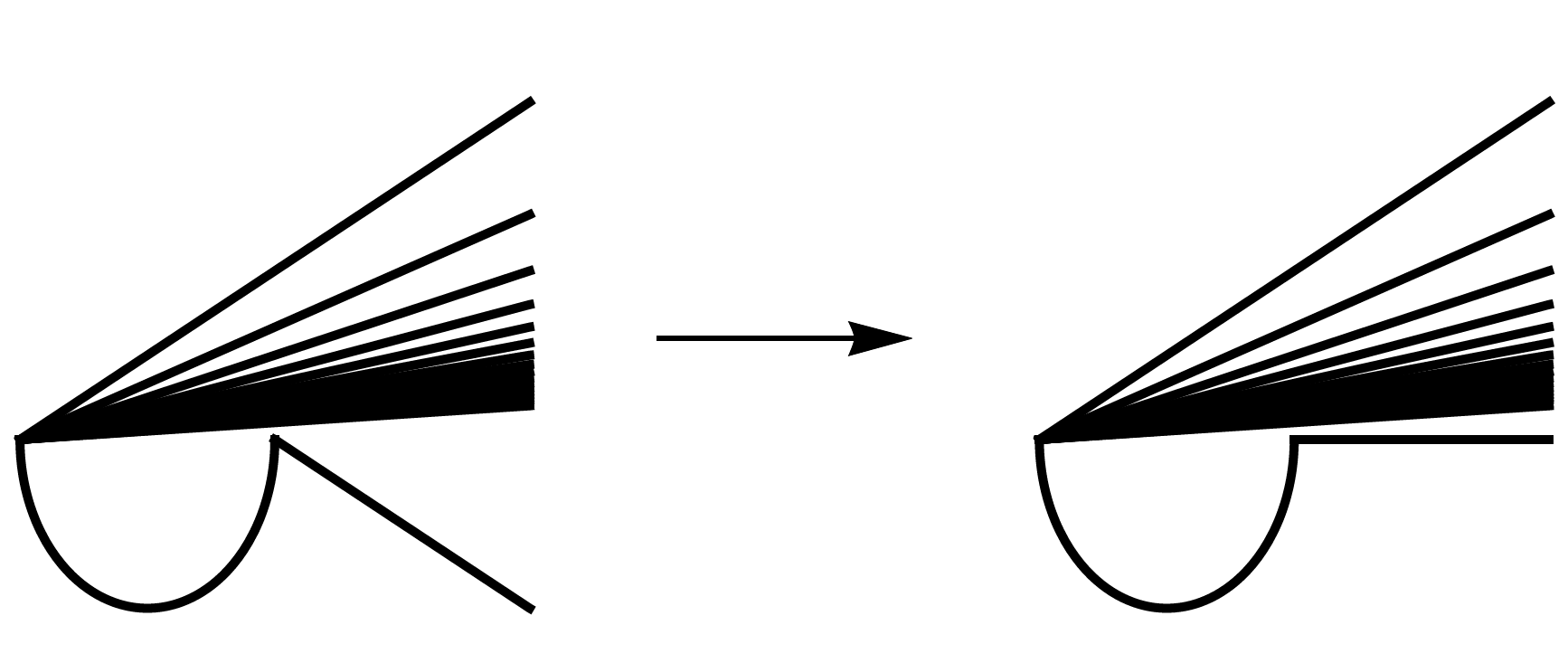
The figure below gives a simple but extreme counterexample, which I think has all the lifting properties one might want except for actually being a true fibration. The map is the identity everywhere except for the linear segment with negative slope, which is mapped to the horizontal segment in the codomain. This map is a continuous bijection so all of the fibers are homeomorphic. It is even a Serre fibration. However, it does not have the homotopy lifting property with respect to the convergent sequence space $Z=\{1,1/2,1/3,\dots ,0\}$, which appears as the middle cross section of both spaces.
answered May 3, 2020 at 23:36