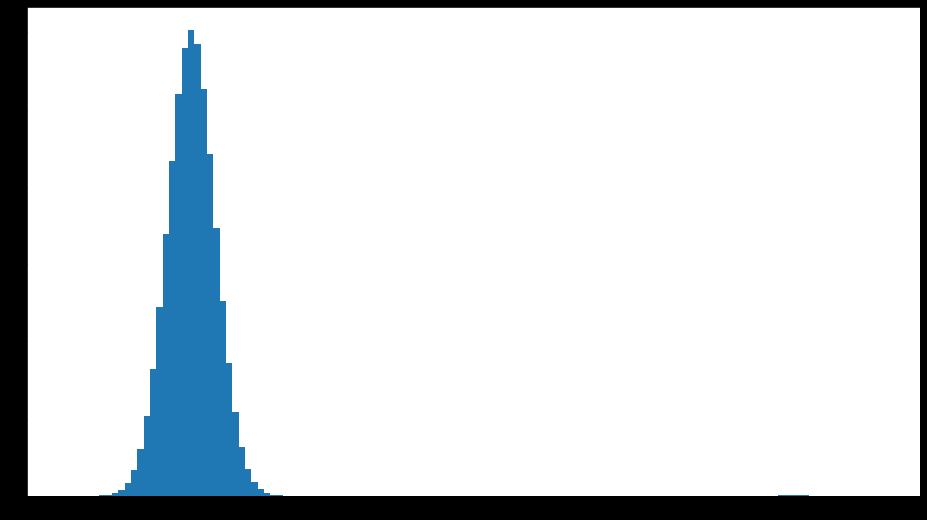
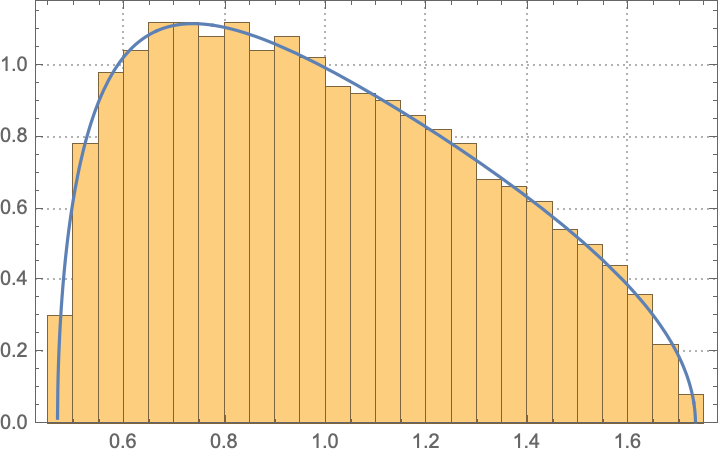
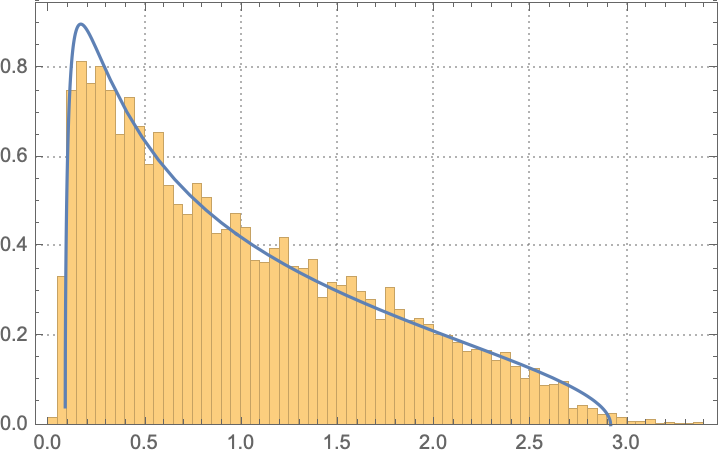
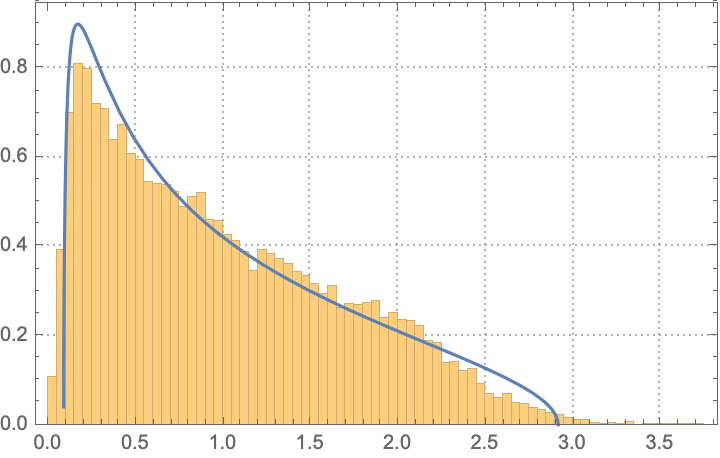
Suppose $X \in \mathbb{R}^{N \times M}$ with elements sampled i.i.d. from $\mathcal{U}(-\sigma, \sigma)$.
I would like to find the marginal distribution of the unordered singular values of $X$. The case for $X \sim \mathcal{N}(0, \sigma)$ has been extensively studied, but i can't seem to find any work on the uniform distribution case.
Or equivalently the distribution of the eigenvalues of $X^TX$. The distribution of the $X^TX$ seems to follow some gaussian with mean zero and a small gaussian in the tail as observed in some simulations. This leads to the eigenvalues not being distributed as gaussian i.i.d.