2
$\begingroup$

I asked this two weeks ago at https://math.stackexchange.com/questions/3571697/squares-in-a-second-order-integer-recursive-sequence

Most of the related question shown to me while composing are about primes, not squares...

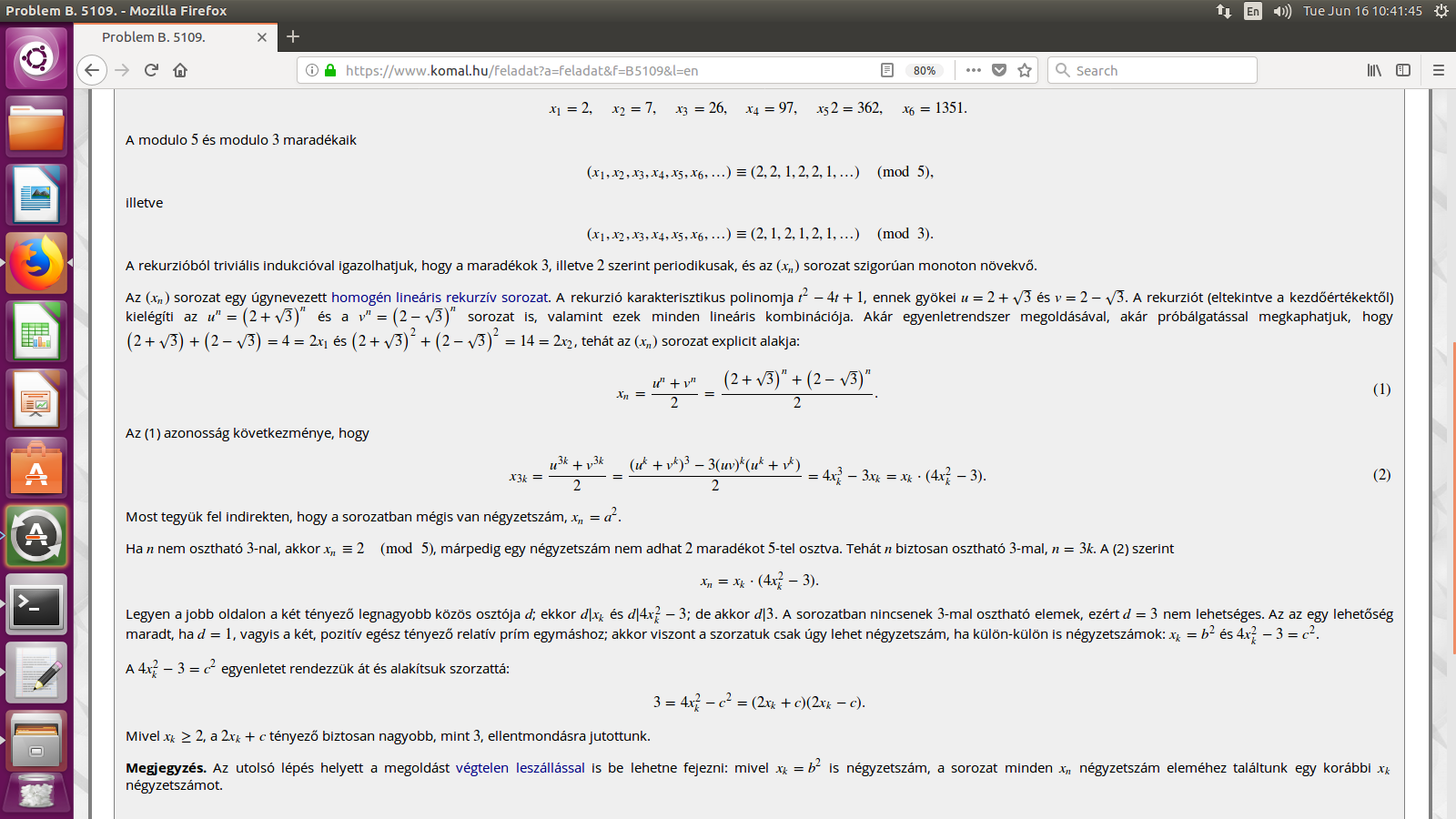
Given a sequence $x_n$ as in https://oeis.org/A001075 $$ 1, 2, 7, 26, 97, 362, 1351, $$ such that $$ x_{n+2} = 4 x_{n+1} - x_n $$

These are the $x$ values in $x^2-3y^2 = 1$

Can we find, and prove, all squares in the sequence and all double squares? I see that Cohn did this for the Fibonacci and Lucas numbers in the 1960's. For this sequence, it seems $1$ is the only square and $2$ is the only doubled square.

alright, an answer at the recent Squares in Lucas sequences does give a reference Petho

Improve this question
$\endgroup$
1
  • $\begingroup$ Is this sequence somehow special? (and why?) $\endgroup$
    – Wlod AA
    Commented Mar 24, 2020 at 9:38

1 Answer 1

Reset to default
2
$\begingroup$

CW. this turns out to be a question from May 2020 in a Hungarian magazine for high school students; a bit deflating. The deadline was June 10th and an answer became available online; they did squares but did not bother with twice squares.

enter image description here

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .