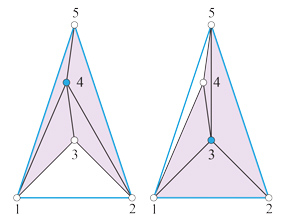
Given a set of $n$ points in the Euclidean plane of which no three are collinear, does there always exist a convex triangulation and how can one be found algorithmically?
In this context a convex triangulation shall mean a triangulation in which the union of triangles with a common corner-point inside the convex hull constitute to a convex polygon.