7
$\begingroup$

Restriction of Scalars and Functoriality of Presheaves.

Let $\phi\colon R\longrightarrow S$ be a morphism of rings. There is associated to $\phi$ a natural functor from $\mathrm{Alg}_S$ to $\mathrm{Alg}_R$, called the restriction of scalars functor: $$f\colon\mathrm{Alg}_S\longrightarrow\mathrm{Alg}_R.$$ In detail, this is the functor taking an $S$-algebra $S\rightarrow A$ to the $R$-algebra $R\rightarrow S\rightarrow A$, which we denote $A_R$.

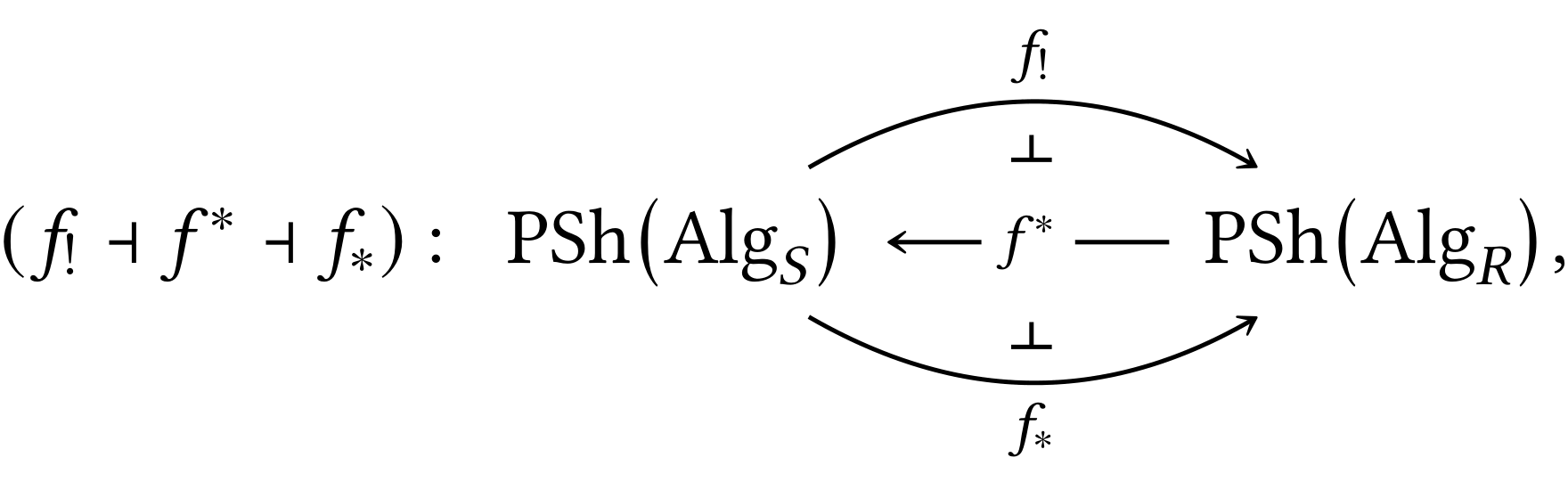
As remarked in this nLab page (and developed in detail in SGA IV, Exposé I, Section 5), there exists an induced adjoint triple of functors between the corresponding presheaf categories:

adjunction

where $f^*\colon\mathrm{PSh}(\mathrm{Alg}_R)\longrightarrow\mathrm{PSh}(\mathrm{Alg}_S)$ is given by precomposition with $f$.

Base Change of Schemes.

Consider the restriction $f^*|_{\mathrm{Aff}_R}$ of $f^*$ to the full subcategory $\mathrm{Aff}_R$ of $\mathrm{PSh}(\mathrm{Alg}_R)$ spanned by the representable presheaves on $\mathrm{Alg}_R$, i.e. by affine $R$-schemes.

This functor takes an $R$-scheme $h_A$ to the presheaf $$f^*h_A\colon\mathrm{Alg}_S\longrightarrow\mathrm{Alg}_R\longrightarrow\mathrm{Sets}$$ defined by $$B\mapsto\mathrm{Hom}_{\mathrm{Alg}_R}(B_R,A)\cong\mathrm{Hom}_{\mathrm{Alg_S}}(B,A\otimes_RS),$$ where the isomorphism comes from the adjunction between restriction and extension of scalars.

That is, $f^*h_A=h_A\times_R h_S$ and the functor $f^*$ is therefore base change of schemes.

Adjoints to Base Change.

As R. van Dobben de Bruyn points in the comments, the right adjoint $f_*$ of $f^*$ is called Weil restriction. While it can fail to be a scheme in general, it is representable by schemes under nice conditions. One may then ask about the left adjoint $f_!$:

Question 1. Is the left adjoint $f_!$ of $f^*$ representable by schemes? Moreover, if it isn't, are there conditions we can require of an $S$-scheme $X$ guaranteeing the presheaf $f_!X$ to be a scheme?

‎

Question 2. What about the non-affine case?

(I gather from the Wikipedia page on Weil restriction that these have been studied in the very general case of schemes over ringed topoi. What reference/s is Wikipedia alluding to?)

Improve this question
$\endgroup$
5
  • $\begingroup$ Could someone please embed the linked image into this question? (MO unfortunately prevents me from doing it myself) $\endgroup$
    – Emily
    Commented Feb 10, 2020 at 4:58
  • 2
    $\begingroup$ For $f_*$ this is studied under the name Weil restriction. It always exists for a finite flat ring extension, but in general is not representable (I don't recall the most general statement right now). This is also related to the more general construction of the Hom scheme $\mathbf{Hom}_S(X,Y)$, which is representable for a pair of proper flat morphisms $X, Y \to S$, but not in general. $\endgroup$
    – R. van Dobben de Bruyn
    Commented Feb 10, 2020 at 8:25
  • $\begingroup$ @R.vanDobbendeBruyn Thanks! I have rewritten the question, both correcting some mistakes I made and making modifications which take into account your comment. $\endgroup$
    – Emily
    Commented Feb 10, 2020 at 21:10
  • 2
    $\begingroup$ Isn't $f_!$ just given by postcomposing the structure morphism with $f$? $\endgroup$
    – Denis Nardin
    Commented Feb 10, 2020 at 21:15
  • 1
    $\begingroup$ @DenisNardin You're right. Combined with R. van Dobben de Bruyn's comment, this settles the question. Thanks! (And sorry for taking so long to reply!) $\endgroup$
    – Emily
    Commented Feb 23, 2020 at 19:31

0

Reset to default

You must log in to answer this question.

Browse other questions tagged .