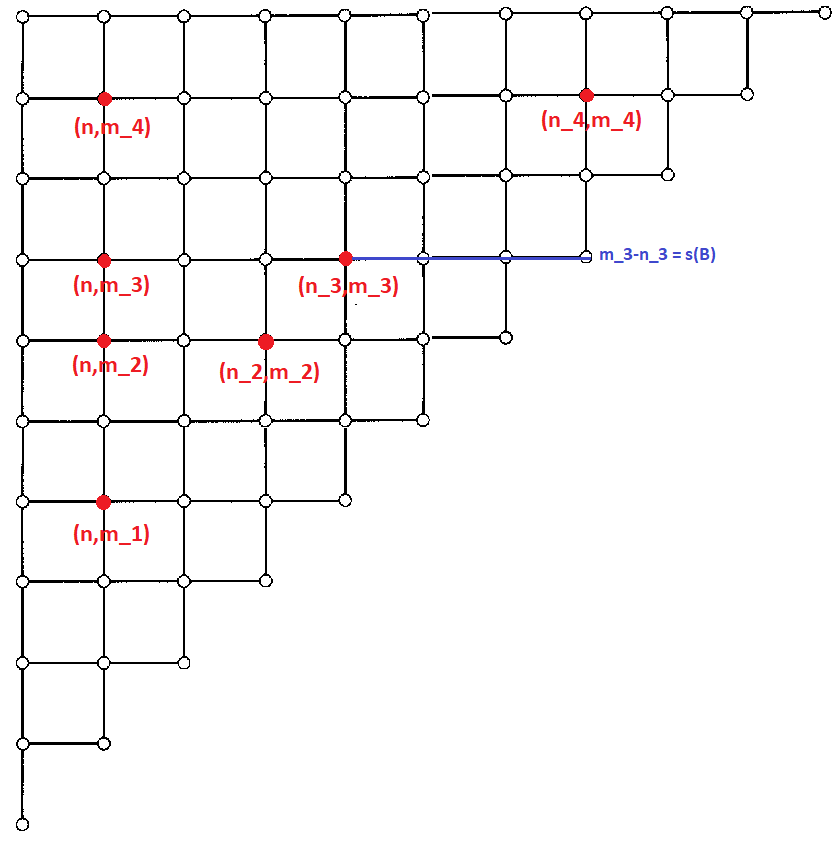
Let $$ \Lambda = \{(x,y)\in\mathbb{N}^2:y\geq x\} $$ the upper triangular lattice and $d:\Lambda\to\{1,\dots,c\}$ a coloring (i.e. an arbitrary function) on $c$ colors. Let $k\geq2$. I am looking for $n\in \mathbb{N}$, $m_1<m_2<\dots m_k$ and $n_j \in [n,m_j]\cap\mathbb{N}$, $j=2,\dots,k$, such that the set $$ B = \{(n,m_1),(n,m_2),\dots,(n,m_k), (n_2,m_2),(n_3,m_3),\dots,(n_k,m_k)\}$$ is monochromatic (i.e. all its points have the same color). See the picture below for an example.
From the ergodic proof of multidimensional Van der Waerden theorem it is easy to show that such set $B$ always exists; moreover, one also has $$ s(B) := \max_{1\leq j\leq k}m_j-n_j \leq m_1$$ My question is: can I take $B$ such that $s(B)$ is bounded by something depending only on the number of colors $c$?