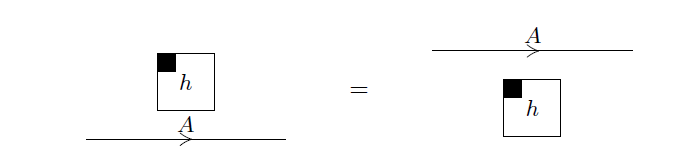An example I learned about in Khovanov's "Heisenberg algebra and a graphical calculus", 2010, is the restriction and induction functors for the infinite chain $S_0\subset S_1\subset S_2\subset\cdots$ of symmetric groups. This is also explained in Likeng and Savage, "Embedding Deligne's category $\operatorname{Rep}(S_t)$ in the Heisenberg category," 2019.
Let $\mathcal{S}=\prod_{m\in\mathbb{N}}\bigoplus_{n\in\mathbb{N}}\mathrm{Bim}{(S_n,S_m)}$, where each $\mathrm{Bim}(S_n,S_m)$ is the category of $(S_n,S_m)$-modules over a field $k$. This has a monoidal structure given by tensor products of compatible bimodules, with the monoidal unit being $I=\prod_{m}k[S_m]$ with each $k[S_m]$ as an $(S_m,S_m)$-module. We may regard $(S_n,S_m)$-modules as objects of $\mathcal{S}$ by setting all non-$m$ indices to the $0$ bimodule.
The induction functor $\mathrm{Ind}_{S_n}^{S_{n+1}}$ can be given as an object $$\mathrm{Ind}=\prod_{n\geq 1} {}_n(k[S_n])_{n-1},$$ where the subscript notation means $k[S_n]$ is treated as an $(S_n,S_{n-1})$-module. This gives induction in the sense that, for each $S_n$-module $M$, the object $\mathrm{Ind}\otimes M$ is $\operatorname{Ind}_{S_n}^{S_{n+1}}M$. Similarly, restriction is given by $$\mathrm{Res}=\prod_{n\geq 1}{}_{n-1}(k[S_n])_n.$$
Just as induction and restriction are biadjoint functors, the $\mathrm{Ind}$ and $\mathrm{Res}$ objects are left and right duals to each other. Two of the four associated pairings and copairings are $\mathrm{Ind}\otimes\mathrm{Res} \to I$ and $I\to \mathrm{Ind}\otimes\mathrm{Res}$.
Since $$\mathrm{Ind}\otimes\mathrm{Res} =\prod_{n\geq 1} {}_{n}(k[S_n]\otimes_{S_{n-1}}k[S_n])_n,$$
we may give their definitions in the form
\begin{align*}
\mathrm{Ind}\otimes\mathrm{Res} &\to I\\
(g\otimes h&\mapsto gh)_{n\geq 1}\\
\end{align*}
and
\begin{align*}
I &\to \mathrm{Ind}\otimes\mathrm{Res}\\
(g& \mapsto \sum_{i=1}^n gg_i\otimes g_i^{-1})_{n\geq 1},
\end{align*}
where $g_1,\dots,g_n\in S_n$ form a set of coset representatives for $S_n/S_{n-1}$.
The composition $h:I\to \mathrm{Ind}\otimes\mathrm{Res} \to I$ of these is $h=(n\operatorname{id})_{n\geq 1}$.
We can calculate
\begin{align*}
\operatorname{id}_{\mathrm{Ind}}\otimes h &= ((n-1)\operatorname{id})_{n\geq 1}\\
h\otimes \operatorname{id}_{\mathrm{Ind}} &= (n\operatorname{id})_{n\geq 1},
\end{align*}
and therefore $\mathcal{S}$ is not spacial.
Graphically, this is that counter-clockwise loops cannot be dragged across an upward strand, imagining $\mathrm{Ind}$ as an up-arrow and $\mathrm{Res}$ as a down arrow.