Polynomials that share at least one root

Question

This is a generalization of an MSE question, Polynomials that share at least one root.

Let $P(x)$ be a specific polynomial of degree $d$, with given real coefficients $A_i$ ($A_d=1$), and real roots: $$P(x) = x^d + A_{d-1}x^{d-1} + A_{d-2}x^{d-2} + \cdots + A_{0}\;. $$

Q. What does the set of polynomials, with real coefficients $a_i$, $$p(x) = x^d + a_{d-1}x^{d-1} + a_{d-2}x^{d-2} + \cdots + a_{0} \;,$$ look like (geometrically) in $\mathbb{R}^d$, when each $p(x)$ shares at least one root with $P(x)$?

I am seeking a description of this set in the space of the $d$ coefficients, $(a_0,\ldots,a_{d-1})$. The reason there is hope for a nice description, is that it makes a pretty picture for $d=2$.

Let $P(x) = x^2 + A_1x + A_0$, with $A_1=3$ and $A_0=-1$. The plot in the $a_0 a_1$-plane of all other $p(x)= x^2 + a_1x + a_0$ that share a root with $P(x)$, looks like this:

---

         
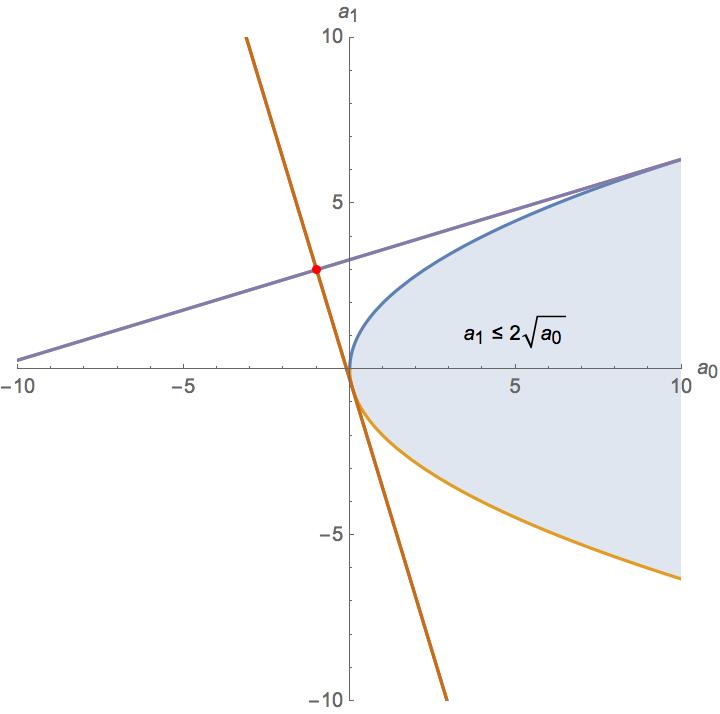
          ^{Lines intersect at $(a_0,a_1)=(-1,3)$. The discriminant is $a_1^2 = 4 a_0$.}

---

All those $(a_0,a_1)$ on the two lines share a root with $x^2 + 3x -1$. The lines are tangent to the discriminant parabola.

Answer 1

The locus of real polynomials $p(x)$ sharing a root with $P(x)$ is the union of the hyperplanes $H_\alpha : p(\alpha)=0$, where $\alpha$ runs over the roots of $P(x)$. This is an arrangement of hyperplanes.

The intersection of $H_\alpha$ with the discriminant variety $D : \mathrm{disc}(p)=0$ contains the subspace $H^{(1)}_{\alpha} : p(\alpha)=p'(\alpha)=0$. In fact $H_\alpha$ and $D$ are tangent along $H^{(1)}_\alpha$. To see this, take a generic polynomial $p_0(x)=(x-\alpha)^2 q_0(x)$ in $H^{(1)}_\alpha$. Near $p_0$, polynomials in $D$ will be of the form $p(x)=(x-\alpha+\varepsilon)^2 q(x)$ where $q$ is near $q_0$. Then $p(\alpha)=\varepsilon^2 q(\alpha)$, while the distance from $p_0$ to $p$ is of first order with respect to $\varepsilon$.

Answer 2

I tried to make a 3D image for $P(x)=x^3+3 x^2-2 x-1$. The set consists of three planes, each tangent to the discriminant surface. But it became too visually complex, partly because the discriminant is complicated. For what it's worth:

---

         
          ^{Discriminant surface: blue.}

---

Perhaps someone can do better...