I asked this a week ago at math.stackexchange, without success, so I hope it will be appropriate here.
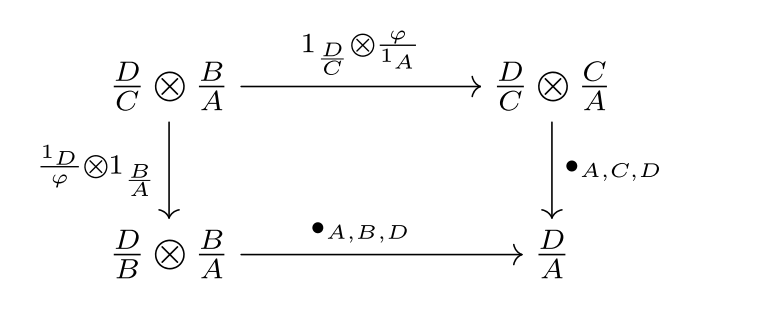
Let ${\mathcal C}$ be a symmetric closed monoidal category, and let me denote the internal hom-functor by a fraction $$ (X,Y)\mapsto\frac{Y}{X}, $$ so that we have an isomorphism of functors $$ \operatorname{Mor}(A\otimes B,C)\cong \operatorname{Mor}\left(A,\frac{C}{B}\right). $$ As is known, ${\mathcal C}$ is an enriched category over itself. For each objects $A,B,C$ let me denote by $\bullet_{A,B,C}$ the "inner composition" in ${\mathcal C}$ as in an enriched category, i.e. the morphism $$ \bullet_{A,B,C}:\frac{C}{B}\otimes\frac{B}{A}\to\frac{C}{A} $$ with the corresponding properties.
I wonder if the following identity always holds $$ \bullet_{A,C,D}\circ\left(1_{\frac{D}{C}}\otimes\frac{\varphi}{1_A}\right)= \bullet_{A,B,D}\circ\left(\frac{1_D}{\varphi}\otimes1_{\frac{B}{A}}\right) $$ (for arbitrary objects $A,B,C,D$ and for arbitrary morphism $\varphi:B\to C$).
This is strange, I can prove this only in the case when the unit $I$ is a separating object in ${\mathcal C}$ (what does not always hold). Is it possible that there is a counterexample?