6
$\begingroup$

I am a bit confused about the statement of Theorem 1.1 in this paper by Brian White. For convenience, I will restate it here.

Theorem: Let $\Omega$ be an open subset of a Riemannian $3$-manifold. Let $g_i$ be a sequence of smooth Riemannian metrics on $\Omega$ converging smoothly to a Riemannian metric $g$. Let $M_i \subseteq \Omega$ be a sequence of properly embedded surfaces such that $M_i$ is minimal with respect to $g_i$. Suppose also that the area and the genus of $M_i$ are uniformly bounded on compact subsets of $\Omega$. Then (after passing to a subsequence) the $M_i$ converge to a smooth, properly embedded $g$-minimal surface $M$. For each connected component $\Sigma$ of $M$, either

  1. the convergence to $\Sigma$ is smooth with multiplicity one, or
  2. the convergence is smooth (with some multiplicity $> 1$) away from a discrete set $S$.

In the second case, if $\Sigma$ is two-sided, then it must be stable.

My question concerns the last sentence of the statement above. It seems very surprising to me the conclusion about the stability. What if $g$ is a metric with strictly positive Ricci curvature?

I was thinking about the following 2-dimensional situation. Imagine to have a sequence of metrics $g_i$ on the 2-sphere such that the limit metric $g$ has positive curvature. Imagine that each $g_i$ carries two closed geodesics which are converging to an unstable geodesic with multiplicity 2. See this poor quality picture: 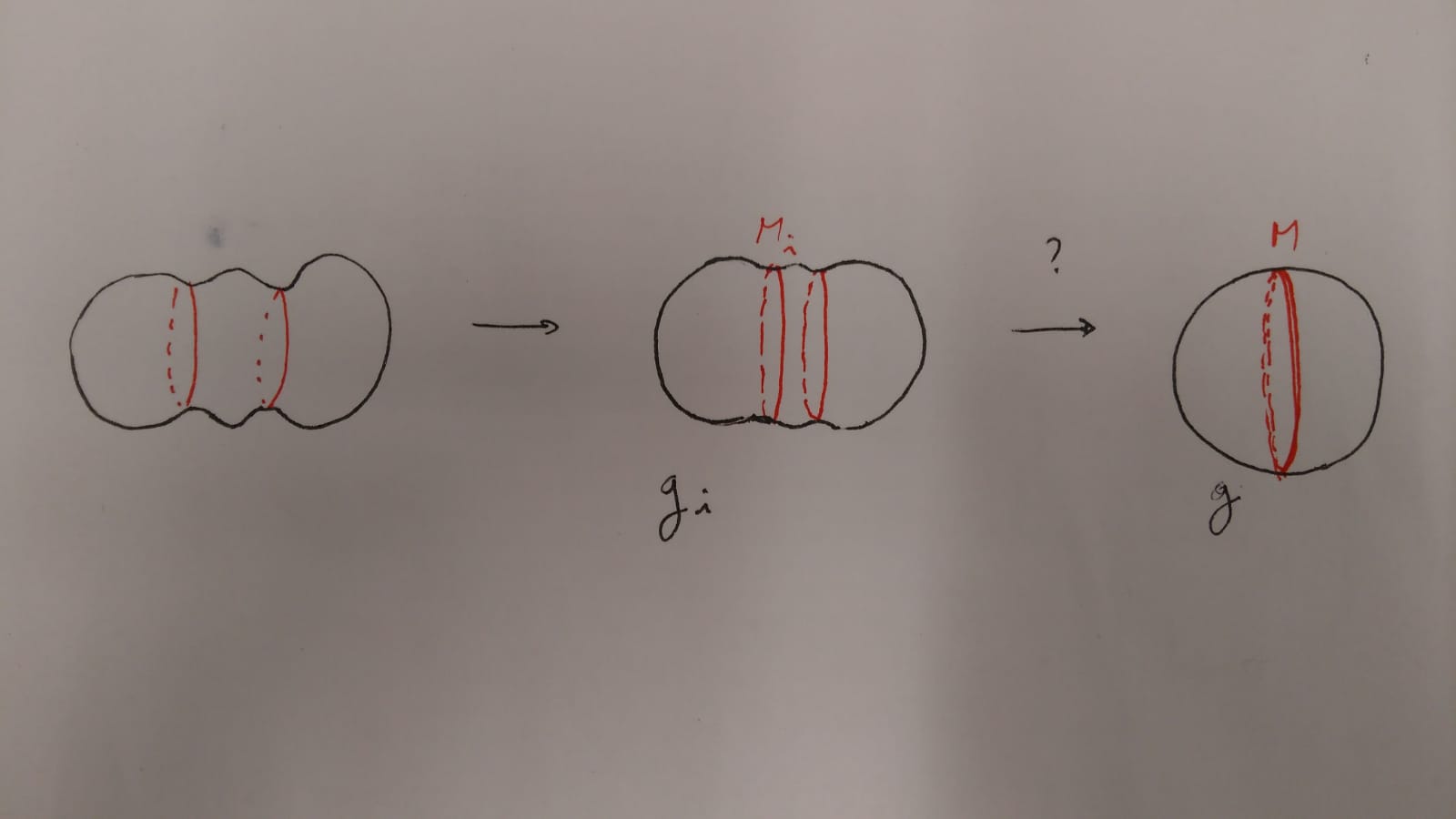enter image description here

The compactness theorem above says that one cannot construct such an example on the $3$ sphere and using minimal surfaces instead of geodesics. Can anyone give me an intuition of what is going on here?

Thank you!

Improve this question
$\endgroup$

1 Answer 1

Reset to default
6
$\begingroup$

What's going on with the picture you drew is that because the $g_i$ have regions of negative curvature (in the "valleys" where the $M_i$ sit) that become arbitrarily close to the "hilltop" where $M$ sits), one must have that the curvature of $g$ along $M$ is identically zero. This means that $M$ is actually weakly stable as the constant function $1$ is an eigenfunction of the stability operator (and is the lowest as it doesn't change sign) with eigenvalue $0$.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thank you for your answer! So essentially you are saying that the limit metric $g$ in my example cannot actually be the standard metric of $\mathbb{S}^2$? $\endgroup$
    – Onil90
    Commented May 1, 2019 at 14:31
  • 2
    $\begingroup$ @Onil90 Yes. Think of $f_\epsilon(x)=x^4-2 \epsilon^2x^2$. This has strict local minima at $x=\pm \epsilon$ and a strict local maxima at $x=0$ when $\epsilon>0$. However, $f_0=x^4$ has only a degenerate local minima at $x=0$. $\endgroup$
    – RBega2
    Commented May 1, 2019 at 17:15
  • $\begingroup$ Oh, right! Thanks a lot for the clarification!! $\endgroup$
    – Onil90
    Commented May 1, 2019 at 19:53

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .