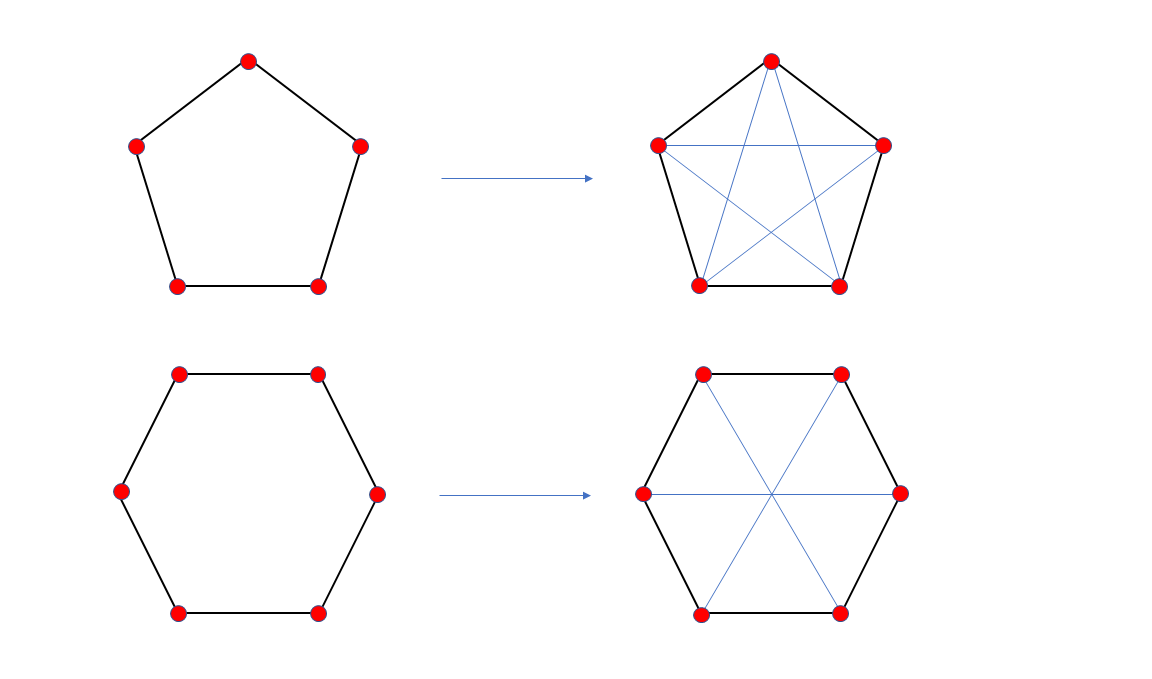
A 2-connected $3$-connected graph $G$ is "Almost Planar" Locally Nonplanar if it has a a $2$-connected spanning subgraph $H$ and an embedding in the plane such that $H$ is planar in this embedding and all the crossing edges are cords of faces of $H$ (all crossings and crossing edges are contained inside the faces of $H$.)
Is there any literature on this class of graphs? Are they classified by any other name? What kind of graphs are in this class? Are there graphs which are not Almost Planar?
Any information on this will be appreciated.