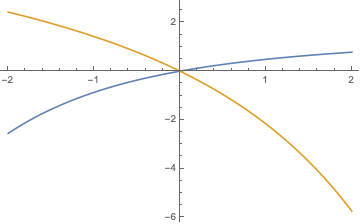Is there a closed-form expression for this series?
$\displaystyle\sum_{k\geq 1}^\infty \frac{\lambda^k e^{-\lambda}}{k!}\cdot[1-(1-x)^k]\cdot \frac{1}{k}$
Any answers, ideas or references would be appreciated.
$\begingroup$
$\endgroup$
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
3
No closed form expression in terms of elementary functions, but if you are satisfied with special functions (incomplete Gamma function $\Gamma$ and exponential integral Ei), then $$\displaystyle\sum_{k=1}^\infty \frac{\lambda^k e^{-\lambda}}{k!}\cdot[1-(1-x)^k]\cdot \frac{1}{k}=$$ $$=e^{-\lambda}\,\Re \bigg(\text{Ei}\left(\lambda\right)+\ln (x-1)+\Gamma \left[0,\lambda (x-1)\right]\biggr),\;\;\lambda,x\in\mathbb{R}.$$ ($\Re$ indicates the real part.) Here is a plot of the $x$-dependence for $\lambda=+1$ (blue) and $\lambda=-1$ (gold).
answered Jan 24, 2019 at 18:32
-
$\begingroup$ Is "No closed form expression in terms of elementary functions" a theorem, or a conjectural observation? $\endgroup$– LSpiceCommented Jan 24, 2019 at 19:59
-
2$\begingroup$ well, this would imply that $\Gamma(0,x)$ is an elementary function, which it is not. $\endgroup$ Commented Jan 24, 2019 at 21:28
-
$\begingroup$ You might want to put a carriage return after the equal sign to force all of the RHS to be on one line. And does "It's this expression for any real λ or x" mean "It's this expression if λ is real or x is real" rather than "It's this expression if λ is real and x is real"? $\endgroup$ Commented Jan 24, 2019 at 22:29