Consider the 3rd order ODE
$$\dddot{x}+A\ddot{x}-\dot{x}^{2}+x=0$$ where $\dot{x}\equiv \frac{dx}{dt},\ddot{x}\equiv \frac{d^{2}x}{dt^{2}}, etc$. $A$ is a constant.
If we multiply this equation by $\ddot{x}$ and integrate we can convert it into
$$\frac{1}{2}\ddot{x}^{2}-\frac{1}{3}\dot{x}^{3}+x\dot{x}=C+\int_{0}^{t}(\dot{x}^{2}-A\ddot{x}^{2})ds$$
where $C$ is a constant and $t>0$. If $A\leq 0$ the integral diverges as $t\rightarrow \infty$ and at least one of $\dot{x},\ddot{x},x$ must also diverge.
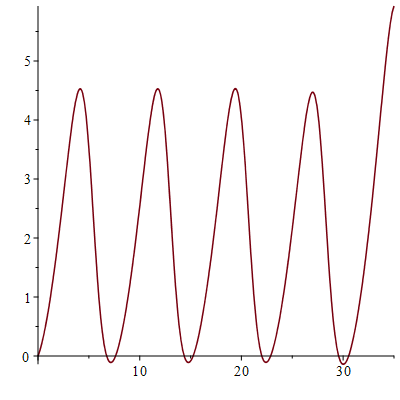
By numerically integrating it seems that for $0<A<1.98$ the solution also diverges very quickly.
I have been wondering whether there is a way of proving this, i.e. that for all $A<A*, A*=1.97...$ (or some other constant $A*>0$) the motion is unbounded (with the exception of $x(t)=0$).