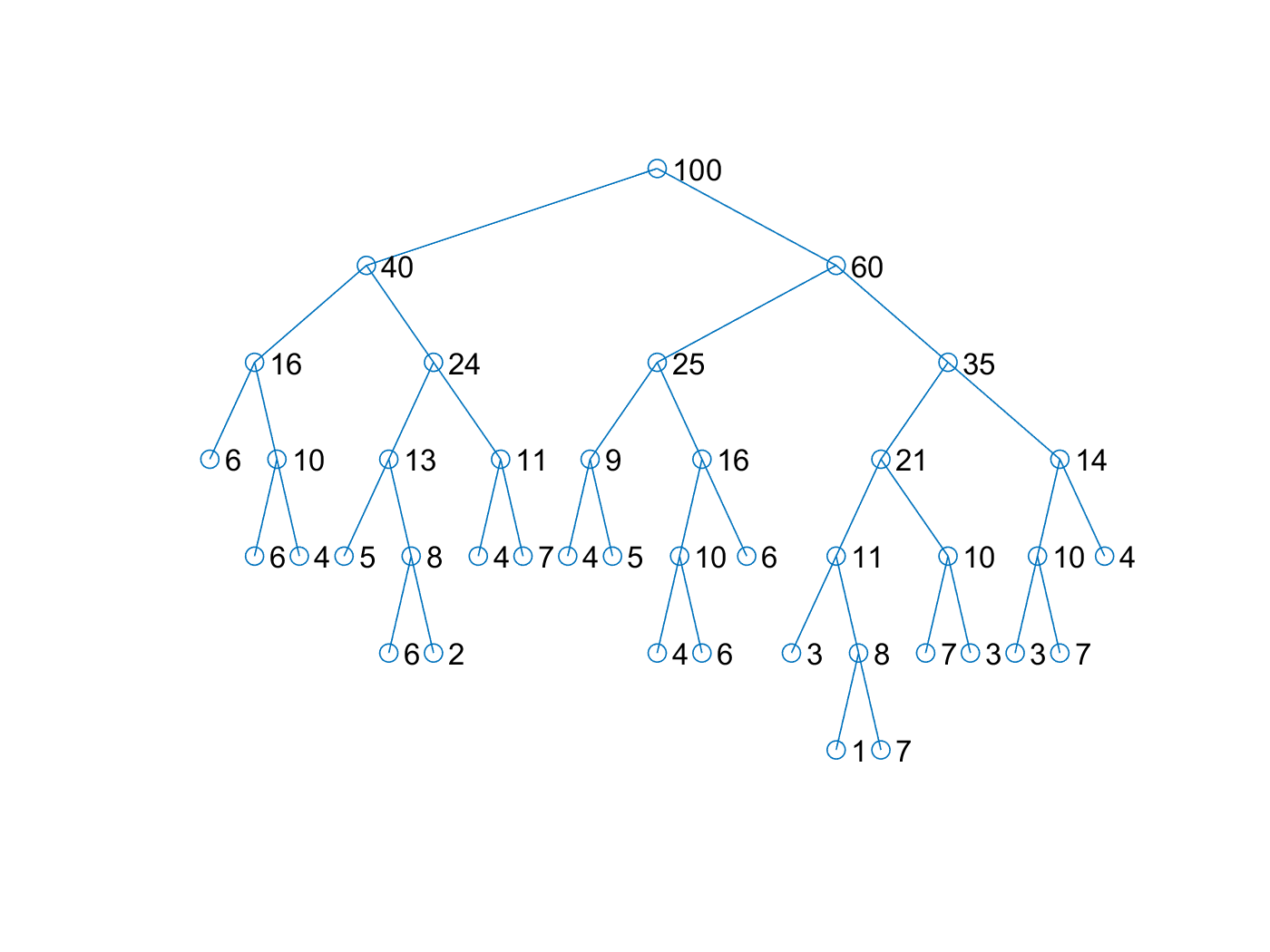
I have come across the following stochastic process which seems very elementary, although I do not know any appropriate terminology for it; I greatly appreciate any suggestions!
Suppose I am given integers $n$ and $k$, with $k\ll n$. I create a binary tree as follows:
- Create a root node, with label $n$.
- While there exists a leaf $u$ whose label $\ell$ is greater than $k$, add two descendants to $u$ whose labels are $X$ and $\ell-X$, where $X$ is a binomial random variable having parameters $1/2$ and $\ell$ respectively.
I am interested in the basic distributional properties of this tree where $n\to\infty$ and $k$ is fixed, such as the depth and the labels of the leaves at termination. Does this have a name? I created one output below for $n=100$ and $k=7$: