Are the following concepts known in graph/group theory, and if Yes, what are they called and where to read about them? Because I do not know better, I gave them placeholder names for now.
1. Transitive edge-colorings of $K_n$
Given a group of permutations $G\subseteq\mathrm{Sym}(n)$ on the $n$ vertices of $K_n$ (the complete graph on $n$ vertices). A transitive edge-coloring on $K_n$ is given by the edge-orbits under $G$, i.e. two edges receive the same color if they belong to the same orbit under $G$. This gives a decomposition of $K_n$ into edge-transitive graphs $\Gamma_1,...,\Gamma_k$ with $G\subseteq\mathrm{Aut}(\Gamma_i)$.
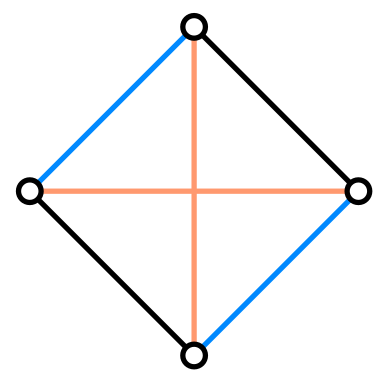
Example. The following transitive coloring was generated by a group $G\subset\mathrm{Sym}(4)$ ismorphic to $\Bbb Z_2\times\Bbb Z_2$. Note that $G$ is a proper subgroup of $\mathrm{Aut}(\Gamma_i)$ for all $\Gamma_i$.
2. Induced transitive edge-colorings
Given an edge-transitive graph $\Gamma$ on $n$ vertices. Then $\mathrm{Aut}(\Gamma)$ induces a transitive edge-coloring on $K_n$ with one of the color classes being $\Gamma$ itself. We say that $\Gamma$ induces the transitive edge-coloring.
Example. The following transitive coloring was generated by $C_6$ (cycle on six vertices). Note that $C_6$ has the strictly smallest automorphism group of all the color classes.
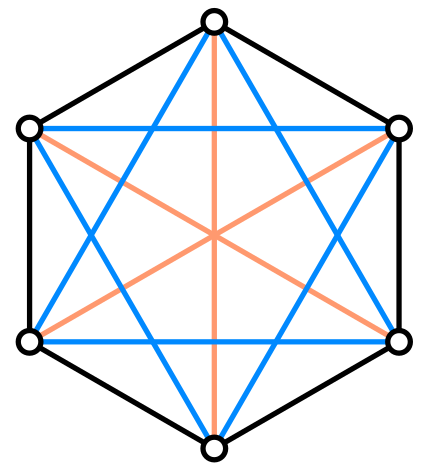
Especially interesting are graphs $\Gamma$ that induce transitive edge-colorings with only connected color classes, because this happens exactly when the $\mathrm{Aut}(\Gamma)$ acts primitively.
3. Transitive decomposition of $K_n$
If all the color classes $\Gamma_1,...,\Gamma_k$ of a transitive edge-coloring induces this coloring, then I call this a transitive decomposition of $K_n$. This means that all the $\Gamma_i$ have the same automorphism group.
Example.
Any edge-transitive graph $\Gamma$ on $n$ vertices with an edge-transitive complement gives a decomposition of $K_n$ with two colors, one for the edges of $\Gamma$, one for the edges of the complement. Among these graphs are the simultaneously arc-transitive and self-complementary graphs, which are fully enumerated by the Payley graphs, Peisert graphs and another exceptional graph on $23^2$ vertices. Another example is the Petersen graph and its complement, the line graph of $K_5$.
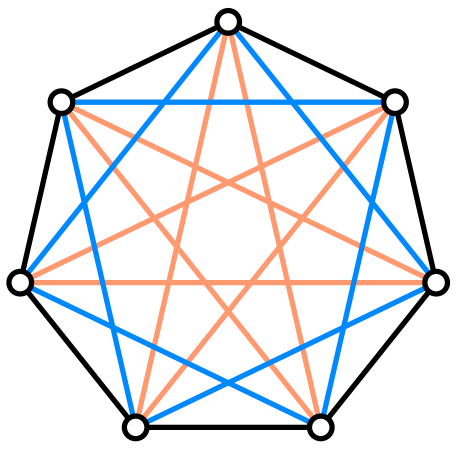
A cycle $C_p$ with $p$ prime gives a transitive decomposition of $K_p$ with $(p-1)/2$ colors. All the color classes are isomorphic. The image below shows the transitive decomposition into three $C_7$'s.