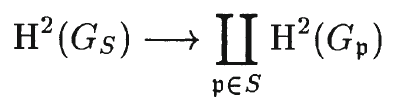I want to know whether for any global number field $k$, the closed subgroup of the idele corresponding to the maximal elementary $p$-extension ($p$ is a prime number) is $k\ J^p$.
The critical point is to show that the subgroup $k\ J^p$ is closed.
If so, as the idele group is locally compact, the quotient group is totally disconnected such that $k\ J^p$ is the intersection of open subgroups of $J$ containing $k\ J^p$. Using the compactness of the quotient group, we see that $k\ J^p$ corresponds to an infinite Abelian extension. By group theory, the Abelian extension must be the maximal elementary $p$-extension.
I checked this to be true when $k$ is $Q$ or an imaginary quadratic extension. In these cases the above is true because the unit group is finite. For general number fields I haven't checked this yet.
So I hope someone can give me a reference or show me a proof or a counterexample.
This fact is of importance, because it is used in the book "Galois Theory of p-Extensions" to bound the kernel of the localization map.