7
$\begingroup$

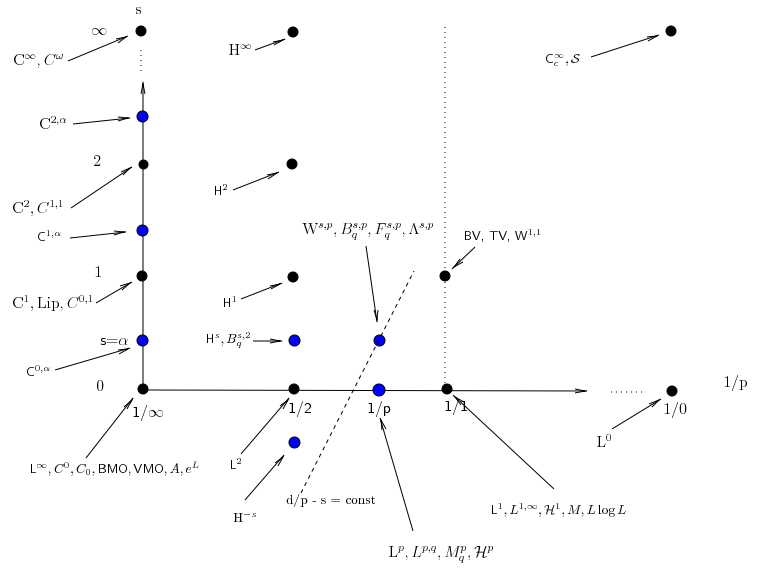
Is there any resource (book, article,...) that presents all the basic function spaces (Metrizable, Normed, Banach, Sobolev, Hilbert, $L^p$, C[a,b], etc.) together with their properties (completeness, separability, reflexivity, compactness, and other special properties maybe...,etc) and diagrams with arrows (which space is also other space> example Normed Space is also a Metrizable space)?

I find it difficult to search anytime properties of these spaces...

Thanks!

Improve this question
$\endgroup$

2 Answers 2

Reset to default
7
$\begingroup$

Terry Tao wrote a blog post describing the following "type diagram of function spaces" a few years ago:

Tao's type diagram for function spaces

The axes are labeled by exponents $s$, corresponding to the regularity and $p$ corresponding to the integrability. See the blog post for more details.

Improve this answer
$\endgroup$
9
$\begingroup$

Some good references are

Dunford, Nelson, and Jacob T. Schwartz. Linear Operators Part I: General Theory. Vol. 7. New York: Interscience publishers, 1958.

(Chapter IV. Special Spaces)

Johnson, William B., and Joram Lindenstrauss. Handbook of the geometry of Banach spaces. Vol. 1. Elsevier, 2001.

(The first contribution "Basic concepts in the geometry of Banach spaces" is quite instructive.)

Triebel, Hans. Theory of function spaces (I-III), Springer

(As the title says, its more focused on function spaces…)

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .