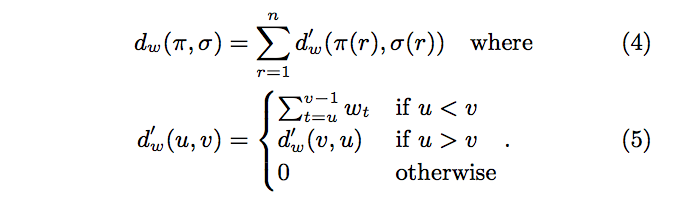What is the maximum weighted earth-mover's distance (as defined in Sun et. al. 2010) between two permutations in $\mathfrak S_n$ where the transposition $(i, i+1)$ has cost given by weight $w_i$. In other words
Is it
$$(n-1)w_1 + (n-2) w_2 + \cdots + w_{n-1}$$
for the permuations $(12\cdots n)$ and $(n(n-1)\cdots 1)$?
Apologies if this is a very obvious question!