Applications of cut locus structure theorems

Question

I am preparing a presentation discussing the structure of the cut locus on Riemannian and Finsler manifolds. Since my audience will consist mostly of applied mathematicians, I was hoping to include some nice applications to other (non-geometric) areas of mathematics which might appeal to the majority of the audience. While I'm vaguely aware that there are some applications to regularity theory, I do not understand these results. I am therefore looking for a nice introduction to this topic in addition to any other applications which might appeal to a group of applied mathematicians.

Answer 1

The cut locus of a point on a compact manifold has zero measure. So this allows to use a single chart centered at the point e.g. to compute integrals (typically radial coordinates in a chart given by the exponential).

You can exemplify this with the sphere (easy, the cut locus is a single point) and tori. The tori are related to Fourier analysis, so this hopefully speaks to applied mathematicians. Then show that there are more than just "square" tori. The cut locus depend on the lattice and since this is a Riemannian invariant, you can show that different tori are not isometric, and explain why we talk about the hexagonal lattice for the lattice built on $1$ and $e^{i {\pi \over 3}}$ (hint: the cut locus is a regular hexagon).

Concerning tori, you can then go slightly further along the relationship between Riemannian geometry and harmonic analysis, the link between isometry and isospectrality, and end up with the famous "Can we hear the shape of a drum" talk and the 16-dimensional Milnor counterexample of two isospectral but not isometric tori, if you have time.

Answer 2

This does not fit your non-geometric requirement, but nevertheless "might appeal to a group of applied mathematicians":

If you cut the cut locus of a point $x$ on the surface of a convex polyhedron $P$ in $\mathbb{R}^3$, then $P$ unfolds to the plane without self-overlap.

---

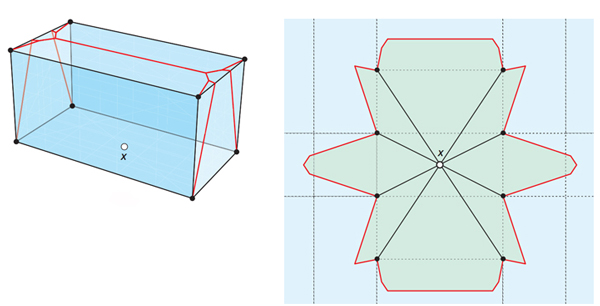
^{Left: The cut locus (red) w.r.t. $x$ on a box. Right: Unfolding resulting from cutting the cut locus.
(Figure from Discrete and Computational Geometry.)}

---

This result generalizes to $\mathbb{R}^d$ for $d > 3$, unfolding without overlap to dimension $d-1$.

Answer 3

Here is a possibility:

Villani, Cédric. "Regularity of optimal transport and cut locus: From nonsmooth analysis to geometry to smooth analysis." Discrete Contin. Dyn. Sys. 30.2 (2011): 559-571.

Abstract. In this survey paper I describe the convoluted links between the regularity theory of optimal transport and the geometry of cut locus.

Theorem 4.1 (convex Earth). [...] Informally speaking: assume that the Earth is very smooth, but not exactly round (there are hills etc.) The theorem says that if you draw a map of the Earth from one given point, it may not look round, but at least it will look convex"