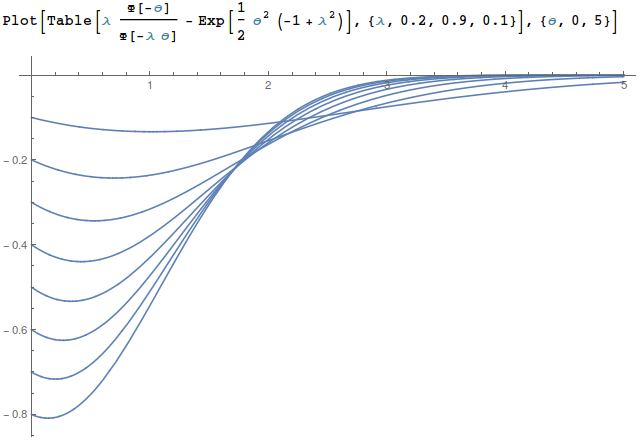
Let $\theta>0$, $0<\lambda<1$, and $\Phi$ be the standard normal cumulative distribution function. Is it true that
$$\frac{\lambda \Phi(-\theta)}{\Phi(-\lambda \theta) }< e^{\frac{\theta^2(-1+\lambda^2)}{2}} $$
Preliminary numerical analysis appears to agree with my assertion. Here are plots of $\lambda=0.2,0.3,\ldots,0.9$.
I claim that even $$\frac{\Phi(-\theta)}{\Phi(-\lambda \theta) }< e^{\frac{\theta^2(-1+\lambda^2)}{2}}.$$ Rewrite this as $h(\theta)<h(\lambda \theta)$, where $h(\theta)=\Phi(-\theta)e^{\theta^2/2}$. So, the claim is that the function $h$ decreases on $(0,+\infty)$. We have $$\frac{d\log h}{d\theta}=\frac{-\Phi'(-\theta)}{\Phi(-\theta)}+\theta=\frac{-e^{-\theta^2/2}}{\int_{-\infty}^{-\theta} e^{-t^2/2}dt}+\theta,$$ the desired inequality $\frac{d\log h}{d\theta}<0$ rewrites as $$\int_{-\infty}^{-\theta} e^{-t^2/2}dt<e^{-\theta^2/2}/\theta$$ This becomes equality when $\theta$ goes to $+\infty$, and the difference $$g(\theta)=\int_{-\infty}^{-\theta} e^{-t^2/2}dt-e^{-\theta^2/2}/\theta$$ increases as is seen from $$\frac{dg}{d\theta}=e^{-\theta^2/2}/\theta^2>0,$$ this finishes the proof.
