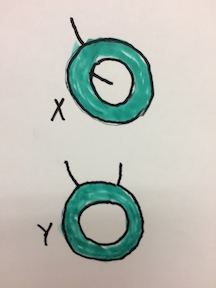
@WlodekKuperberg (perhaps) and I (for sure) were exposed to this kind of examples by Karol Borsuk, or possibly Karol Borsuk simply had an example like the one I will present below:
\begin{equation}
D\ :=\ \{z\in\mathbb C: |z|\le 1\}\ \subseteq\ \mathbb C
\
\end{equation}
\begin{equation}
A\,\ :=\,\ D\times\{0\}\ \cup\ \{1\ \ \ i\ \ -\!1\ \ -\!i\}\times [-1;0]\,\ \subseteq\,\ \mathbb C\times\mathbb R
\end{equation}
\begin{equation}
X\,\ :=\,\ A\,\ \cup\,\ \{-1\ \ 1\}\times [0;1]\,\ \subseteq\,\ \mathbb C\times\mathbb R
\end{equation}
\begin{equation}
Y\,\ :=\,\ A\,\ \cup\,\ \{i\ \ \ 1\}\times [0;1]\,\ \subseteq\,\ \mathbb C\times\mathbb R
\end{equation}
Then $\ X\ $ and $\ Y\ $ are not homeomorphic while $\ X\times I\ $
and $\ Y\times I\ $ are.
REMARK One may check Karol Borsuk's series of publications about the uniqueness of topological decomposition into Cartesian products, and a paper by Hanna Patkowska about the uniqueness of the decomposition of ANRs into 1-dimensional ANRs.
A kind request (I'd greatly appreciate): Wlodek Kuperberg, please add a picture to my analytic description; let the pictures of $\ X\ $ and $\ Y\ $ be embedded into $\ \mathbb C;\ $ I mean homeomorphic copies of $\ X\ $ and $\ Y$.
ACKNOWLEDGEMENT I am grateful to Wlodek Kuperberg for providing such a very nice graphics (so cute and psychologically loaded; it's the first graphics illustration in my MO posts)). *** Włodek, congratulation on your another NICE answer (Gauss said, a few but ripe).