Your question is essentially about extension complexity. In general, the extension complexity of a polytope $P$ is the minimum number of facets over all polytopes $Q$ which project to $P$. You are interested in the extension complexity of polygons. Fiorini, Rothvoß, and Tiwary proved that regular $n$-gons have extension complexity $O(\log n)$. For lower bounds, they give examples of $n$-gons which have extension complexity $\sqrt{2n}$.
It is an open question if there exists for infinitely many $n$, an $n$-gon with extension complexity $\Omega(n)$.
Edit. Shitov has recently shown that every convex $n$-gon has extension complexity at most $147 n^{2/3}$, which resolves the open question in the negative.
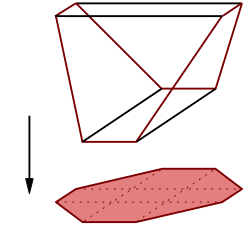
As a bonus, here is a picture of the polytope in Nate's answer (courtesy of Samuel Fiorini).