Solving the geodesic equation for a singularity crossing curve

Question

[Apologies if this question is not considered research level, but it received no substantive comments and no answers at math.SE; I thought it was straightforward, but maybe it isn't.]

Part I - What are the formal conditions for the solubility of the geodesic equation

$$\frac{d^2 x^\mu}{ds^2} =- \Gamma^{\mu}_{\alpha \beta} \frac{dx^\alpha}{ds} \frac{dx^\beta}{ds} ?$$

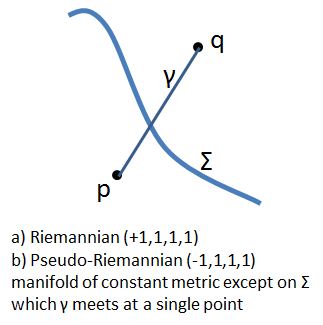
Part II - Consider (see figure) the particular case of a pseudo-Riemannian manifold whose metric is everywhere constant except on the surface $\Sigma$ (and the metric on $\Sigma$ may vary smoothly from place to place) such that every causal curve from $p$ to $q$ intersects the surface $\Sigma$ at a single point (except possibly in the null case), which surface - appearances in the figure not withstanding - might be or have null regions, and across which the metric is discontinuous.

Are there any conditions on the metric on $\Sigma$ (or otherwise) that make the geodesic equation soluble? (Possibly piecewise?).

Notes

The usual approach to solving the geodesic equation will not work, I believe, because of the curvature discontinuity introduced by $\Sigma$ that entails undefined derivatives across $\Sigma$, but maybe if one took as an assumption that $\Sigma$ is nowhere null, one could consider the curves to $\Sigma$ on each side separately and seek identity of their limit points.

If there are regions of $\Sigma$ that are null it seems to me it might be possible for the limit points of the two segments of $\gamma$ to be separated in $\Sigma$.

PS I'm not a mathematician, so in attempting to be suitably precise for those who are, I may in fact have had the opposite effect; I hope others can read between the lines.

Answer 1

This is probably a longer comment, but let me make this into an answer.

Suppose $\gamma$ is a curve in your manifold and we are asking if it satisfies the geodesic equation in some sense. Then either

(a) it hits $\Sigma$ at a discrete set of points $t_k$. Then the natural requirement that $\gamma$ satisfies the geodesic equation on the intervals $(t_k, t_{k+1}), \dots$ plus asking that the left and right sided derivatives at the points $t_k$ coincide already makes it into a geodesic of usual Minkowski space, i.e. a straight line, and the modification of the metric on $\Sigma$ does not play a role.

(b) The set of times $t$ such that $\gamma(t) \in \Sigma$ is not discrete. Then one would need to make sense of the geodesic equation at $\Sigma$, which seems kind of hard, because there is no way to define the Christoffel symbols etc. Also, a weak formulation (using Sobolev spaces etc.) does not really make sense, because $\Sigma$ is a zero set, hence the metric modified on $\Sigma$ as your describe defines the same element in $L^2$ as the original Minkowski metric.

So it seems that either we are in a trivial situation, and your modification just doesn't play a role, or we are in a situation where it is impossible to say what the geodesic equation even means.

Answer 2

Perhaps you mean to say that your metric behaves rather like those arising in Snell's law from geometric optics where to model the refraction of light as it passes from one medium to another you take an index of refraction $n$ constant along slabs with a jumps at the interface? The optical metric is $(1/n) (dx^2 + dy^2 + dz^2)$ where the scalar function $n$ is piecewise constant, suffering a jump at the interface. Do you perhaps mean your pseudo-Riemannian metric to have the form $A_- (dx^2 + dy^2 + dz^2 - c^2 dt ^2 )$ on one side of your hypersurface $\Sigma$, and $A_+ (dx^2 + dy^2 + dz^2 - c^2 dt ^2 )$ on the other side, where $A_+, A_-$ are positive constants? If so, a kind of `Snell law'' will hold. To compute this law for the angle of ``refraction' as your geodesic crosses $\Sigma$ you extremize its arclength relative to the constraint that the curve is piecewise linear with a jump at $\Sigma$. This will yield a calculus problem in 4-space.