relativistic dipole problem

Question

I am trying to understand something in a relativistic dipole problem. The system is described by the following Hamiltonian function $$H=\sqrt{p_r^2+\frac{p_\varphi^2}{r^2}+m^2}+\gamma\frac{\cos\varphi}{r^2},$$ where $m, \gamma$ are positive constants and $r, \varphi$ are the polar coordinates in the plane.

*QUESTIONS.

(a) I am interested in solutions for values near $r\to 0$.

(b) What about asymptotic-in-time behavior of those solutions? It seems that those solutions must form smooth manifolds in the phase-space.

Actually I can not see any thread to pull to unravel this problem. Please do let me know any references, at least to similar problems. Anyway, I appreciate any prompt comments or references.

Answer 1

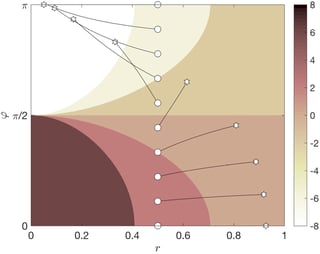
Qualitatively, there are two types of solutions to this problem: trajectories are either attracted (resp. repelled) to the negative (resp. positive) end of the dipole. Which type of solution depends on the initial angle of the particle and its initial radius.

This conclusion is supported by numerical evidence (samples are given below) obtained by solving this Hamiltonian system using a fourth order accurate Runge-Kutta method. Note that it's a bit tricky to apply a symplectic integrator in this context because the Hamiltonian of this system is not separable, but otherwise this problem is trivial to numerically solve because the time intervals of simulation are not that long.

Before delving into these numerical results, let me just say that it does not seem that this conclusion depends strongly on whether the kinetic energy is relativistic (i.e., strictly convex in momentum) or classical (i.e., strongly convex in momentum). It is simply a consequence of the potential energy lacking coercivity, and its not clear how either type of kinetic energy can compensate for this.

For $m=\gamma=1$, here is a sample output showing pieces of trajectories in the $(r,\varphi)$-plane starting (resp. ending) at the point marked with a circle (resp. hexagram). The initial radius for all trajectories is $1/2$ and the initial angles vary as shown. In the background, a few filled contours of the dipole potential energy $U(r,\varphi) = \gamma \cos(\varphi)/r^2$ are shown. Note that for any $r>0$, this potential energy is positive when $\varphi \in [0, \pi/2)$ and negative when $\varphi \in (\pi/2,\pi]$.

Here is a plot of the total energy along the trajectory starting at $\varphi=0$, which is near the positively charged end of the dipole). It shows the RK integrator preserving total energy (black) quite nicely, and the potential energy (blue) being converted to kinetic energy (red) as the distance to the dipole increases.

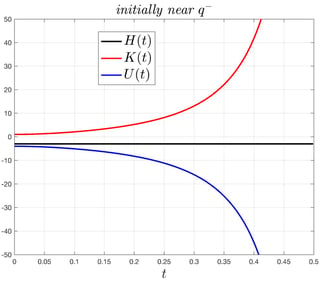
Here is a plot of the total energy along the trajectory starting at $\varphi=\pi$, which is near the negatively charged end of the dipole. In this case, while the total energy (black) is preserved the kinetic and potential energies become unbounded as the distance to the dipole decreases.