This question is migrated from this one on MSE and rephrased more simply.
In this question the following closed form was derived, with $0 < \sigma<1$ and $\sigma,x \in \mathbb{R}$:
$$ \frac{1}{\pi\,\Gamma(2\,\sigma)}\int_{0}^{\infty} \zeta(\sigma + x \, i)\,\zeta(\sigma - x \, i)\,\Gamma(\sigma + x \, i)\,\Gamma(\sigma - x \, i)\,dx =\frac{(2\,\sigma-3)}{(2\,\sigma-1)}\,\zeta(2\,\sigma-1)-\zeta(2\,\sigma)\qquad{(1)}$$
Numerical evidence strongly suggests that (1) continues to hold for $\sigma=\beta\pm t\,i, |t|>0, \,\,\beta,t \in \mathbb{R}$. If true, this implies that either of the two $\zeta$s on the RHS could be made $0$ at a non-trivial zero $\rho$.
We could make $\zeta(2\,\sigma-1)=0$ at e.g $\sigma = \frac34 + \frac{14.134725..i}{2}$, or more generally: for each $\sigma$ where:
$$\displaystyle \frac{1}{\pi\,\Gamma(2\,\sigma)}\int_{0}^{\infty} \zeta(\beta +t\,i+x\,i)\,\zeta(\beta +t\,i-x\,i)\,\Gamma(\beta +t\,i+x\,i)\,\Gamma(\beta +t\,i-x\,i)\,dx +\zeta(2\,\sigma)=0 \qquad{(2)}$$
we will always induce a $\rho$.
To illustrate that it works, here is a graph of the LHS of (2), for $\sigma=\frac34 + \frac{t}{2}\,i$. Integral from $0...48$:
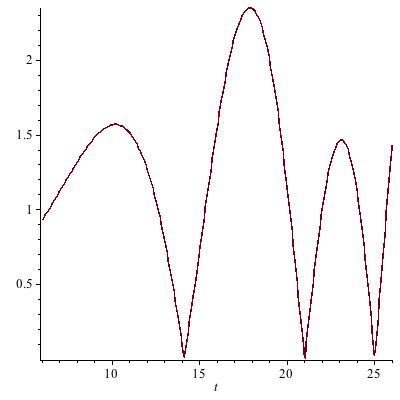
So assuming RH, this would imply that by running the integral through $\sigma = \frac34+\frac{t}{2}\,i$ and adding $\zeta(1.5+t\,i$) (that now can be written as $\displaystyle\prod_{p \in \mathbb{P}} \frac{p^{1.5+t\,i}}{p^{1.5+t\,i}-1}$ or $\displaystyle \sum_{n=1}^{\infty}\frac{1}{n^{1.5+t\,i}}$), we would find all $\rho$s.
Tested it numerically at high accuracy and pretty convinced the results are correct. The integral is quite tough to evaluate though and could become unstable at higher $t$. However, I did get very firm results from using the Adaptive Gaussian Quadrature method for numerical integration.
Note that it is tempting to rewrite the integral into $\displaystyle \int_0^\infty |\zeta(\sigma+x\,i)\,\Gamma(\sigma+x\,i)|^2 dx$. This is fine when $\sigma\in \mathbb{R}$, however when $\sigma = \beta \pm t\,i, |t| >0$, the symmetry of the integrand breaks down -see eq.(2)- and seems to block easy access to integral transforms or other tools from complex analysis.
Question:
Is there a way to proof that equation (1) remains valid for $\sigma=\beta \pm t\,i,|t| >0$ (from which the rest of my question would follow)?
