My question comes from the paper: https://arxiv.org/abs/0911.2750 (p.2~p.3)
For $n\in \mathbf{N}$
- Let $X = (X_1,\ldots,X_n)$ be an $n$-tuple of variables.
- Let $\mathbf{R}[X]$ denote the real polynomial ring in these variables.
- $\mathbf{R}[X]_d$ denotes its finite-dimensional subspace of polynomials of degree at most $d$.
I have two questions on p.3 of that paper as following:
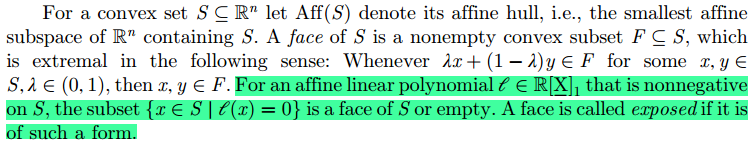
- Does the last sentence make the exposed face any different? (For example, if the face is exposed, then the subset $\{x\in S \mid \textit{l}(x) = 0\}$) is not empty.)
- What about if the face is not exposed?
I do not quite understand the meaning of the green part.
