Before I start, just want to comment that the three claims of the statement above are not quite true. Claim 2 is true only in the direction "if the hexagon $A_1...A_6$ is inscribed then $C_7=C_1$". The converse is not true. The proof goes as follows: first I prove Claim 2 in the proper direction, disproving the converse, and then I prove Claim 1 and Claim 3.
Projectivities and central projectivities:
By projectivity I mean a projective transformation of the
projective plane. They map points to points and lines to lines. By
central projectivity with center $B$ (a point) and axis $b$ (a
line), or in short just central projectiviy, I mean a projective
transformation that fixes $B$ and fixes $b$ point-wise. Its
existence is equivalent to Desargue's theorem. Basically, given
the point $B$, the axis $b$ and two points $A$ and $A'$ not equal
to $B$ and not on $b$, then the central projectivity $\sigma$ with
center $B$ and axis $b$ is uniquely defined by the condition
$\sigma(A)=A'$. Central projectivities are involutions, projective
generalizations of Euclidean reflections in a point). Observe that
because $\sigma^2 = id$ then $\sigma(A') = A$.
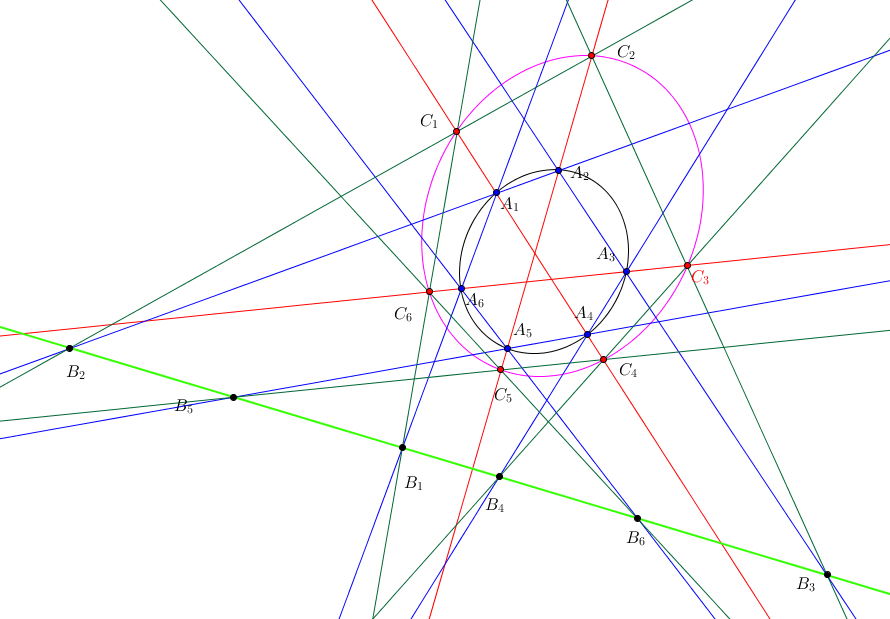
Some constructions and notations: Now, let us have an arbitrary hexagon $A_1A_2A_3A_4A_5A_6$, a line
$L$ disjoint from the vertices of the hexagon, and a construction
of the points $B_1,..., B_6 \in L$ and $C_1,..., C6, C_7$ as
described in the problem. Denote by $E_{36}$ the intersection
point of lines $A_1A_4$ and $A_2A_5$ (from now on, I denote the
point of intersection of any pair of lines by $E_{36} = A_1A_4
\cap A_2A_5$). Analogously, let $E_{14} = A_2A_5\cap A_3A_6$ and
$E_{25} = A_3A_6\cap A_1A_4$. Also, let
$$D_{12} = A_6A_1 \cap A_2A_3, \,\, D_{23} = A_1A_2 \cap A_3A_4,
\,\, D_{34} = A_2A_3 \cap A_4A_5,$$ $$D_{45} = A_3A_4 \cap A_5A_6,
\,\, D_{56} = A_4A_5 \cap A_6A_1, \,\, D_{61} = A_1A_2 \cap
A_5A_6.$$ Define the lines $$b_2 = D_{12}E_{36}, \,\, b_3 =
D_{23}E_{14}, \,\, b_4 = D_{34}E_{25},$$ $$b_5 = D_{45}E_{36},
\,\, b_6 = D_{56}E_{14}, \,\, b_1 = D_{61}E_{25}.
$$
Denote by $\sigma_i$ the central projectivity with center $B_i$
and axis $b_i$ such that $\sigma_i(A_{i}) = A_{i-1}$ for
$i=1,..,6$ where in the case of $\sigma_1$ we have
$\sigma_1(A_{1}) = A_{6}$. By construction $\sigma_i(L)=L$ (not
point-wise) for all $i=1,..,6$. Moreover, since $\sigma_2(A_2) =
A_1$ and $\sigma_2(E_{36})=E_36$, the line $A_1A_4$ gets mapped to
the line $A_2A_5$ and $A_2A_5$ gets mapped to $A_1A_4$ by
$\sigma_2$. Absolutely analogously, the same is true for the rest
of the central projectivites $\sigma_3,..., \sigma_6, \sigma_1$
and the appropriate pairs of lines formed from the set of three
lines $A_2A_5, A_3A_6$ and $A_1A_4$.
Define the projectivity $f = \sigma_1 \circ \sigma_6 \circ
\sigma_5 \circ \sigma_4 \circ \sigma_3 \circ \sigma_2$. Let
$M_{14} = L \cap A_{1}A_{4}, M_{25} = L \cap A_{2}A_{5}$ and
$M_{36} = L \cap A_{3}A_{6}$. Then $$\sigma_2(M_{14}) = M_{25},
\sigma_3(M_{25}) = M_{36}, \sigma_4(M_{36}) = M_{14},$$ $$
\sigma_5(M_{14}) = M_{25}, \sigma_6(M_{25}) = M_{36},
\sigma_2(M_{36}) = M_{14}.$$ Therefore $f(M_{14}) = M_{14}.$ In
addition to that, if we look at the images of the points $B_1$ and
$B_2$ under the consecutive application of the projectivites
$\sigma_2,..., \sigma_6, \sigma_1$ in this order, we arrive at the
chain of images
$$B_1 \mapsto B_3 \mapsto B_3 \mapsto B_5 \mapsto B_5 \mapsto B_1 \mapsto B_1,$$
$$B_2 \mapsto B_2 \mapsto B_4 \mapsto B_4 \mapsto B_6 \mapsto B_6 \mapsto B_2.$$
Therefore, $f(B_1) = B_1$ and $f(B_2) = B_2$. But a projective
transformation, in this case $f$, which fixes three points on a
line, fixes the whole line point-wise. Hence, $f$ fixes $L$
point-wise. Moreover, by construction, $f(A_1) = A_1$. Therefore,
$f$ is a central projectivity with a center $A_1$ and axis $L$.
Also by construction, $f(C_1) = C_7$.
Lemma 1: If the six lines (the axes of the central
projectivites $\sigma_1,...,\sigma_6$) $b_1,...,b_6$ intersect at
a common point $O$, then $f$ is the identity and so $C_7 = f(C_1)
= C_1.$
Proof of Lemma 1: Indeed, by construction $f(O)=O$ and since $O$
is different from $A_1$ and does not lie on $L$, the
transformation $f$ is a central projectivity which fixes one extra
point, which is possible only when $f$ is the identity.
Corollary 1: If the hexagon $A_1...A_6$ is superscribed
around a conic, then $f$ is an identity and $C_7 \equiv C_1$ which
means the polygonal chain $C_1...C_6$ closes up to a hexagon.
Proof of corollary 1: By Brianchon's theorem the diagonal lines
$A_1A_4, A_2A_5$ and $A_3A_6$ intersect in a common point, call it
$O$. Then $O\equiv E_{36} \equiv E_{14} \equiv E_{25}$ and
therefore by construction the axes $b_1,..., b_6$ intersect in
$O$.
As you can see by the latter corollary, $C_7 \equiv C_1$ occurs in
the case of any superscribed around a conic hexagon and since
there are definitely superscribed hexagons that are not inscribed
in conics, the statement that "if $C_7 \equiv C_1$ then
$A_1...A_6$ is inscribed in a conic" cannot be true.
Proof of Claim 2 from the main statement.
Let $A_1...A_6$ be a
hexagon inscribed in a conic.
Lemma 2: The three lines $D_{12}D_{45}, D_{23}D_{56}$ and
$D_{34}D_{61}$ intersect in a common point called $O$.
Proof of Lemma 2: Look at triangles $D_{12}D_{34}D_{56}$ and
$D_{23}D_{45}D_{61}$. By Pascal's theorem for $A_1...A_6$ the
intersection points $D_{12}D_{56} \cap D_{23}D_{45}, \,\,
D_{12}D_{34} \cap D_{45}D_{61}$ and $D_{34}D_{56} \cap
D_{23}D_{61}$ lie on a common line. By Desargue's theorem, applied
to the triangles $D_{12}D_{34}D_{56}$ and $D_{23}D_{45}D_{61},$
the three lines $D_{12}D_{45}, \, D_{23}D_{56}$ and $D_{34}D_{61}$
intersect at a common point, denoted by $O$.
Lemma 3: I claim that $E_{25} \in D_{61}D_{34}, \, E_{36} \in
D_{12}D_{45}$ and $E_{14} \in D_{23}D_{56}$. Consequently
$D_{61}D_{34} = b_1=b_4$ and $D_{12}D_{45} = b_2=b_5$ and
$D_{23}D_{56} = b_3=b_6$.
Proof of Lemma 3: The (self intersecting) hexagon
$A_1A_2A_3A_6A_5A_4$ (observe the order of the vertices!) is
inscribed in a conic, because $A_1A_2A_3A_4A_5A_6$ is inscribed by
assumption. By Pascal's theorem for $A_1A_2A_3A_6A_5A_4$, the
points $E_{25}, D_{61}$ and $D_{34}$ are collinear, that is
$E_{25} \in D_{61}D_{34}$ and thus $D_{61}D_{34} = b_1=b_4$. The
rest of the claims follow analogously.
By combining Lemma 2 with Lemma 3, one concludes that the axes of
the central projectivities $\sigma_1,...,\sigma_6$ pass through a
common point $O$ and therefore by Lemma 1 it follows that the
projectivity $f$ is the identity and $C_7 = f(C_1) = C_1$.
Proof of Claim 1 from the main statement.
Let $A_1A_2A_3A_4A_5A_6$ be inscribed in a conic. By Claim 2,
$C_7 \equiv C_1$ and so $B_1$ lies on the line $C_1C_6$. By
Desargue's theorem applied to the triangles $A_1B_1C_1$ and
$A_2B_3C_2$ it follows that the three intersection points
$E_{36}=A_1C_1 \cap A_2C_2, \,\,
D_{12}=A_1B_1 \cap A_2B_3$ and $F_{12}=B_1C_1 \cap B_3C_2$ are
collinear and since $D_{12}E_{36}=b_2,$ clearly $F_{12} \in b_2.$
Next, apply Desargue's theorem to the triangles $A_4B_4C_4$ and
$A_5B_6C_5$ in order to conclude that $E_{36}=A_4C_4 \cap A_5C_5,
\,\,
D_{45}=A_4B_4 \cap A_5B_6$
and $F_{45}=B_4C_4 \cap B_6C_5$ are collinear. Since
$D_{45}E_{36}=b_4=b_2$ by Lemma 3, as before $F_{45} \in b_2.$
Therefore the three points $F_{12}, \, F_{45}$ and $E_{36}$ lie
on the line $b_2$.
Consequently, if one looks at the hexagon $C_2C_3C_4C_1C_6C_5$
(observe the order of the points!)
one can conclude that since $F_{12}, \, F_{45}, \, E_{36} \in b_2$
by Pascal's theorem $C_2C_3C_4C_1C_6C_5$ is inscribed in a
conic. Hence, the hexagon $C_1C_2C_3C_4C_5C_6$ is inscribed
in the same conic.
Conversely, let $A_1A_2A_3A_4A_5A_6$ be an arbitrary hexagon
and $C_1C_2C_3C_4C_5C_6$ be constructed by following the
procedure given in the main statement but stopping after
constructing the point $C_6$ and then simply connecting $C_6$ to
$C_1$, assuming that $C_7$ and $C_1$ may not coincide.
Furthermore, assume that $C_1C_2C_3C_4C_5C_6$ is inscribed in a
conic. Denote by $B'_1$ the intersection point of $C_6C_1$ with
$L$. By interchanging the two hexagons in the construction given in
the main statement, we can use $C_1C_2C_3C_4C_5C_6$ as a
starting hexagon, the line $L$ and then starting from point
$A_1$ follow the same method of constructing $A_2, A_3, A_4, A_5, A_6$
and then using $B'_1$ construct $A_7$. But by Claim 1, $A_7\equiv
A_1$ because $C_1C_2C_3C_4C_5C_6$ is inscribed in a conic. By
the already proven direction of Claim 2, the hexagon
$A_1A_2A_3A_4A_5A_6$ is also inscribed in a conic. Finally, that
guarantees that $B_1, C_6$ and $C_1$ are collinear and hence
$B'_1 \equiv B_1$.
Proof of Claim 3 from the main statement.
Assume
$A_1A_2A_3A_4A_5A_6$is inscribed in a conic. By Claim 2, $B_1 \in
C_6C_1$ and by Claim 1 $C_1C_2C_3C_4C_5C_6$ is inscribed in a
conic.
Some notations: Let $P_{14} = A_2A_3 \cap A_5A_6, \,
P_{25} = A_1A_2 \cap A_4A_5$ and $P_{36} = A_3A_4 \cap A_6A_1.$
By Pascal's theorem the three intersection points
$P_{14}, P_{25}$ and $P_{36}$ lie on a common line $p$.
Furthermore, let $Q_{14} = C_2C_3 \cap C_5C_6, \,
Q_{25} = C_1C_2 \cap C_4C_5$ and $Q_{36} = C_3C_4 \cap C_6C_1.$
By Pascal's theorem the three intersection points
$Q_{14}, Q_{25}$ and $Q_{36}$ lie on a common line $q$.
Claim 3 from the main statement: The three lines $p, q$ and
$L$ are concurrent.
Let $H_{14} = A_1A_4 \cap D_{23}D_{56} = A_1A_4 \cap b_3,
\, H_{25} = A_2A_5 \cap D_{34}D_{61} = A_2A_5 \cap b_1$ and
$H_{36} = A_3A_6 \cap D_{45}D_{12} = A_3A_6 \cap b_2$.
Lemma 4: If $A_1A_2A_3A_4A_5A_6$ is inscribed in a conic then
- the three points $H_{14}, H_{25}$ and $P_{36} = A_1A_2 \cap A_4A_5$
are collinear;
- the three points $H_{36}, H_{14}$ and $P_{25} = A_3A_4 \cap A_6A_1$ are collinear;
- the three points $H_{25}, H_{36}$ and $P_{14} = A_2A_3 \cap A_5A_6$
are collinear;
Proof of Lemma 4: Look at triangles $A_4D_{23}H_{14}$ and
$A_5D_{61}H_{25}$. Then $A_4H_{14} \cap A_5H_{25} = A_4A_1 \cap
A_5A_2 = E_{36}$, as well as $D_{23}H_{14} \cap D_{61}H_{25}
= b_2 \cap b_1 = O$, and finally $D_{23}A_4 \cap D_{61}A_5 =
A_3A_4 \cap A_5A_6 = D_{45}$. By Lemma 2, $O \in b_3 =
D_{45}E_{36}$, therefore by Desargue's theorem the three
intersection points $D_{61}D_{23} \cap A_4A_5 = A_1A_2 \cap
A_4A_5 = P_{36}$ are collinear. The rest of the statements are
proven analogously.
Next, apply Lemma 4 to the inscribed in a conic hexagon
$C_1C_2C_3C_4C_5C_6$ in place of $A_1...A_6$ and observe that
by construction
$A_1A_4=C_1C_4, \, A_2A_5 = C_2C_5, \, A_3A_6 = C_3C_6$. Then
one concludes that $Q_{36} \in H_{14}H_{25}, \,
Q_{25} \in H_{36}H_{14},$ and $Q_{14} \in H_{25}H_{36}$ because
the points $F_{12}, D_{12}, E_{36}, O, D_{45}, F_{45} \in b_2$,
as well as $F_{23}, D_{23}, E_{14},$ $O, D_{56}, F_{56} \in b_3$
and $F_{34}, D_{34}, E_{25}, O, D_{61}, F_{61} \in b_1$
according to Lemma 2, Lemma 3 and the facts that the points
$F_{ij}$ lie on the appropriate axes $b_j$ established in the
proof of Claim 1 from the statement. Therefore
$H_{25} \in P_{36}Q_{36}$ and
$H_{25} \in P_{14}Q_{14}$. Since $H_{25} = A_2A_5 \cap b_1$ and
$A_2A_5 = C2C_5$, one concludes that $H_{25} \in C_2C_5$ and
thus $H_{25} = P_{36}Q_{36} \cap P_{14}Q_{14} \in C_2C_5$.
Look at triangles $B_2P_{36}Q_{36}$ and $B_3P_{14}Q_{14}.$ Then
$B_2Q_{36} \cap B_3Q_{14} = C_2 \in C_2C_5$, furthermore
$B_2P_{36} \cap B_3P_{14} = A_2 \in C_2C_5 = A_2A_5$ as well as
$P_{36}Q_{36} \cap P_{14}Q_{14} = H_{25} \in C_2C_5$. By
(the converse) Desargue's theorem, the three lines
$B_2B_3 = L, \, P_{36}P_{14} = p$ and $Q_{36}Q_{14} = q$ are
concurrent.