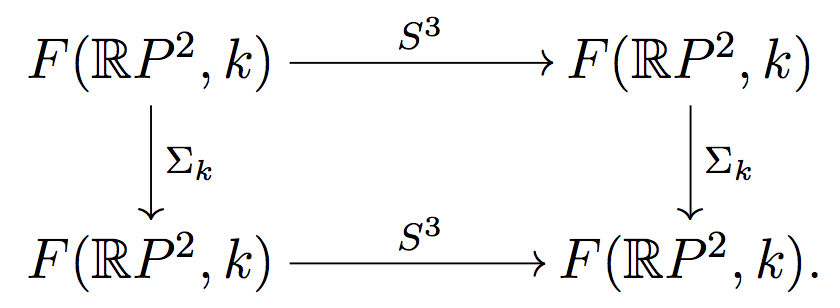
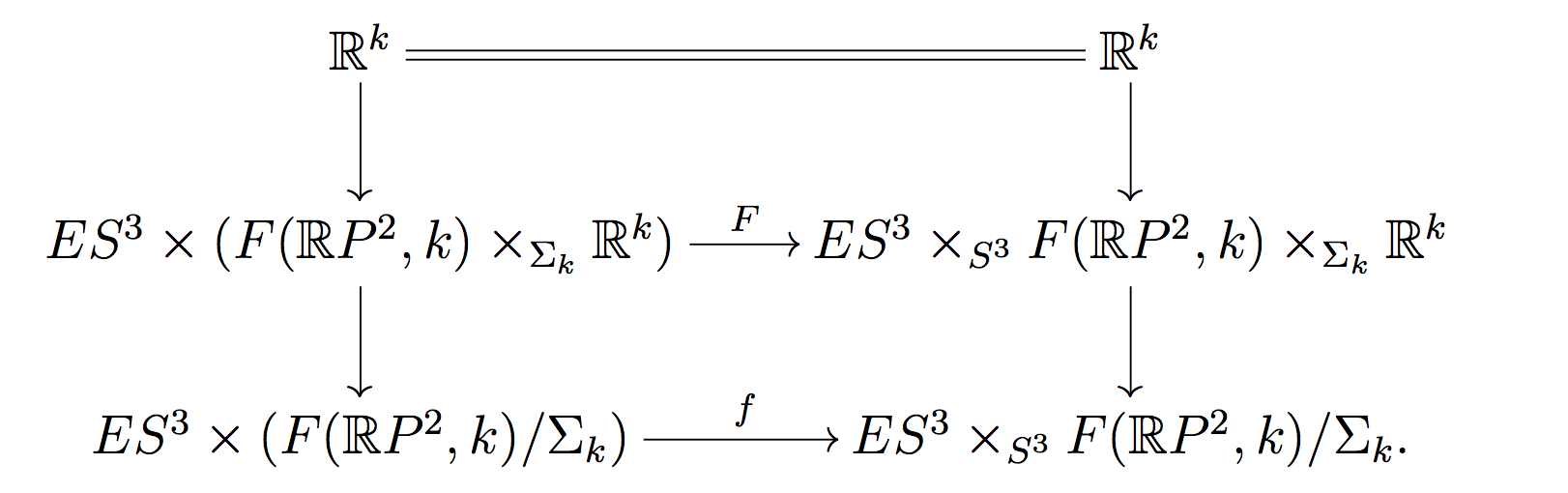Let $\mathbb{R}P^2$ be the lines in $\mathbb{R}^3$ passing through the origin. Let $SO(3)$ act on $\mathbb{R}^3$ canonically. Then $SO(3)$ has an induced action on $\mathbb{R}P^2$. Let $F(\mathbb{R}P^2,k)$ be the configuration space consisting of all ordered $k$-tuples of distinct points in $\mathbb{R}P^2$. Then $SO(3)$ acts on $F(\mathbb{R}P^2,k)$ diagonally. Let $S^3$ be the $2$-sheeted cover of $SO(3)$. Then $S^3$ also acts on $F(\mathbb{R}P^2,k)$. Let the $k$-th symmetric group $\Sigma_k$ act on $F(\mathbb{R}P^2,k)$ by permuting coordinates. Then we have a commutative diagram
Question. Whether can we obtain a pull-back diagram of vector bundles or not?
Here $F$ and $f$ are obtained by modulo the actions of $S^3$.